Last year, in a series of posts, I gave you a tour of quantum field theory, telling you some of what we understand and some of what we don’t. I still haven’t told you the role that string theory plays in quantum field theory today, but I am going to give you a brief tour of string theory before I do.
What IS String Theory? Well, what’s Particle Theory?
What is particle theory? It’s nothing other than a theory that describes how particles behave. And in physics language, a theory is a set of equations, along with a set of rules for how the things in those equations are related to physical objects. So a particle theory is a set of equations which can be used to make predictions for how particles will behave when they interact with one another.
Now there’s always space for confusion here, so let’s be precise about terminology.
- “Particle theory” is the general category of the equations that can describe particles, of any type and in any combination.
- “A particle theory” is a specific example of such equations, describing a specific set of particles of specific types and interacting with each other in specific ways.
For example, there is a particle theory for electrons in atoms. But we’d need a different one for atoms with both electrons and muons, or for a bottom quark moving around a bottom anti-quark, even though the equations would be of a quite similar type.
Most particle theories that one can write down aren’t relevant (or at least don’t appear to be relevant) to the real world; they don’t describe the types of particles (electrons, quarks, etc.) that we find (so far) in our own universe. Only certain particle theories are needed to describe aspects of our world. The others describe imaginary particles in imaginary universes, which can be fun, or even informative, to think about.
Modern particle theory was invented in the early part of the 20th century in response to — guess what? — the discovery of particles in experiments. First the electron was discovered, in 1897; then atomic nuclei, then the proton, then the photon, then the neutrino and the neutron, and so on… Originally, the mathematics used in particle theory was called “quantum mechanics”, a set of equations that is still widely useful today. But it wasn’t complete enough to describe everything physicists knew about, even at the time. Specifically, it couldn’t describe particles that move at or near the speed of light… and so it wasn’t consistent with Einstein’s theory (i.e. his equations) of relativity.
What is Quantum Field Theory?
To fix this problem, physicists first tried to make a new version of particle theory that was consistent with relativity, but it didn’t entirely work. However, it served as an essential building block in their gradual invention of what is called quantum field theory, described in much more detail in previous posts, starting here. (Again: the distinction between “quantum field theory” and “a quantum field theory” is that of the general versus the specific case; see this post for a more detailed discussion of the terminology.)
In quantum field theory, fields are the basic ingredients, not particles. Each field takes a value everywhere in space and time, in much the same way that the temperature of the air is something you can specify at all times and at all places in the atmosphere. And in quantum field theory, particles are ripples in these quantum fields.
More precisely, a particle is a ripple of smallest possible intensity (or “amplitude”, if you know what that means.) For example, a photon is the dimmest possible flash of light, and we refer to it as a “particle” or “quantum” of light.
We call such a “smallest ripple” a “particle” because in some ways it behaves like a particle; it travels as a unit, and can’t be divided into pieces. But really it is wave-like in many ways, and the word “quantum” is in some ways better, because it emphasizes that photons and electrons aren’t like particles of dust.
To sum up:
- particles were discovered in experiments;
- physicists invented the equations of particle theory to describe their behavior;
- but to make those equations consistent with Einstein’s special relativity (needed to describe objects moving near or at the speed of light) they invented the equations of quantum field theory, in which particles are ripples in fields.
- in this context the fields are more fundamental than the particles; and indeed it was eventually realized that one could (in principle) have fields without particles, while the reverse is not true in a world with Einstein’s relativity.
- thus, quantum field theory is a more general and complete theory than particle theory; it has other features not seen in particle theory.
Now what about String Theory?
In some sense, strings also emerged from experiments — experiments on hadrons, back before we knew hadrons were made from quarks and gluons. The details are a story I’ll tell soon and in another context. For now, suffice it to say that in the process of trying to explain some puzzling experiments, physicists were led to invent some new equations, which, after some study, were recognized to be equations describing the quantum mechanical behavior of strings, just as the equations of particle theory describe the quantum mechanical behavior of particles. (One advantage of the string equations, however, is that they were, from the start, consistent with Einstein’s relativity.) Naturally, at that point, this class of equations was named “string theory”.
An aside: theories of non-relativistic strings have appeared in the literature, for instance in Gimon et al. from 2002. There must be earlier versions, but I haven’t found them. [Experts will think of light-cone gauge.]
A few more years of study of these equations led to a number of realizations about the simplest forms of string theory. Note my use of the vague term “simplest forms”. I’ve used this to alert you that what I’m about to say about string theory is not always true. It is true of what people knew about string theory back in 1985 or so. But keep in mind this is not the final word on what “string theory” means; it was, rather, just the first attempt. I’ll make this concept much less vague in my next post.
Anyway, here are some things that people learned about this theory in the 1970s and 1980s, presented in a somewhat ahistorical order.
Just as particles are ripples in fields, strings can be seen as a sort of ripple in string fields. (See Figure 1.) But unlike particle theory, which is actually incomplete unless one takes a field point of view, the mathematics of the string equations make it easier to understand strings on their own, without the use of string fields. And string field theory is very complicated, and still very poorly understood even today. This is part of why, for strings, people usually talk about “string theory” and not “string field theory”, while for particles, people usually talk about “field theory”, not “particle theory”.

In its simplest context, a theory of strings is equivalent to a theory of a huge number of fields and their particles. Roughly, even though there’s only one type of string, a string can move or vibrate in different ways. A string vibrating in one way will appear in an experiment as though it is one type of particle; a string vibrating in a different way will appear to be a different particle. In short, a single type of string, though so small it seems like a particle in current experiments, has many types of vibrations, and these would appear in current experiments to be many types of particles. See Figure 2. The masses and other properties of these particles, and the forces by which they interact with each other, are arranged in special patterns, a point I’ll return to in a moment.
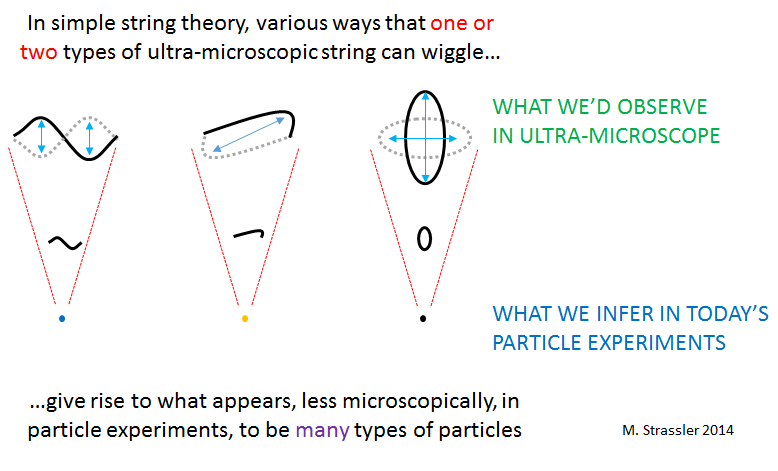
The simplest string theories have only boson particles (particles which, like Higgs particles, photons and gravitons, have spin = 0,1,2…). The next simplest are superstring theories, which also have fermion particles (which have spin 1/2, 3/2, …; electrons and quarks have spin 1/2.) To describe our world, then, superstrings are necessary.
Moreover, for a theory of strings to be consistent and stable (remember a theory is a set of equations — it has to make mathematical sense, or you can’t use it to make predictions of any sort) the strings have to be superstrings, at least approximately.
In addition, for a theory of superstrings to be consistent, the strings have to [with a few subtle caveats] move in a space with 9 spatial dimensions. That is, adding on time as one more dimension, they can exist only in universes with a total of 10 space-time dimensions. That sounds bad… but it isn’t. It might be that only a few of those dimensions are like the ones we know; the others might be so short that we might not notice them, much as a sheet of paper appears two-dimensional if you don’t notice its thickness (Figure 3). So our world could be described by string theory as long as there are six unseen “extra dimensions”, which are too short for us to detect with current experiments.

In a (simple) superstring theory which describes a world similar to the one we live in, with three very large spatial dimensions, the pattern of masses for the particles in that universe would be something vaguely like that shown in Figure 4. There would be huge numbers of types of heavy particles, with masses so large that we aren’t even close to producing them in our experiments, and in the near term we have no hope of checking whether they can exist. Only a small number of types of particles, massless or with rather small masses, will be easily observable. These include (Figure 4)
- particles and fields similar in type, though with details that may vary widely, to those that we find in the Standard Model of particle physics (the quantum field theory that describes the known particles and non-gravitational forces.) The equations that describe them would be those of quantum field theory.
- a graviton: a spin-two particle that is a ripple in a gravitational field. The equations for this field are those of Einstein’s general relativity, in which gravity is an effect of the curvature of space and time. But these equations are generalized into a quantum mechanical form, which we call “quantum gravity”.
Let me say that again, because it’s a prediction — not a hugely impressive one, because it is vague and after-the-fact, but nevertheless, something deserving of the name.
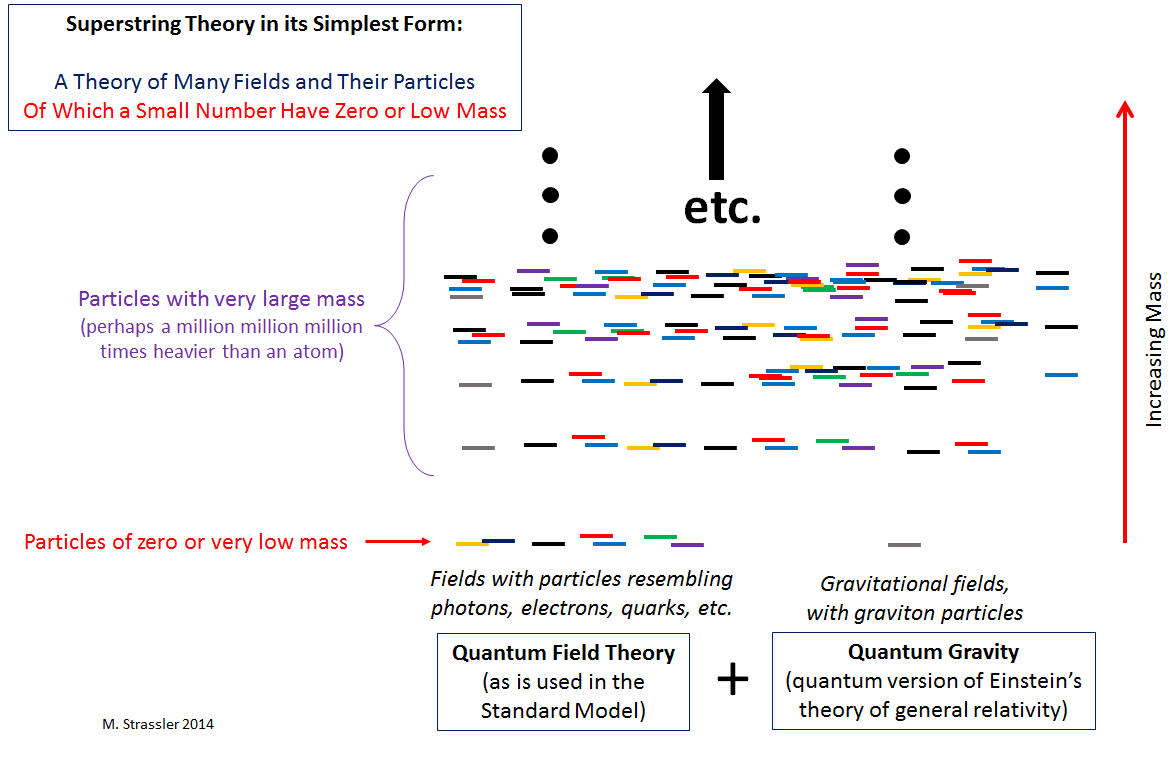
String Theory’s First Prediction (Vague, and with Loopholes)
In its simplest forms, string theory, at least naively, predicts that in a universe whose basic objects are superstrings, one will probably observe a quantum version of something similar to (and possibly identical to) Einstein’s vision of gravity, and probably other very lightweight and massless particles not entirely dissimilar from the ones we observe, along with additional forces like the ones we observe… all of which can be described using quantum field theory.
Now this is a remarkable prediction of the theory [again, I remind you, of the theory in its simplest form, i.e. as it was understood in 1985], because this prediction agrees with data.
But how excited should we be about this success? The prediction is quite vague; it’s analogous to the fact that (as I explained here) the simplest forms of quantum field theory (before you choose a particular example) make only a few, very vague, predictions: that there will be particles in the world; these will be fermions or bosons; and any two particles of the same type will be literally identical. This prediction of string theory is so vague that its success is hardly convincing; one could imagine there are lots of other theories out there that predict the same thing. And just as complicated quantum field theories don’t always have particles, this simple prediction isn’t necessarily true when string theory gets complicated.
Furthermore, it’s a prediction of something we already knew about the world, which physicists sometimes call a postdiction. It is a lot easier to make a prediction about nature when you already know what the answer has to be! An example of a postdiction is Einstein’s calculation that his theory of general relativity, which he was developing at the time, predicted the small observed shift in Mercury’s orbit. The shift was already known from data, so he had a target to aim at… and that’s part of why people were mildly impressed (his theory could have failed at this step by giving the wrong answer) but hardly convinced. It was (and is) much more impressive that the theory correctly predicted things that had never previously been observed — the deflection of light by the sun, the gravitational slowing-down of clocks, the gravitational redshift of light, energy loss by radiation of gravitational waves, etc.
But historically and sociologically, this first prediction of string theory was a very important one. People had been trying for many years to find a quantum theory of gravity that would also be consistent with the quantum field theories used to describe other particles… or perhaps something more general that would contain both Einstein’s theory of gravity and the quantum field theory that describes the other forces and particles. It was a sort of “holy grail”, one that Einstein himself spent his last 30 years seeking fruitlessly. So you have to understand that it was quite remarkable that a theory of strings, once the equations were worked out carefully, simply dumped the holy grail on the table without anyone asking or looking for it to do so.
Of course, we didn’t and still don’t know it is the only holy grail; maybe there are others. But at worst, a simple form of superstring theory is a very interesting example of how a quantum theory containing both gravity and field theory might work!
Is Our World a World of Strings?
In short, back when physicists were still new to string theory, they found that simple forms of string theory could potentially describe universes much like the one we live in. And this fact generated a lot of optimism that maybe there is a string theory that describes our own universe. But if so, which one?
By 1983, it turned out that most string theories anyone had written down were mathematically inconsistent (or had to be very complicated to make them consistent), but not all of them. A short list of 5 simple superstring theories survived all mathematical requirements, and only one seemed relevant for our own universe. I’ll say a bit more about them in my next post. And since there was only one of these that seemed directly relevant, a huge wave of over-optimism swept across the string theory experts [I was an undergraduate, watching with some skepticism, at the time] that they were on the verge of figuring out the complete theory of elementary particles, forces and space-time.
I should note that at this same time the string theory folks took the unfortunate step of annoying all the other physicists, along with chemists, biologists, social scientists of all sorts, artists, writers, lawyers, and chefs by calling this potentially complete theory of particles, forces and spacetime a “Theory of Everything”. We won’t use that term here.
But This Was Just The Beginning of a Saga
Let me pause at this moment to make some important cautionary remarks.
Up to this point, the methods that people had used to study string theories were of a similar type used (as I described here) in the simplest quantum field theories. This is a method known as “perturbation theory”, which is a technique of successive approximation: the full calculation is written as a simple estimate, plus a small but more complicated correction to the estimate, plus a smaller but even more complicated correction to the correction… etc.
The problem is that — just as in quantum field theory — successive approximation only works when all relevant forces are relatively weak (in a technical sense — see this post). But there are some questions in string theory — most notably, how does the world end up with 3 large spatial dimensions rather than 9? and why don’t the strengths of all forces end up being zero? — for which the method of successive approximation is not good enough. When this was realized in the mid-1980s, progress in string theory slowed down markedly, and the goal of a complete theory of particles, forces and space-time receded for the moment.
Nevertheless, some experts continued to explore string theory, and made slow but steady progress. And then, spurred in part by advances in quantum field theory in 1993-1994, a major set of realizations occurred in string theory studies during the period 1994-1998. By the time that mini-revolution was over, what string theorists knew about this theory had dramatically expanded and changed. And that will be the subject of my next post in this series.



109 Responses
Thanks for the link to your discussion of extra dimensional space. I was unaware of the prior tutorial. Two quick follow-ups if I may.
First, why are the Euclidean spatial dimensions seemingly unbounded (astronomical scale awareness), but the conceptual subatomic scale spatial dimensions are thought to be bounded and very uniquely shaped? Why would nature be so fickle.
Second, the “time” dimension can be thought of as a surrogate parameter for the cosmological motion that has been ongoing since the Big Bang, and consequently has mathematical analog to movement within the 3 spatial dimensions. As such, could the known spatial dimensions fluctuate minutely, thereby opening voids that may account for extra dimensional phenomena?
Just a quick question relating to the meaning “dimensions” in this context.
It seems to me that there are at least two possible conceptions of the idea that there may more than 3 spacial dimensions.
The first is that we (as human thinkers) lack the intuitive ability to visualize space in more than 3 spacial dimensions, and therefore develop confusing analogies or rationalizations which imply validity and justification.
The second is that all spacial dimensions in excess of the 3 Euclidean dimensions simply overlap with the original 3, but we treat them differently because the math is easier within the context of the newly envisioned space. Another way to say this is that atomic space overlaps astronomical space, but you cannot efficiently use astronomical math to solve problems in atomic space, so a new thought paradigm is necessary. In this sense, the term “dimension” would really mean parameter.
No, dimensions really means dimensions of space. There is no sense in which they overlap with the ones we know; they are orthogonal to ones we know, in the strict logical, mathematical and physical sense of “orthognal”, and in the same way that the thickness of a table adds to and is orthogonal to the surface area of a table. Read the articles in http://profmattstrassler.com/articles-and-posts/some-speculative-theoretical-ideas-for-the-lhc/extra-dimensions/ . These dimensions are not some abstract concept; these are directions into which sufficiently small or sufficiently free objects can really move, do dances, pirouettes, and all the things you can do in the diemnsions we know. In particular, an object that moves in the direction of one of those dimensions has motion-energy, just as objects that move along one of the dimensions we’re used to — with an analogous Einsteinian formula relating energy, momentum and mass.
@TomA,
We could agree on the fact that the average layperson does not have the proper tools to visualize a space with any abritrary number N of dimensions, with N being any natural number larger than 4, but humans are capable of visualizing such a thing, at least any human being that also happens to be a subject matter expert.
Just as Matt has described, dimensions on any metric space are all perpendicular (“orthogonal” is the proper word in the jargon) to each other, so, as they have no projections on each other (they don’t cast a shadow on each other), there is not a chance that they could overlap.
The molecules, atoms and particles exist in the very same space that we do, within the very same dimensions, there is no “special” space for atoms, particles and quantum fields.
Kind regards, GEN
while googling for “string theory dark matter” i came across this site:
http://superstringtheory.com
wich seems to be devoted to explain stringtheory to the non-experts too.
And it’s one of the reasons Einstein took such issues with all flavors of Quantum Mechanics and QED, in addition to his objections re. determinism and causality. So the whole concept of a “spin-2 graviton” gets to be problematical. And from my limited understanding, I believe that the equations of most of these “simple” versions of string theory still assume that the spacetime dimensions act like a Minkowsky flat spacetime.
))))))))))))))))
This is Smolin’s complaint about string theory, it’s lack of background independence. Is this really still true in M theory?
Matt, thanks for another excellent article.
Just one more suggestion re. being more specific. Several times you refer to “relativity”, when you should specify “Special Relativity” or “General Relativity”. For example, the quantum field theory of the Standard Model is based only on the Minkowsky spacetime of Special Relativity; it is completely incompatible with the core concepts of General Relativity. It’s not just a technical detail that GR can’t be quantized the way other forces are. In General Relativity, there is NO “force of gravity”, just curvature of spacetime due to the presence of energy and momentum (and “stress”) within that spacetime. (We don’t feel a “force of gravity”; in open space, we just move along an inertial path through a curved spacetime: along a geodesic path according to the local metric of that spacetime. We only feel a force that prevents us from moving along that path — e.g., the electromagnetic forces between the atoms in us and those in the surface of the Earth that impede that motion, rather than letting us continue in free-fall.) All of the equations of QM and (most) QFT assume an underlying linear, flat spacetime — where, for example, “parallel transport” between points in spacetime is independent of the path taken. (In GR, things like the rate at which time evolves and the angles between vectors between to two points can depend on the path taken between them, in non-linear ways. Even concepts such as “velocity” can get tricky and subtle.) This, along with the concepts of general covariance and the non-linearity of Einstein’s equations, are what keep GR from being equivalent to a perturbative theory of gravity. And it’s one of the reasons Einstein took such issues with all flavors of Quantum Mechanics and QED, in addition to his objections re. determinism and causality. So the whole concept of a “spin-2 graviton” gets to be problematical. And from my limited understanding, I believe that the equations of most of these “simple” versions of string theory still assume that the spacetime dimensions act like a Minkowsky flat spacetime.
However, one should also note that Einstein’s Equations in General Relativity are notoriously difficult to solve and there are a wide range of possible solutions, most of which result in universes nothing like our own. We can describe the observable universe on large scales very well with a very simple Friedmann-LeMaitre-Robertson-Walker solution with a few minor adjustments for things like dark matter, but describing things like the spacetime around black holes requires completely different solutions. However, useful solutions and approximations for GR were found and exploited very early on to make testable predictions, and led to its adoption. But I would argue that we still probably don’t know what “the” correct General Relativity solution is to fully describe our real universe. So, just as with QFT, to be precise you often need to specify whether you are referring to general properties of GR (such as the above), or specify _which_ solution of General Relativity you are talking about.
@Wlm,
If you check on many other posts by Matt in this very same blog, he’s very precise in mentioning that when he uses the term “Relativity” in the context of talking about some detail of a Quantum Field Theory (any given QFT, actually), he always means the “Special Relativity” (SR) kind that is the one that applies to quantum mechanical effects.
Besides, from a purely relativistic perspective, SR is completely contained within GR, or we could say that SR is just a sub-set of GR, and this is simple to prove: if we use the complete set of equations for GR for the case of inertial reference frames (the only types of reference frames considered by SR), which it would mean to assign zero to certain terms in the complete set of equations, and them we simplify the equations by eliminating the terms that cancel out, voilá!, we end up having a simpler set of equations that are exactly the SR equations.
Regarding gravity not being a force from a GR perspective while at the same time from a Standard Model perspective it is a quantum mechanical force, with its own vector boson as mediator, there is no issue with that.
The simplest way to show that there is no real issue with that is by remembering Niels Bohr’s Correspondence Principle.
Kind regards, GEN
A graviton (spin-2), is it not a tensorboson?
It’s a tensor boson… but specifically a spin-two tensor boson. There are many types of tensor bosons, because the term “tensor” is very general, much more general than “vector”
ofor “scalar”.Thank you.
BTW of is Dutch for or 🙂
Gaston,
Yes, you are right that Special Relativity is, by design, a subset of General Relativity. One of Einstein’s criteria when devising GR was that SR is what is obtained in the “limit” of zero curvature. But it is a very proscribed subset (with a specific, constant Lorentzian metric) that does not reflect the complexities and subtleties of GR, either mathematically or geometrically. This is especially true when you have equations with derivatives based on a linear time parameter. So basing a QFT just on SR, and not GR, has some fairly radical consequences — like being able to write consistent QFT equations from a Hamiltonian and solve them perturbatively (or non-perturbatively), which still hasn’t been accomplished for a realistic curved universe in 3+1 dimensions.
But I wrote my post because, from some comments above, some people seem to be confusing the term “relativity” with “Einsteins’s theory of gravity”, when Standard Relativity (that was incorporated into QED and later QFTs) has nothing at all to do with gravity. Hence my suggestion.
Cheers,
Wlm
@Wlm,
You have a point with that.
I’m not an expert on these matters, but it is my understanding that the problem that you describe is mostly significant for those events of our universe where you need to combine both QFT and GR equations to describe it, like say, the collapse of a massive star, including black holes but not limiting to them.
Besides this, the combination of QFT and GR equations might also be significant to further the study of the hierarchy problem (this is a guess of mine).
Differential geometry is a relatively new segment of mathematics, it is a rather complex subject to tackle, in particular when you consider its use to apply it to describe GR, since you have to find a way to work out how to mix and match constraints from non-euclidean spaces (the types that are useful for GR) and the physical constraints required for GR, such that it all makes sense.
Kind regards, GEN
String theory is good because it gives you a “holy grail”? Well, I guess that’s consistent with my general impression of string theory: a quasi-religious enterprise pushed by a small band of die-hard enthused zealots. Evidence-wise, it’s a complete bust. String theory is based on SUSY, and SUSY is on life-support, it’s predictions not having been confirmed by LHC.
@M Mahin,
Even though the LHC data collected so far has put into question certain variants of SUSY, there are other variants of SUSY that are still into the competition in rather good shape, so, it is not proper to assert that the LHC has ruled out SUSY en bloc.
Why must there be finite dimensions? Yes, I believe every variable (of nature) has a finite range or else the universe would not exist, i.e. either drift (tear itself apart) to infinite space or collapse (dissipate) to “absolute zero” (as opposed to ZPE state).
We perceive ourselves living in a 3D world because that is how we elected to write our equations, x,y & z. An astronaut in orbit cannot make the distinction between linear and rotational motion without an external reference frame, he will only see changes of speeds (velocities w/o directional cues). Is it because the dimensions are infinite and we and everything else are moving in curved space? Every point in space is moving relative to the next, adjacent point, in infinite directions, but to an external observer you see a continuum of space (3D volume).
“The biggest problem in this whole business — and that is why I am writing these posts — is that no one who is prominent in the public string debate (Greene, Woit, etc.) is sufficiently precise about what they are talking ”
…………….
so why do you think these brilliant physicists are not being precise.?
covering their bases ?
“Each field takes a value everywhere in space and time, in much the same way that the temperature of the air is something you can specify at all times and at all places in the atmosphere.”
Except that temperature can be measured.
Writing F(x,y,z,t) does not imply that F has physical existence. A field thus takes values at points of the spacetime coordinate domain, not in real space. In the same sense that the lattice of lattice-QCD is not a physical latttice.
The fact that QFT is only an effective theory implies directly that the fields do not really exist. A Lagrangian is not something that flies around in space, either. In QFT particles do not even have a position. That is why everything is described in terms of impulse p.
And therefore particles cannot be said to be ripples in a field; they are described (approximately) by field ripples, that is something else.
Martin,
Well said. I had the same response when reading Matt’s description. “Temperature” came about because macro-sized things were discovered to expand or contract in a way that corresponded to how “hot” something seemed. It was something that could be used consistently to get useful experimental results. It was only later that it became incorporated into a methematical theory of heat. And then it turns out that it really corresponds to a statistical, probabilistic average related to how often and how hard (with what “kinetic energy”, in Newtonian physics terms) a large group of molecule-sized objects bang into each other (or a measuring probe). Does it exist “at every point”? Only theoretically. What’s the “temperature” inside a proton? Does the space between molecules in a very rarified gas have a real temperature, or only a theoretical expected value?
This seems like an example of the “physics is math; math is physics” logical error. Another one: that Fourier analysis shows that every wave is the sum of an infinite number of harmonics of waves up to those with an infinitessimally small wavelength. Does a sound wave — a group of a large but finite number molecules shoved closer together then farther apart for a brief period of time — vibrate with all possible wavelengths at every point? It’s an excellent model, but is it “real”? Of course not. But that’s what you need to assume to make the math work out right, and to get answers that correspond to things on the scale that we can measure.
The added problem with QFT is the very nature of the “fields”. These are very abstract things that are “vibrating”: an infinite space of an infinite series of complex-valued functions with some very specific properties under some very specific kinds of mathematical operators. That’s why Matt can’t quite explain “What’s vibrating” — because the answer is hard to even describe, much less translate into anything someone without detailed knowledge of the math can understand.
Do these “fields” actually exist? The correct answer is that no one knows. It seems clear that, whatever subatomic “particles” really are, they are absolutely nothing like tiny little billiard balls. We may have absolutely nothing in the macro world that is anything like them, and the descriptive label “particle” is a complete misnomer. But just like our very early understanding of temperature, that does not mean that our current mathematical way of describing their action will turn out to be of much use in describing what is actually going on and what they actually are. It may only provide some of the criteria to help us tell whether future efforts to better describe them are getting things right or not.
I also have a question:
The Higgs Boson, a ripple in the Higgs field, has spin-0.
The Photon, a ripple in the electromagnetic field, has spin-1.
The Graviton, a ripple in the gravitational field, has spin-2.
Different fields, different ripples, different functional specs , different technical specs?
To Matt Strassler: “a graviton: a spin-two particle that is a ripple in a gravitational field.” -> why is a graviton predicted to be “spin-two” particle? instead of any other spin:0, 1, 3, 4.. etc.?
Oh! I am sorry. Rick mentions the same thing in the comment just above his last comment. I noticed it after I posted the above comment and there was no way to cancel my comment. Anyway ,when you get time, I would also like to see your opinion on this.
If the critical dimension in bosonic string theory is
D = 2 – 2/(1+2+3+…), then doesn’t that mean D = 2 since the sum in the denominator diverges? Since D has to be at least 4, doesn’t that disprove string theory?
Your premise is wrong, so your conclusion is too. There are some 2 (i.e. 1 + time) dimensional string theories, but the critical dimension in what people usually mean by bosonic string theory is 26 (i.e. 25 + time). There are other problems with bosonic string theory, which is why superstrings (with critical dimension 9+time = 10) are generally what you hear about.
Matt: There is a discussion going on another blog that actually the apparently divergent seies 1+2+3+…. should be interpreted as -1/12 by regularization etc. Then the formula quoted by Rick would work OK as 26. What is your opinion on this procedure?
Kashyap,
See my response to Rick above. I think the formula is ok for total number of dimensions (assuming 1 of time the rest of space) but doesn’t this only hold for certain String theories? Regardless, it is bad form to write it as D = 2 -2/1+2+3… (unless you are trying to impress/confuse someone), rather D = 2 – 2/ζ(-1) = 26.
@S. Dino. You do not have to believe in String Theory to be amazed by such results. I am not sure but the theory may give 1+2+3..before giving zeta function. Perturbation theory if you take literally as it comes diverges anyway. For one thing, the summation of such divergent series was given by all time great mathematicians, such as Euler, Borel, Ramanujan and others who did not know anything about ST. Motl’s blog has several references and one excellent video lecture by Carl Bender explaining this stuff. Good mathematicians accept these results. Analytic continuation is also acceptable in general. By the way, I asked a question about D for fermions and such infinite series give also a correct answer D=9 (10 with time). Disputes about validity of ST is altogether a different question. For me the most amazing thing is that such amusing mental gymnastics gives agreement with experiments (sometime) which people perform using things which they can hold in their hands and are as real as one can get!!
Can you please shed light on this whole thing about why 1 + 2 + 3 + 4 +… equals -1/12 and its relation to string theory? I’m sorry, but I have no idea why that divergent sum must equal -1/12. Any light shed on this issue would be greatly appreciated! I’ve seen many people on many different blogs talk about this (eg. Lubos).
Rick,
Of course 1+2+3+… = infinity. However 1+2+3+… = 1/1^-1 + 1/2^-1 + 1/3^-1 = ζ(-1). IF the extended definition of the Zeta function is used ζ(-1) = -1/12. You can extend the definition of ζ(s) to s < 1 using a process called ‘analytic continuation’. Using this in a consistent manner (regularization of divergent series) it is possible to have some unwanted nasty infinities cancel out. This is deeply linked to the renormalization of divergent integrals in String Theory. Hope this helps…
Oops, meant 1+2+3+… = 1/1^-1 + 1/2^-1 + 1/3^-1 +… = ζ(-1). Forgot the +… after the 1/3^-1 above.
S. Dino,
Thanks very much! That was helpful. Do you, by any chance, know why one can get the same answer (-1/12) by doing funny tricks with the series 1 – 1 + 1 -… = 1/2. I don’t know if you have seen the Numberphile youtube video which shows a tricky way to evaluate 1 + 2 + 3 +… without using the zeta function.
I am glad that you continue to help to explain the basis of the physics approach as a fundamental description. This is important in maintaining some hold on the purpose of this foundational math as a attribute. To me, it is very important in light of the mathematics chosen, as a descriptor of the given reality.
I’ve found your expositions to be remarkably clear and hugely illuminating, and I’m deeply grateful for you efforts. Thank you.
“Vague and with loopholes”
I guess that is what I don’t like about string theory. Its predictions, as you noted, are vague and with loopholes. To me that is not a very satisfying construct. To me a useful scientific theory, regardless of how it is arrived at, should be testable with todays’ technology or technology we can strive for in the near future. Theories that do not meet this criterion should be put on the shelf until they do become testable.
It helps physics ADVANCE when theories that make very specific predictions and without loopholes are found to be in error by experiment or discovery thus forcing a scientific revolution and ultimately the development of a new theory whose equations reduce to the older theory under the conditions it was successful but also predicts new phenomena that accounts for the experiment or discovery that the older theory could not. And so it goes…advance after advance…scientific revolution after scientific revolution…
If anybody thinks the physics of the 31st Century will look like the physics of the 21st Century … good luck with that.
By the equivalence principle, it must look *almost exactly* like it! 😉
People apply a double-standard to String Theory, asking it to predict everything, and complaining when it can only make predictions once assumptions are made. Well, field theory also makes only vague predictions with loopholes, until you make assumptions: that there are electrons and photons and quarks, that they have masses with certain values, that gravity is not important for particle physics, that the universe has a roughly flat space-time with slow expansion — etc. Newton’s laws do NOT predict the planets’ orbits, until you first measure the current location and velocity of the sun and the planets. Every theory requires some level of input before it can make precise predictions; if you don’t put in any inputs you get vague predictions with loopholes. For example, without inputs, Newton’s laws only predict that you *can* have planets orbiting stars; they don’t tell you whether any given star actually has planets, and they don’t tell you the radii of the orbits or whether there are several planets orbiting in a plane or anything like that.
This is what I’m talking about when I say that people are not precise in their thinking. They apply one standard to one theory and a different standard to a second theory, and complain that the first theory is worse than the second because it fails their expectations, even though the second theory would fail the same set of expectations if those expectations were applied to it.
Just in case you mean people like me when you say “some people”; I am not applying a double-standard to String Theory, asking it to predict everything. I am asking it to predict something, anything, that is testable beyond what GR and QFT predicts. Input whatever assumptions seem reasonable to you – do you get any testable predictions beyond GR and QFT?
Also you note that the string equations are consistent with Einstein’s Relativity. So let me ask you a simple question. If Relativity turns out to be wrong is String Theory then also wrong?
I think the ability of a theory to make many precise predictions, and the value of the theory that it gets as close as we can get to describe our world, are two different things. A theory may have difficulties to make predictions, at least on our current incomplete level of understanding, and with experimental technology confined to our present capabilities, but still may be, in the long run, the best way to describe (or even “explain”, althoug the meaning of this word is not precisely defined here) our physical world.
Einstein thought that some of the predictions of his theories might never be testable because the effects are really small, but this did not stop him in believing his theory is correct. Only some decades later technology and ideas for experiments have sufficiently advanced to experimentally proof the existance of gravitational lenses predicted by Einstein et al.
This suggests that theories with predictions that are difficult to test should not be thrown out too early. They might be the best theory available, and a clever idea for a new experiment or an unexpected technological advance may put new ways of verification more rapidly into reach than expected.
There is no reason to expect that Nature´s laws are, in the set of all possible laws, those that are most easily understood or experimentally detected by human beings.
Hi Markus,
Einstein put forth GR in late 1915. Observations of the deflection of starlight by the Sun in accordance with the theory came in 1919. I never intended that EVERY prediction of a new useful theory must be testable by the technology of the day or near future .
Newton used Kepler’s Laws of planetary motion to inductively reason the equations for gravity, that is, Newton started from the facts determined by previous experiments.
As far as I can remember, the only idea that Newton “inserted” out of nowhere into the theory was the inverse-square law: Kepler’s Laws gave him no insights regarding this, and it was the only way he found to make gravity work in such a way that he could calculate the orbits and periods for the known planets.
More and more I’m not buying it. As pretty as it is, it is pure inductive logic. Statistical probabilities based on mathematical models doesn’t cut it for me. There is something inherently wrong. Rather than using deductive reasoning we have devolved into quasi inductive reasoning science. It could be that I’m still thinking 19th century physics of cause and effect, but I don’t think so. What does the evidence of experiment show and what does it mean without preconceptions?
Physics has never used deductive reasoning, since it became successful. It’s always been inductive; you inductively reason from experiments.
It is my understanding that getting the Mercury perihelion correct was more impressive than the light deflection experiments, even though the former was long known. In truth, it is the very fact that the Mercury anomaly (for Newton) was known and that scientists had long tried to account for it (through means determined to be ad hoc) that made it so impressive when GTR predicted it directly. I’ve written this quickly in passing, but have discussed it in a book of mine….
Part of this balance is that the initial light-deflection measurements weren’t very convincing. Yes, the other suggestions for how to fix Mercury’s orbit tended to be partly ad hoc, but they showed that there were multiple possible explanations, not all of which required something quite so radical as a rewriting of Newton’s gravity! Dust was another possibility, though the case became weaker over time.
In any case, many people did not believe Einstein’s theory through the 1920s, despite the postdiction of Mercury’s orbit and the weak confirmation of the prediction of the bending of light. The evidence wasn’t viewed as strong. Only in the 1960s, when more tests became available, did the case become more convincing.
Let me say this more clearly.
Mercury’s orbit was shifting by a certain amount per year — that is, by a certain angle. Many people came up with models for where that shift could come from. Einstein’s theory predicted it in a single shot. That’s impressive. But still, it was a prediction of one number.
Supersymmetric grand unification, similarly, predicts one number… which you can phrase, if you like, as the mixing angle known as theta_weak that tells you how electromagnetism is a mix of hypercharge and weak isospin. Do we therefore believe in supersymmetric grand unification? Some people do. Some people don’t. In this case, the prediction wasn’t even entirely a postdiction, because the data got better and the prediction survived the improvements.
Generally, we don’t start believing theories as a community until they start predicting many things… many numbers, or even entire functions, which we obtain through many different types of measurements. It’s one thing to be impressed, another thing to be convinced. When Einstein’s theory starting giving correct answers for multiple types of measurements, including some that humans could set up themselves, that’s when the balance really tipped.
My model might be under investigation at Fermilab. Have you tipped them?
No.
Just to support Matt’s point regarding this.
The existence of the planet Neptune was first predicted by Le Verrier during the first half of the XIX century, based on the irregularities measured in the orbit of Uranus.
Then Neptune was discovered just where Le Verrier had predicted that it should be.
Also, during the XIX century, Bessel had predicted that (Alpha) Sirius (the star) might be actually a binary star system, accompanied by a smaller (and dimmer) but very dense star, by doing calculations based on the “wobliness” of the trajectory of (Alpha) Sirius.
Beta Sirius was discovered in 1971, and Bessel’s predictions were validated.
Because of these events, some astronomers during the XIX century proposed that the anomaly in Mercury’s orbit could be due to the gravitational influence of an unknown planet.
The truth is that Einstein’s postdiction of the actual deviation was not that impressive. What was really impressive at the time (1916) was the qualitative and quantitative prediction that heavy bodies like stars can warp space-time in such a way that the path followed by light is not a straight line in the vicinity of said massive objects, or that heavy bodies like stars or planets can warp space-time in such a way that time runs slower when measured closer to the massive object.
The slowing down of time by massive bodies could not be detected by experiments until the 1970s, with the use of atomic clocks.
It seems pretty clear that any expert in HEP may realize, sooner rather than later, from a conversation with somebody else if that other person is or is not an expert in the field, too.
Many theories predict mass of particles in much more explicit way, than the string theory does already (www.blacklightpower.com/wp-content/uploads/theory/TheoryPresentationPt3.pdf, arxiv.org/ftp/arxiv/papers/1308/1308.1849.pdf, http://www.cosmology-particles.pl/files/LP.pdf, quantoken.blogspot.cz/2005/02/guitar-predictions-of-muon-mass.html) We shouldn’t forget Heim’s theory in this connection.
String theory doesn’t predict the masses of the known particles at all, or even what the types of particles that we find in our universe should be, so you’re setting a very low bar.
I do hope you will say a few words about the cancellation of gravitational anomalies. It was the first instance of a recurring phenomenon appearing all across string theory. Namely the fact that string theory is overly constrained and could prove inconsistent in dozens of ways, but never does.
You’ve said this in a strange way, and I’m not sure I completely understand or agree with the way you said it — maybe you can clarify it? I would have said that most string theories proved inconsistent. And so instead of your statement that “string theory is overly constrained and could prove inconsistent in dozens of ways, but never does“, I would have said “… and usually (but not quite always) does.” The amazing thing is that after all the dust settled, and most string theories were completely dead, there were five left over that were, remarkably, consistent… and that even more amazing (next post) they all turned out to be related to one another.
Indeed, my formulation was not precise. By “string theory” I had in mind the 5 five remaining consistent string theories. My point is that after the worldsheet analysis which selects them, there is a long list of consistency constraints, starting with the cancellation of the local gravitational anomalies in the effective spacetime supergravity, which in all likelihood should have failed. The fact that they did not is what really launched the subject. For instance the anomalies of type IIB supergravity cancel because an over-constrained system of 3 linear equations in 3 variables has a non-trivial solutions. Type I looks naively inconsistent, but, the theory miraculously cures itself through the Green-Schwarz mechanism.
Thanks…
Matt: your remark” in particular, whether such theories can really be stable, and thus really describe any long-lived universes.’ Tell me if this is correct. Without SUSY Higgs mass is so high and/or Higgs is unstable so that universe is not stable. Is this correct?
It’s not correct, because there are several different notions here, and because you’re making an error.
First there is the issue of the hierarchy problem, also known as the naturalness problem. A universe with the Standard Model and a low-mass Higgs, with nothing else nearby, is, among similar theories, extremely unusual. That does not mean that it is extremely unstable.
Supersymmetry at the LHC scale is ONE OF MANY ways to solve or mitigate this problem. You are making a big mistake if you think supersymmetry is the only possible solution.
Then there is, separately, the issue of *metastability*. It turns out that for a Higgs of mass 125 GeV/c^2 and a top quark of about 173 GeV/c^2, the Standard Model may be very slightly unstable (“metastable”). This is not a big deal since the universe will still probably last far, far longer than it has so far. In short, this is not a problem that requires a solution — it’s perfectly fine, as far as our universe is concerned, if this is the case.
When I say that these problematic string theories don’t describe stable long-lived universes, I mean that the universes that they could host would last less than a tiny tiny fraction of a tiny tiny fraction of a tiny tiny fraction of a second. THAT’s instability.
Thanks for clarification. Unless I misunderstood there is a possible typo in “In the simplest contexts, superstring theories in more than 9 spatial dimensions have anomalies (and string theories that aren’t superstrings have anomalies in more than 25 spatial dimensions.) Shouldn’t “more” be “less” ? i.e to avoid anomaly you have to go at least 9 and 25 dim resp?
No, there’s no typo. Roughly speaking: you need to have 9 and only 9 to avoid anomalies; 6 is bad, 13 is bad, etc. You can have less than 9 only in a subtle way, by replacing the missing dimensions with something that isn’t really geometry but leaves a comparable number of particles in the theory (very technical subject.) You certainly cannot have more than 9 — within weakly interacting string theory. You can have 10, in fact, if interactions are strong, but again only in a subtle context to be described soon. You cannot have 11 or more in a superstring-like context.
Thanks again. I see that there must be equations where you have to put 9 or 25!!. This proves for the nth time that it is too much to hope for that one can understand theoretical physics without math!!
In the end, yes, the math really matters.
Theories in physics must follow certain rules:
They must be mathematically consistent, as they are deduced out of the use of a certain “section” of math, then they also have to be consistent with existing principles and concepts of theoretical physics, and if the theories are “lucky” enough, they are also favored by nature, that is, they are validated by experiments.
Once you take into account the constraints to the math applied by the consistency with physical principles and concepts and any additional constraints that could come from the experiments, you can just use the math to deduce new properties, concepts and equations to the theory.
So, math is the language of science, but in science, the math that is used is bound by certain constraints.
Excellent article, as always, very exciting stuff and well described, helps even the layperson, like myself, visualize and dream, thank you Prof.
Even, after reading (and studying, playing mind games with various permutations of all ideas, theories) I still converge to a universe which is merely an ensemble of distorted space-time. Here is one of my dreams (illusions? :-));
A large, circular flat (2D), uniform, flexible fabric … stretch it tight, up to but not beyond it’s plastic deformation stress-strain point, … fix the circumference solid in a complete 360 degree clamp. Now go in center of this enormously large surface area fabric and pinch a 1-2 inches in length and fold it once but careful not yield (go beyond plastic deformation) and release, … it will “spring” back to it’s original shape. Now fold it again us many times necessary to yield the two ends (pinched ends) so it stays folded.
Here is the dream part, :-), assume the fabric is space and the “yielding”, the change of parameters, characteristics, is time. Yielding of the boundaries of this small section of the fabric is like time dilation caused by
the rotations of this affected space and at some point when it yields, reaches a critical velocity (since a section of space is changing at different times, vectors will be created) it will take hold and keep those parameters, characteristics.
This critical velocity would be the “constant” speed of light, the minimum rate of change of space to maintain stability.
Finally, if you assume this pinched section to be the quantum of “distorted space” apply it in a 3D world and an infinite array, in all 3 directions, of these quanta. … (gravitons?)
Do I need professional counselling? 🙂
An additional point. Even without String theory the existence of a landscape is strongly supported in cosmology. So I don’t mean to imply that the landscape counts against string theory, quite the opposite in fact. One could argue, I think . that our current best understanding in cosmology which is well supported by observation counts as support for string theory. I think many cosmologists think this way.
Strongly implied might be better.
. An additional point. Even without String theory the existence of a landscape is strongly supported in cosmology. So I don’t mean to imply that the landscape counts against string theory, quite the opposite in fact. One could argue, I think . that our current best understanding in cosmology which is well supported by observation counts as support for string theory. I think many cosmologists think this way.
That may be; but I would argue that the landscape fails to explain some obvious things about LHC physics, so that cuts the other direction.
In response to Matt
I agree Matt that not “all” aspects of the laws of physics can be environmental, there has to exist a meta theory of the landscape itself. Perhaps the Noether symmetries are fundamental in every vacua state.
I still think the landscape is an unfortunate development though it is believable ., especially based on our best cosmological theory of origins , eternal inflation and quantum tunneling in third quantization
Let me clarify my point about SUSY. I know SUSY isn’t direct evidence for String theory, but String theory needs SUSY, so the lack of SUSY at CERN leaves us without something String theory needs. I think that’s not good news for String theory. It’s true that SUSY can break outside the reach of the LHC in string theory, but a SUSY breaking at such high energy means it can’t do the things we expect it to like solve the Hierarchy problem. This will cast a shadow over SUSY for sure and consequently string theory which needs SUSY. So I don’t think I am in contradiction here.
I still don’t agree with the logic of your third paragraph.
Ruling out SUSY that can solve the hierarchy problem is a bigger problem for SUSY on its own. It would make SUSY not as interesting. That does not necessarily mean a String Theory which spits out a non-hierarchy-solving SUSY is also not interesting, because SUSY is not the only feature of String Theory.
Matt–
Just a terminological/semantic issue that might be a source of confusion for readers whom I’d like to point to this article — it regards the way that you say that “quantum mechanics” was historically not capable of handling relativity and was replaced by quantum field theory.
As you well know, quantum mechanics is the overarching framework of Hilbert spaces, operators, Born’s rule, etc., that contains as examples nonrelativistic systems, quantum field theories, string theory, etc. We often refer to (0+1)-dimensional QFTs as “quantum mechanics,” when what we really mean is “nonrelativistic quantum mechanics”, i.e., a quantum-mechanical system with finitely many degrees of freedom.
Quantum field theory is a different model inside quantum mechanics that improved on the nonrelativistic quantum mechanics of finitely many point particles, but QFT was not a replacement for quantum mechanics as a whole, which has survived for a century.
I just don’t want lay readers (including young people who don’t this stuff yet but might be interested in going into physics) to get the idea that quantum mechanics was overturned by quantum field theory, or, at least so far, by anything else.
@Matt297,
It was Paul Dirac’s equation of the electron that offered the best and closed approach of Quantum Mechanics (QM) to integrate itself with special relativity.
It was a very good interim solution, but it was far away from being able to explain how matter interacts with the electromagnetic force bosons or photons (that would require QED to really solve and explain).
QM was able to predict quantum entanglement, but it did not predict the infinite stories that pertain to the paths of particles … That would have to wait for QED to predict that.
It took the genius of Dick Feynman’s intuition to think about the double slit experiment from a completely different perspective to fully grasp this concept of a particle running down all possible paths at the same time to unleash and let free the concepts behind QED.
Kind regards, GEN
Your point is a fair one. Let me think about how to handle it. I usually use “quantum theory” to mean “quantum mechanics the framework” and use “quantum mechanics” to mean “quantum mechanics the particle theory and as taught in undergraduate courses.” In this post I am indeed in danger of misleading readers about this point… though the fact that the word “quantum” appears in “quantum field theory” and in my discussion of string theory should help mitigate this.
I concur with you on these caveats: QM is a particle theory and not a QFT.
QM (the particle theory) and QED (the QFT) make the same numerical predictions of many experiments, but the equations and explanations are very different.
QM, the particle theory is a nice first try, but is neither as complete a theory nor a proper approach to really understand and describe all the experiments.
GEN– With all due respect, what are you talking about?
Look, I have other work to do, but on the off-chance that this discussion leads to confusion among readers, classical field theory is a branch of classical mechanics, and quantum field theory is a branch of quantum mechanics. QED is a kind of QFT which is a kind of quantum-mechanical system, just as Maxwell’s electromagnetism is a kind of classical field theory which is a kind of classical-mechanical system.
Every quantum-mechanical theory, including any QFT, has a Hilbert space, satisfies a Schrodinger equation over that Hilbert space (even if the wave-function is actually a wave-functional), and has the Born rule for computing probabilities of measurement outcomes. Every quantum-mechanical theory with continuous degrees of freedom, whether nonrelativistic point-particle theories or quantum fields, has Feynman path integrals in it. The nonrelativistic harmonic oscillator has path integrals just as does QED.
The discussion with Matt Strassler is just over terminology—whether one should use “quantum theory” for the overarching class that contains nonrelativistic quantum-mechanical systems, QFTs, and string theory, or whether one should use the term “quantum mechanics” for that overarching class. I have argued that to avoid confusion or ambiguity, one should not use “quantum mechanics” to refer solely to nonrelativistic systems of quantum particles, but that one should explicitly say “nonrelativistic quantum mechanics.” That’s the entire point of this discussion.
I agree about the terminological discussion as the main issue that you were arguing.
Factical sciences and other professions (like, say, engineering) that derive from factical sciences need the use of unambiguous terms and definitions.
Matt297 is correct, GEN, that this is purely a matter of terminology. Some people would say “classical field theory” is a branch of “classical mechanics”; others would say it is a branch of “classsical physics”; and this is purely an issue of definition of terms. There is a similar issue about how the words “quantum mechanics” are used; as I said, I use “quantum theory” whereas Matt297 uses “quantum mechanics” to describe the full range of quantum phenomena. No one is right or wrong; there is simply an issue of needing to be clear to non-experts.
Matt–
So I’m basically just suggesting a slight clarification — maybe something as simple as modifying your statement to “But nonrelativistic quantum theory [my edit] wasn’t complete enough to describe everything physicists knew about, even at the time. Specifically, it couldn’t describe particles that move at or near the speed of light… and so it wasn’t consistent with Einstein’s theory (i.e. his equations) of relativity.”
Maybe also in parentheses as note about this terminological ambiguity, something like “(A note on terminology: I use the term “quantum mechanics” here to refer just to the specific nonrelativistic equations that needed to be replaced by quantum field theory, whereas other use “quantum mechanics” to mean the whole framework of using quantum theories, in which case quantum field theory is just an example.)”
Thanks!
I mean, Sakurai’s classic book on quantum field theory is called “Advanced Quantum Mechanics.”
Meanwhile, Wikipedia is rather schizophrenic about all of this. (http://en.wikipedia.org/wiki/Quantum_mechanics) It says that “quantum mechanics” and “quantum theory” and “quantum physics” are all synonymous, but then also says of quantum mechanics that “It is the non-relativistic limit of quantum field theory (QFT), a theory that was developed later that combined quantum mechanics with relativity.” The Wikipedia entry for “quantum theory” is just a menu of redirects to other entries.
As I understand it, the uncertainty principle is a natural consequence of certain properties of the math (the equations) used in the quantum theories.
The equations used have a general form that contains eigen values and eigen functions.
The quantum numbers that determine the (quantum) state of the system are contained within the eigen values.
The eigen values determine the quantized properties of the system. Certain combinations of properties have such behavior that both the values of their quantum properties cannot be determined (measured) with certainty at the same time, even though they do allow to be measured with certain one at a time.
These certain pairs of quantum properties that show this “weird” behavior are described as non-commuting operators.
Again, it is a natural consequence of the math used by the equations. Just as Benjamin Peirce said: “Mathematics is the science that draws necessary conclusions.”
Great series of posts. I have a question about how the uncertainty principle comes into play in both String theory and QFT.
Does the uncertainty principle have to be included ad hoc into both these theories? Or does is “pop out” of the equations somewhere? For example, in QFT, if I write down a Lagrangian, how do I know it obeys the uncertainty principle, or does it not have to?
I think an uncertainty principle arises in pretty much any theory with waves. It’s really a property of Fourier Transforms, which are pretty fundamental to the study of anything that oscillates.
A function that looks like a lump of some kind can be written as a sum of sinusoids at different frequencies. The narrower you want to make that lump, the wider the set of frequencies of different sinusoids you have to add together to get it. So how precisely the lump occupies a particular position is inversely proportional to how precisely it occupies a particular frequency. That’s the gist of the uncertainty principle.
@Xezlec,
Any classical physics phenomenon that can be described by waves and it includes wave packets will present a behaviour identical to the uncertainty principle, as it pertains to nature of waves.
But there is an additional aspect to the uncertainty principle in all quantum mechanical theories that is not present in classical theories, and that is the behaviour of non-commuting operators. I will leave the mathetical side of this for now, and will describe this aspect from a more “physical” perspective.
The state of any quantum particle is described by a certain set of integer numbers, aptly called quantum numbers. These are the ones responsible for the fact that certain physical properties of the particle are quantized, that is, those properties can only have a discrete set of possible values as their magnitude.
With experiments, we can measure any one of these quantized properties and get a precise measurement of its value at the moment of the experiment.
Many pairs of these properties can be measured at the same time and we can measure very precises values of these properties, but there are certain pairs of these quantized properties that cannot be measured at the same time with precision: if we measure one of them with precision, we get an uncertain value for the measurement of the other properties of the pair.
This is a rather unique behaviour present in all quantum mechanical theories and it is not present in classical physics.
We can use the math of wave packets to obtain the widely known expressions for the uncertainty principle, the expression with the “thresholds” to the uncertainty.
But with the classical physics behaviour alone we can’t predict these pairs of properties that cannot be measured with precision at the same time.
This is a very important aspect that sets apart quantum mechanical theories from classical physics theories.
Kind regards, GEN
Do I understand that even in absence of supersymmetry, both fermions and bosons can be understood as vibration modes of the strings with no essential difference between the modes?
No, you still need something on the string which distinguishes fermions from bosons. This is a bit subtle, and I didn’t describe it carefully in this post. Maybe I’ll think of a way to do a better job, but what I hinted at — that there might be supersymmetry in nature but it may be profoundly hidden and not look anything like what you might naively have expected — was an attempt to paper over some subtleties.
“In addition, for a theory of superstrings to be consistent, the strings have to [with a few subtle caveats] move in a space with 9 spatial dimensions.”
Interesting- I’d always been under the impression that the extra dimensions were necessary for a theory that could reduce to the Standard Model, but not for the mathematical consistency of the theory itself. Are you able to give any sort of account of how string theory falls apart in fewer than 9+1 dimensions?
There is something called a “gauge anomaly” which afflicts certain field theories. A field theory with a gauge anomaly has the property that everything you try to calculate — say, the annihilation of two particles to make two other particles — gives you zero divided by zero. Obviously, a theory where every prediction for experiment is 0/0 is completely useless and describes nothing.
The tricky part is that this gauge anomaly is not visible if you do classical physics. It is a property that can only arise in quantum mechanics. As a result, there are quantum field theories that seem to exist (because they have a classical analogue) but do not (because they have a gauge anomaly.)
String theories, and other theories of quantum gravity, can have gauge anomalies and gravitational anomalies. Similarly to field theories, a string theory with such an anomaly doesn’t make any sense; it may seem to have a classical analogue, but as a quantum theory, every prediction it makes is 0/0.
In the simplest contexts, superstring theories in more than 9 spatial dimensions have anomalies (and string theories that aren’t superstrings have anomalies in more than 25 spatial dimensions.) In fewer than 9, you have to be a little careful about what you mean by dimensions, but essentially the result is the same: if you want to avoid anomalies, you either need 9 dimensions or you need to replace some of those dimensions by something more subtle.
But in any case, this has nothing to do with getting the Standard Model out of the theory. It’s a mathematical consistency condition, without which the theory cannot be used to predict anything at all, not even about imaginary universes.
Very informative post indeed (at least to me), thank you very much! How’s the winter school? What did *you* learn from it (even though you were teaching there)? 🙂
Hmm. I learned a lot of things (as I always do) from Raman Sundrum, one of the other lecturers, but not about what he was teaching. I learned some things that I hadn’t thought through carefully from Gino Isidori about certain models of quark and lepton masses. And I learned how to present the Higgs discovery to students, and about the subtleties that one needs to discuss to make sure they get a clear picture. Maybe other things will occur to me…
If you’re envisioning a distorted lattice, you’re actually envisioning a distortion of space itself — a gravitational wave, whose quantum is a graviton, not a photon.
Matt
Are you saying in the above sentence that “space” is the “field” in which a graviton may be found to be a distortion? That is the gravitational wave is propogating through the field of space?
Roughly speaking yes, but I was not entirely precise. In Einstein’s gravity, you have to be careful because you can choose different points of view. The “field” in which the graviton is a distortion may be thought of as the distance-measuring field (called the “metric”). But a distortion in the distances between grid points can also be viewed as a distortion in the grid itself.
What’s independent of your point of view is that energy and momentum can be carried from one place to another, and the amount transferred and what happens when it hits something doesn’t depend on whether you view the wave as a distortion in the grid or in how you think of the distance between grid points.
What clarity! A perspective like no other. Will be looking forward to your further posts.
Thanks Matt for this very informative post. String-M theory may very well describe our universe, but as I have argued before, it’s not very predictive. It was hoped early on that String theory would make a unique prediction that looked just like the universe we live in. These hopes were dashed with the discovery of the landscape. Both cosmology and String theory point to the TOE being a environmental model. This puts physics in an awkward position with regard to the falsification possibilities of these models. I think the sense that string theory is correct would be greatly boosted if we find SUSY during the next run at CERN. You can have SUSY without string theory, but not the other way around. SUSY might break outside the range of the LHC and String theory could still be correct, but the lack of SUSY would raise doubts if physics took the right turn with String -M theory in my opinion.
I don’t entirely agree with these statements.
“It was hoped early on that String theory would make a unique prediction that looked just like the universe we live in. These hopes were dashed with the discovery of the landscape.” Many of us never had much hope and the hopes died more slowly than you think… because the `landscape’ of possibilities was suspected long, long before it was “discovered” within the theory. I’ll describe this later.
“Both cosmology and String theory point to the TOE [Theory of Everything] being a environmental model.” I don’t agree. In any theory, some things are environmental, others are not. Nothing in either cosmology or string theory indicates that all aspects of the laws of nature are environmental.
” I think the sense that string theory is correct would be greatly boosted if we find SUSY during the next run at CERN. You can have SUSY without string theory, but not the other way around.” Your second sentence logically contradicts the first one. Yes, you can have SUSY without string theory, so finding SUSY tells you nothing about string theory; and even if you can’t have string theory without supersymmetry, that supersymmetry may be greatly hidden from you in many different ways, so not finding it tells you, again, nothing about string theory.
Is there really no hope of string theory making any set of testable predictions? Surely there must be something detectable in our world that would be different if strings exist. Black Hole behavior, very early universe structure or behavior maybe.
You’re asking a profoundly ill-defined question. The reason your question is bad is that you’ve not specified it. Are you talking about a prediction that string theory makes that is true IN ALL ITS FORMS (VACUA)? Are you talking about a prediction that string theory makes that is true IN ITS VACUA THAT ARE DESCRIBED BY SUCCESSIVE APPROXIMATION (perturbation theory) but not in others? Are you talking about a prediction that is true only in those vacua where strings that have lengths near the apparent 4 dimensional Planck scale? Are you talking about a SPECIFIC VACUUM? and if so, which one?
If you make your question sufficiently specific, then the answer may be provided, and it may be “yes” or “not likely in the near future” or “unknown”. But if you don’t make your question precise, then the answer depends completely on how I choose to interpret your question — which is probably not what you wanted.
For example, in certain (not very credible) vacua of string theory, people have already made predictions, which have been tested, and those vacua have been ruled out by data… see Figure 1 of http://arxiv.org/pdf/1010.0203.pdf .
The biggest problem in this whole business — and that is why I am writing these posts — is that no one who is prominent in the public string debate (Greene, Woit, etc.) is sufficiently precise about what they are talking about. And that’s confusing all non-experts, and some experts too.
I have been following the extremes and the mainstreams on this subject for a very long time. Your exposition here is outstanding. You answer the questions put to you. In short you are to be applauded .
I am somewhat of a “frayed knot” at my age. So if if I have it tangled excuse me.
I do hope I read correctly that you seem pretty convinced – as reflected in answer to M. Rally – that the “experts” are imprecise in how they explain this to the non-experts.
If so, I would only add that experts- at least some – benefit tangibly and Intangibly by their positions, it is hard for some to separate themselves from their vested interests. Thanks for staying above it.
Your last paragraph is so true 🙂 Points to Matt.
Great post!
Matt, I hope that at some point in this series of posts, you could describe how String Theories and Super Symmetric Theories are related.
That’s easy; they’re separate issues.
You can have supersymmetric field theories that have nothing to do with string theory.
You can (in principle) have string theories that have no supersymmetry. However, it can be hard to prove things about them — in particular, whether such theories can really be stable, and thus really describe any long-lived universes.
The only string theories that we are *fully confident* make sense for describing an interesting universe have some sort of supersymmetry built in, at least in some sort of approximate way. However, even in such theories, there is no guarantee that this supersymmetry has any near-term experimental consequences. In particular, you may not see any sign of it until you begin to produce the very heavy particles indicated in Figure 4, and even then it may be far, far from obvious.
Hi Matt,
It’s interesting to note that if we forget about string theory, extra dimensions, branes, and even gravity, and consider just Yang-Mills theory in regular old 3+1 dimensions souped up with some (N=4) supersymmetry, then, as you well know, it turns out the Hilbert space is equivalent to that of a 10D string theory. So in some cases the two ideas are connected, although the usual N=1 supersymmetry we’re looking for in accelerators may really be unrelated to string theory.
Matt: I seem to recall you depicting a quantum field as a lattice. Then when you say a photon is a ripple in the field, I can envisage a distorted lattice. No problem there. But nowhere have I seen anything to the effect that “the lattice is made out of string”. String theory seems to be a whole different animal. Can you comment on that, and can you say what the strings in string theory are made of?
A quantum field is not to be depected as a lattice; you’re confused. When people do computer simulations of quantum fields, they make *space* into a lattice, not the fields. If you’re envisioning a distorted lattice, you’re actually envisioning a distortion of space itself — a gravitational wave, whose quantum is a graviton, not a photon.
Instead, you should view a quantum field in these computer simulation in the following way. A nice example of a (non-elementary) field is the density of air. We can measure the density of air at each point in the room and figure out how it will change over time. If we want to simulate this on a computer, however, we might set up a grid of points in the room, one centimeter apart in each of the vertical and two horizontal directions. Then the field becomes the density of the air in the vicinity of each grid point. The density can still have any value, but we only know what is on a spatial grid. And a wave in the density will involve the density increasing and then decreasing at each grid point, but displaced in time from one grid point to the next. The density is changing, not the grid.
String fields are somewhat of a different animal, but for a lot of reasons… one of them being that quantum gravity is included and so space itself is part of the story, rather than being a spectator, as it is for a field theory that does not include gravity. No one knows how to do computer simulations of string fields using a similar approach to the lattice approach to simulating ordinary fields.
It is my understanding that the lattice in the computer simulation is a consequence of the numerical method chosen for the simulation.
If that is so, in itself it has nothing to do with the physics model that is the subject of the simulation.
Kind regards, GEN
Thanks for all that Matt. Yes I was envisioning a distortion of space, but for an electromagnetic wave. A photon is associated with displacement current. Displacement is associated with density variation as per a sound wave. And field is the derivative of potential so integrate your sine wave to a hump going through a lattice. (There was some CERN video like this, but I can’t find it any more). A horizontal lattice line is like a guitar string. Your pluck is like Planck’s constant. Action is associated with reaction. Any cell that’s skewed is a spin1 virtual photon. Any cell that’s flattened is a spin2 virtual graviton. Skewed squares are flattened. A string vibration on a closed path is a fermion. And so on. It doesn’t sound like QFT or string theory, but to me it sounds like their child. To you it doubtless sounds like an ass, so no reply necessary.
John, your statement that “field is the derivative of potential” is not generally true. You are probably thinking of the electromagnetic field strength (the “E” and “B” fields) that can indeed be expressed in terms of the derivatives of the vector potential “A”. Interestingly enough, in quantum electrodynamics it is “A” itself that serves as the field to be quantized, the quanta being called photons. The field “A” has only indirect physical significance and quantum mechanics is subtle, so it is extremely difficult to build an intuition for the “nature” of a photon. (I don’t know if the experts succeed in this – I for sure have not.) That’s why it is so important to cling to the equations. Any simple intuitions, especially mechanical ones, are quite certainly wrong.
I think you will find that Mr. Duffield is interested in his own opinions more than in the facts.