The Higgs field: so important it merited an entire experimental facility, the Large Hadron Collider, dedicated to understanding it. This mysterious field is on average non-zero, suffusing the universe almost like an invisible fluid, affecting the masses of the known elementary particles. What if it were zero on average instead? What would the world be like then?
Fig. 2 from previous article:The particles, with the interactions among the known particles shown as lines. The non-zero Higgs field (green swath) makes the known particles massive, and the Higgs field and Higgs particle have stronger interactions with the heavier particles.
Well, it would be lethal for us — atoms wouldn’t exist —- but in a certain sense, it would look much simpler, and much better organized. Let’s see how.
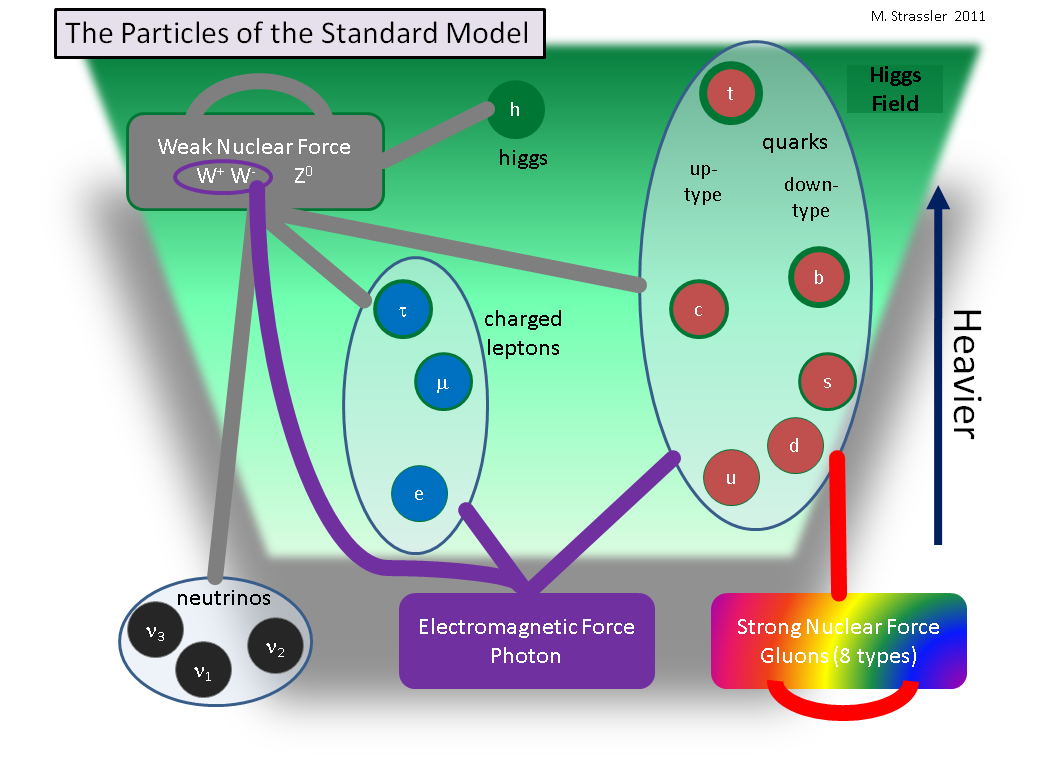
Take Fig. 2 from this earlier article about the known particles [which I recommend you read first, if you haven't already.] This figure shows the known elementary particles of nature (plus the conjectured Higgs of the Standard Model) and the lines indicate which particles directly affect one another. You see three of the four known forces of nature (gravity is left off to avoid clutter): the strong nuclear force (with gluons as force carriers), electromagnetism (whose force carrier is the photon) and the weak nuclear force (with W’s and Z as force carriers). And you see that the neutrinos, charged leptons and quarks do not interact directly with one another; they are directly affected only by the force carriers. And finally, the Higgs field, which is non-zero in our universe [you might want to look at these video clips for more info], and is indicated by the green swath, affects all of the known massive elementary particles, and in fact is responsible for making them massive.
Fig.3 : If the Higgs field were zero, the matter fields would be rearranged, as would be the forces and force carriers. None of the known particles would be massive, though the Higgs particles (of which there would be four, at least) would be massive.
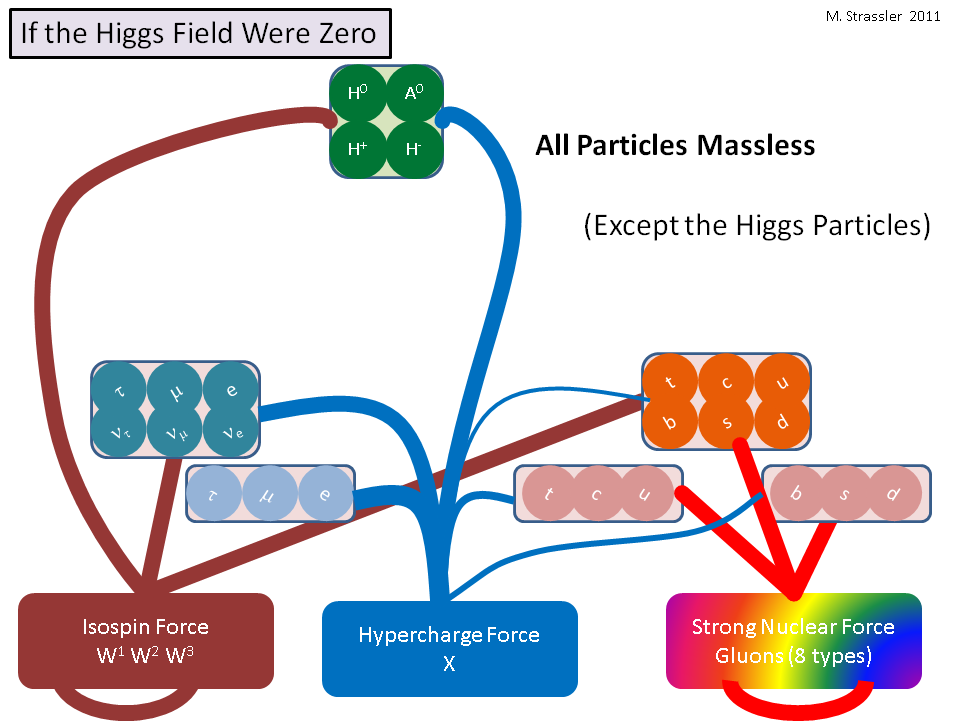
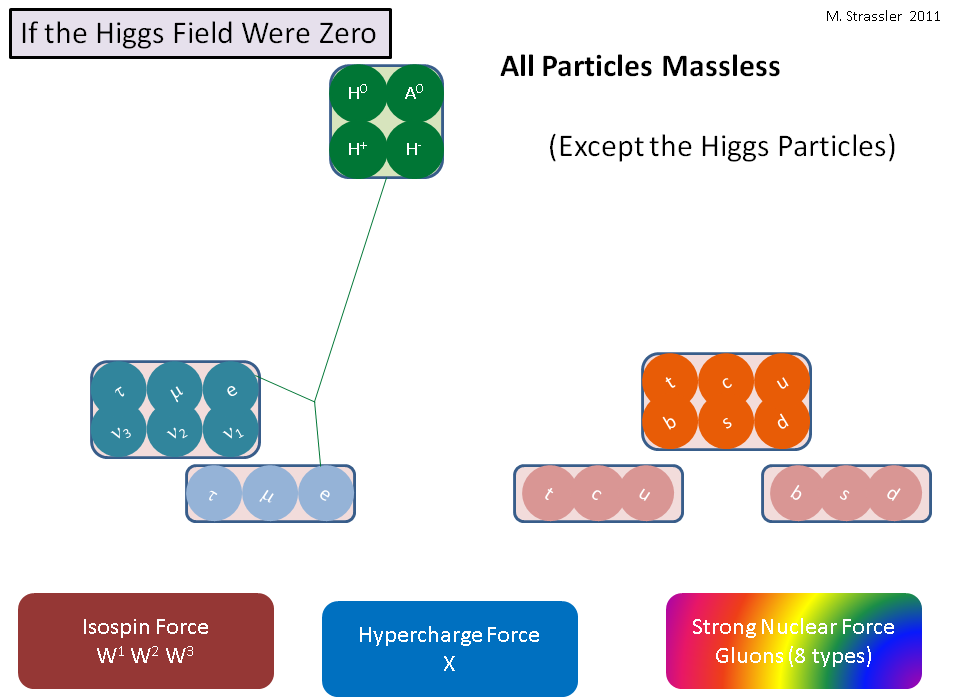
Now compare this with Fig. 3, which shows what the world of particles would be like if the Higgs field were zero. Look closely. You’ll see many differences! [In a little while we'll see where some of them come from.]
- Instead of the electromagnetic and weak nuclear force present in our world, with its non-zero-Higgs-field, a zero-Higgs-field world has these forces scrambled and rearranged. The rearranged forces are called hypercharge and isospin (for historical reasons; the names are just that, names, without other significance.)
- As part of this scrambling, the force carrier particles are changed; there are 3 W particles and an X particle, and the Z0 and photon are missing. And the W and X particles are all massless now.
- The force carriers are now simpler in another sense. The photon affects the W+ and W- particles directly; you can see that in Fig. 2, where they are connected by a purple line. But the X particle does not directly affect any of the three W particles. The gluons affect themselves as before (the red curved line); the W’s affect themselves too; but the X particle affects no force carriers at all.
- For every matter particle (except neutrinos) there are now two particles with the same name. But they’re different, as different as Arnold Palmer and Arnold Schwarzenegger. Physicists (for complicated reasons) have several naming schemes for them, but a top quark by any name would smell as sweet — so for current purposes I’ve indicated them as different by rotating one to the left and one to the right. We can call them top-left and top-right. [This happens in fact to be one of the naming schemes physicists use and it does have a bit of an underlying meaning, but it's best to just view these as names.]
- Notice that the left-particles all come in pairs, one pair for each generation (a term defined here), and are affected by the isospin force. The electron comes with the neutrino-e (or “electron-neutrino”), the up quark comes with the down-quark, etc.
- But the right-particles come singly, one for each generation, and are not affected by the isospin force.
- There are only neutrino-lefts; there are no neutrino-rights.
- In Figure 1, I used the labeling neutrino-1, neutrino-2 and neutrino-3 for the neutrinos, but in Figure 2 I use labels that correspond to the names “electron-neutrino”, “muon-neutrino”, and “tau-neutrino”. This is a subtlety you can ignore unless you are really interested in it, in which case you should read this article.
- All of the particles shown are massless — except for the Higgs particles, of which there are four! (That’s a minimum; the Standard Model, in which one assumes the simplest possible Higgs fields, has four, but the full story could be more complicated.)
How did the non-zero Higgs field alter this simpler and better-organized (but uninhabitable) world and turn into the complicated one that we live in (Fig. 2)? It all has to do with how the Higgs field interacts with the isospin and hypercharge force carriers and with the matter particles. How this works for the top quark, as an example, is sketched in Figs. 4 and 5. The top-left quark and the top-right quark interact strongly with each other and the Higgs particle… and not with other matter particles. In particular, if a top-left quark encounters a Higgs particle, it can turn, with high probability, into a top-right quark. Once the Higgs field is non-zero, this type of interaction causes the two versions of the massless top quark to become a single massive top quark, with a large mass.
Fig. 4: The top-left and top-right massless quarks interact strongly with the Higgs field; when the Higgs field becomes non-zero, a very massive top quark results.
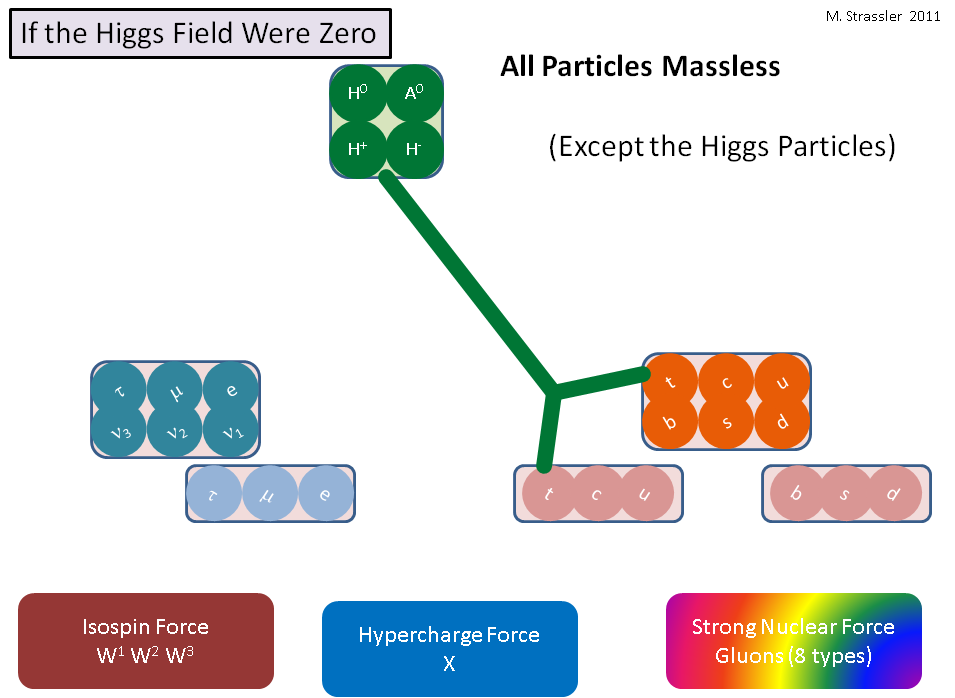
This linking of the top-left and the top-right is not to be confused with the binding of two particles into a composite object, as a proton and an electron are bound together by the electromagnetic force to form a hydrogen atom. It is a different kind of combining, in which two elementary particles are mixed together into a single elementary particle.
Fig. 5: (a) The top-left is massless and travels at the speed of light. (b) Should it bang into a Higgs particle, it can easily turn into a top-right. (c) With the Higgs field non-zero, the top-left and top-right continually are flipped back and forth, which causes them to travel below the speed of light, and gain a lot of intrinsic energy. (d) It is convenient to think of this extra intrinsic energy as the mass-energy of a very massive top quark, and view this peripatetic flip-flopping of top-left and top-right as the normal state of this top quark.
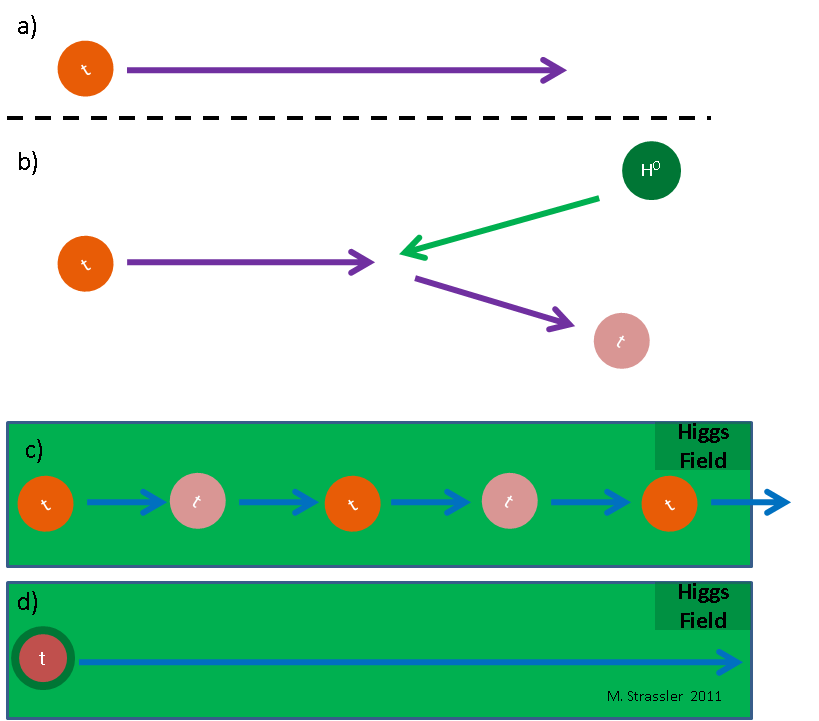
How does this work? Fig. 5 shows a sketch. When the Higgs field is not zero, a top-left particle would travel at the speed of light, alone. The same would be true of a top-right. But when the Higgs field is not zero, its presence, and the fact that it has a direct interaction with the top-left and the top-right, forces the top-left to convert over to a top-right, and back again. How often does this happen? About a 100 trillion trillion (100,000,000,000,000,000,000,000,000) times a second. This conversion process makes it impossible for us to think of the top-left and the top-right as separate particles, because they are inextricably linked together; if you have one, you will very soon have the other. (You never have both at the same time, which is why the top quark remains elementary, not composite.) We call this mixture of these two particles the top quark, collectively. And the non-zero Higgs field, whose presence causes the flipping back and forth between top-left and top-right, endows this mixture with an additional intrinsic energy, even when it is sitting still. That intrinsic energy is indistinguishable from mass-energy (E=m c-squared energy); it behaves the same way in experiments. In other words, what we call the mass-energy of the top quark is really, if you prefer, the energy it picks up when sitting in a non-zero Higgs field. Take the Higgs field away — make it zero — and the top quark goes back to being two separate massless particles, the top-right and top-left.
The same phenomenon makes the electron massive too, but the interaction of the Higgs field with the electron-left and the electron-right is very weak, and so the electron, in the presence of a non-zero Higgs field, is massive but still relatively lightweight. The flip-flopping between electron-left and electron-right happens only .000003 times as frequently as for the top-left and top-right, and it follows (from a little bit of mathematics) that the electron mass is .000003 the mass of the top quark.
Fig. 6: As in Fig. 4, the massless electron-left and electron-right become a massive electron when the Higgs field becomes non-zero, but because their interaction with the Higgs is feeble, the electron remains lightweight.
All the other quarks and charged leptons get their masses in a similar way. The stronger the interaction of the left- and right- object with the Higgs, the larger the mass that results for the mixed object when the Higgs field is non-zero.
What about the force carriers? The Higgs does not affect the gluons, but it rearranges the isospin and hypercharge forces, making the photon out of a mixture of the W3 and X, the Z0 out of a different mixture of the W3 and X and the Higgs particle called the A0, and the W+ and W- out of mixtures of the W1, W2, the H+ and H-. [Warning: very small white lie here, a technicality important mainly for experts; will fill this in in a supplementary post.] This process, called the Higgs Mechanism, makes the W+, W-, and Z0 massive, leaving the photon massless.
Aha!! So that’s how a non-zero-Higgs-field world ends up with 1 Higgs particle (the h) whereas a zero-Higgs-field world has 4, the H+ and H-, A0 and H0. Similarly to the mixing of top-left and top-right to make a massive top quark, the three extra Higgs particles end up mixed with the three mixtures of the massless W’s and X particles to make the massive Z0, W+ and W-!
Fig. 7: The non-zero Higgs field mixes the massless W and X force carriers and the Higgs particles, making three of the force carriers massive, leaving the fourth massless, and retaining one massive Higgs particle from the initial four. This is what happens in the Standard Model; the number of Higgs particles in nature may be different from shown. But the core idea --- that three Higgs fields combine with the massless W's and X to form the massive W's and Z --- is already well-established, using existing data from the past three decades.
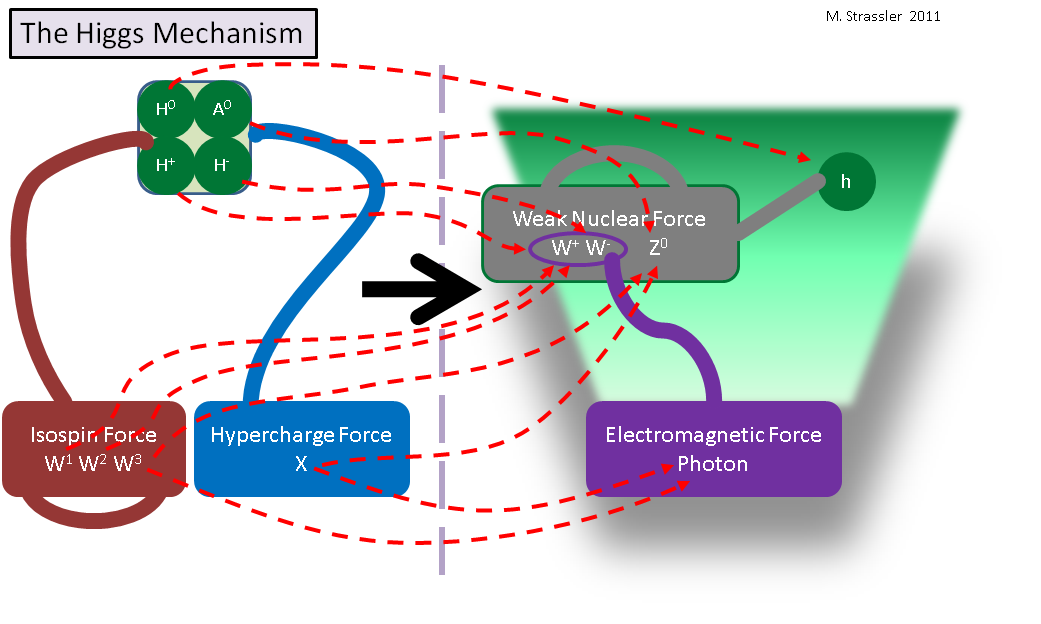
A force whose force carrier is massive turns out to be ineffective at long distances, and this is why the weak nuclear forces seem so weak to us. If the Higgs field were zero, the isospin and hypercharge forces would all be comparably strong. Instead, our world has a rather strong electromagnetic force with a massless photon as carrier, and a weak nuclear force that is so weak that our daily lives are little affected by it — although admittedly it is essential in the internal furnace of stars, including the sun!
The reason that the world looks so complicated, with all those particles with wildly different masses, is partly that the Higgs field and Higgs particle interact with the different matter particles with such different strengths. The problem of diverse particle masses thus is actually a problem of their diverse interaction-strengths with the Higgs field/particle. Why are these interaction strengths so very different? There is no consensus as to the answer to this question (which particle physicists call the “flavor problem” — referring to electrons, muons and taus as different charged-lepton flavors, and similarly for quark flavors). We hope the Large Hadron Collider might give us some insights here, but there is no guarantee that it will.
A loose end — how did the neutrinos get their masses?! The answer is that we’re not entirely sure. One possibility is that there are in fact neutrino-right particles in nature — these would be very difficult to produce in experiments because they are not affected by any of the three forces shown in Figs. 2 and 3 — and the mechanism for giving neutrinos mass is the same as for the other particles. A second possibility is that neutrino-left particles get their masses from an indirect effect involving the Higgs particle — an effect which is not an option for the other particles. This second option is favored by many of my colleagues because it naturally would explain why the neutrinos are all so much lighter than the quarks and charged leptons. But this is a long story…
I’ll end with an important point, one which I hope you’ll find interesting. Many people (including aspiring physicists) assume, when they first encounter the story of the Higgs field, that it must be connected with gravity, which also engages heavier particles more strongly than lighter ones. Gravity pulls on top quarks harder than it pulls on electrons, and so do Higgs forces. But experienced physicists reject this notion. Why?
The point is that for gravity there are no exceptions — gravity always pulls on particles proportional to their masses. (Actually that’s itself a misconception; gravity pulls on things proportional to their energies. In daily life, any object’s energy is dominated by its mass-energy, E = m c-squared, so for rocks, people and stars, energy and mass are in almost exact proportion to one another. But gravity also bends starlight! If gravity only pulled on mass, then it wouldn’t pull on light, which consists of massless photons.)
By contrast, only those particles that get their masses from the Higgs field have a relationship between their masses and the strength of their interactions with the Higgs. In particular, as you can see in Figs. 3 and 7, the Higgs particle itself does not get all of its mass from the non-zero Higgs field — and the strength of its interaction with itself is not directly related to its mass. [There is a correlation, but not proportionality.] This is not unusual. In other articles on this site, you will see many other examples of conjectured particles, such as those that arise in the speculative theories known as supersymmetry and as warped extra-dimensions, that get their masses in other ways.
So the link between gravity and energy (and thereby mass, in daily life) is absolute, while the link between the Higgs and mass is likely to be true only for the known elementary particles, and may not be true for other elementary particles yet to be discovered — and is not even true for the Higgs particle itself.
In other words, any resemblance between the Higgs field and gravity is purely coincidental!
8/10/11
Hello Matt, nice blog you have going here.
I put together a similar periodic table of the standard model (and gravity) recently — thought you might like it:
http://is.gd/I8IaBN
The “hypercharge force” B there is your X, and its interaction lines roughly indicate that Y is a combination of W’³ and B-L.
Garrett — your table is elegant, but to avoid confusing the public you might want to indicate somehow that the existence of the neutrino-right particles is speculative. Normally we do not consider such hypothetical particles part of the Standard Model — they would be considered (by convention only) Beyond the Standard Model. The only hypothetical particle in the Standard Model, as most physicists refer to it, is the Higgs particle.
Does the QCD condensate contribute strong scale masses (of order the pion decay constant) to the Ws and Z or is it entirely the Higgs?
Again, this is way too advanced for this article. The answer, however, is neither yes nor no. Effects of the strong nuclear force do indeed generate a Higgs-field-like effect, and so the statement that the W and Z particles would be massless in a zero-Higgs-field world is a small white lie. (Thanks for reminding me that I should say that somewhere!) However, you have to recalculate everything carefully — and you would find, after recalculating, that the strong nuclear force would be weaker than it is in our world, so the effect would be much smaller in such a world than it is in this one.
More generally, when you have two Higgs-like fields —- in the current example, the Higgs field itself and this “QCD condensate” that you refer to — then they must be combined not linearly but in quadrature: the mass of the W is the square root of the sum of the squares of the two Higgs fields. That means that the effect of the QCD condensate — this second Higgs-like field — on the W and Z masses is about one part in a million — dwarfed by other effects, and far too small to measure.
Thanks.
Good article! In the 0 Higgs field world what is the property that is different between the left particles and right particles? For example what has to be changed by the Higgs interaction to turn an electron-left into an electron-right?
The main difference is the one you see in the figure: the electron-left and neutrino-1-left feel the weak-nuclear force while the electron-right does not. Also, though I didn’t emphasize it, the electron-left and electron-right have different hypercharge — even though they have the same electric charge after the Higgs field is non-zero. When you ask, “what has to be changed by the Higgs interaction” — that’s not really the right question, I think. The Higgs field rearranges pretty much everything all at once to make the world the way it is.
I’m not really sure I’ve satisfactorily answered your question. Feel free to re-ask it.
Based on a little Google research, I think the answer to what is different between the electron-left and electron-right is chirality. Please let me know if that is correct or not. After a little thinking, I can see that the whole concept of the Higgs field is extremely important. If not for the Higgs field giving them effective mass, particles such as electrons, quarks, and whatnot would be zipping around at the speed of light. Due to relativistic effects, these particles would not experience time passage and space passage. So it seems we experience time and space itself because of the Higgs interactions. Am I close on this assumption?
You are correct. The only tricky bit with the term chirality is that it is not helicity (which you can measure physically). I have to emphasize to my particle physics students that helicity is the thing that you colloquially might call chirality as you use it, say, for molecules. Chirality turns out to be something more mathematically subtle. For massless particles chirality and helicity are the same, but not for massive ones. So be careful putting too much weight on the term.
Oh, and about us experiencing time and space because of the Higgs — not really wrong, but on the other hand if the Higgs field’s average value were zero, and electrons were massless, there would be no atoms, and probably little structure of any kind in the universe, so we would have some other problems experiencing space and time!
Pingback: LHC lässt dem Higgs immer weniger Verstecke « Skyweek Zwei Punkt Null
Thanks for a nice clear explanation of how the Higgs mechanism works. That’s the first time I’ve seen it explained without lots of handwaving and very soft analogies!
Could you say a little more about how/why the interaction with the Higgs “endows this mixture [of top-left and top-right] with an additional intrinsic energy”? Is this something special to the Higgs mechanism, or just a general fact that interactions endow particles with energy?
Hmm… I am not sure I can do it without a whole section on how mass and energy work in quantum field theory. It’s not so easy to explain without equations — though the required equations are simple. I do intend to write an article on mass and energy, and I’ll try to fill in this pedagogical hole.
A mass energy article would be very useful. Am I correct in suspecting that mass of a volume of space is nothing more than energy confined within that specific volume? In other words, mass as a separate entity from energy doesn’t exist. It is the same thing.
I have one planned and half-sketched. Mass and energy are very different things, in fact, and it is not true that the mass of a volume of space is simply the energy within that volume. But the subject is tricky enough, and important enough, that I’m trying to make sure I address all of the important subtleties properly before putting the article up. Stay tuned; it will appear.
Excellent article. Just having one question,
What points of this article must be rewritten if the Higgs particle (both 1 and 4) is ruled out (that is, no interaction with the Higgs)?
Your question could mean two things. Do you mean to ask about how the article would change if the Standard Model version of the Higgs particle is ruled out? If so, the answer is that the Higgs sector may be somewhat more complicated, with more Higgs particles, or perhaps a whole new set of particles and forces. The truth will be determined from experimental data. The details of the Higgs sector will change, the basic structure of the relation between the Higgs and the matter fields may not change that much.
Thanks for the quick reply. My question is “if there is no Higgs of any kind”, that is, no Higgs to interact with at all in all cases. This might be a hypothetical question. Yet, can it be addressed theoretically?
Again your question could mean two things. Do you mean: no Higgs particle of any kind”? Or do you mean “no Higgs field of any kind”? These are quite different, as I’ve emphasized in the Higgs articles http://profmattstrassler.com/articles-and-posts/the-higgs-particle/360-2/ and http://profmattstrassler.com/articles-and-posts/the-higgs-particle/implications-of-higgs-searches-as-of-92011/ .
I truly enjoy your posts. In addition to being a physicist, you are a good linguist. It is super important on the nitty-gritty of the semantic meaning of each term.
My true question is about Higgs mechanism.
For Standard Model, Higgs mechanism is the foundation for making SM meaningful by providing a mechanism to give particle its mass. Indeed, for any model, it needs a Higgs-like mechanism to provide the Higgs-effect (providing mass for particles). Thus, as long as the Higgs-effect is produced, can a whatnot mechanism be just as good as the Higgs mechanism?
In your article, the Higgs mechanism gives a massless hand-particle a mass by a Higgs-ball bouncing between the Lift and Right hands in the Higgs field.
I do know another mechanism which gives a massless foot-particle a mass. In the space-time sheet, there is a “step”, and this massless foot-particle is jumping up-and-down and is jumping down-and-up endlessly at this “step”. This process will give this foot-particle a mass too.
While the hand-particle bouncing a Higgs ball, the foot-particle is, in fact, bouncing the entire space-time sheet under its feet. They are identical mechanism while playing (bouncing) different balls. Of course, they will be different physics.
Thus, my true question is “Can there another mechanism be more “real” than the Higgs mechanism?”
Yes, being very careful with both one’s thoughts and one’s means of expressing them is essential to being a good physicist.
One has to remember that the Standard Model of particle physics, with the one exception of the Higgs particle itself, is very well-tested. The structure of the theory is very well known, and any major changes to the mass-generation mechanism for W particles and top quarks (and to a lesser degree the lighter-weight particles) risks screwing up the Standard Model’s many successful predictions. There are several mechanisms for mass-generation known in particle physics, but only a sub-class of them, all of similar type, would be consistent with existing data. And in particular, I think the Higgs mechanism for generating the masses of the W,Z and top quarks has passed enough experimental tests that it is very unlikely to be dislodged. But this refers only to the claim that some kind of Higgs field with some particular general properties has a non-zero value, and not to any of the nitty-gritty details of the Higgs field, or its particles (if any).
Pingback: Standard Model Tutorials for the Masses (…er, sorry about the pun…) « Whiskey…Tango…Foxtrot?
Pingback: The Higgs Boson: Backed Into A Corner « Whiskey…Tango…Foxtrot?
Thank goodness! I work at a science museum, and I expect any time now to have to answer questions to the general public about the Higgs. I’ve looked and looked and looked for a non-mathematical description that didn’t use the “movie star” analogy or something equally weak. Finally, here is something I can both understand and sink my teeth into. Of course, it’s still way too much for a general public that still doesn’t know what an electron is. But at least now I can have a little bit more confidence that I understand it at a higher level than the movie star analogy. Thank you. I’ll be back to study this more fully.
Thanks for your positive feedback. Let me know what you don’t understand, I’m always working to improve the site.
Oh, I will. I hope you don’t get sick of me. If it helps, remember that you’re not just helping me. Think of me as a “middle man” trying to educate people even less knowledgeable than myself about why the big headlines in the newspaper matter.
First question (and I apologize that you’ve started to answer this in some comments above): your description of how the top quark gains mass through collisions with Higgs particles makes me wonder why the mass doesn’t go up as speed increases (of course I know it does in special relativity, but you’re not saying that this is the mechanism of increasing mass in SR, are you? Sorry, that’s two questions). Also, why does the stationary top quark have mass at all? How is it that a stationary top quark collides with Higgs particles? OK, that’s three questions.
Next question (and again I apologize if you’ve discussed this elsewhere; I’ve only just discovered your site): all this discussion of how the Higgs mechanism gives mass to the particles that make up the world seems very separated from the search for the Higgs particle. Can you give me a simple, layman’s explanation of how smashing protons together produces a particle that is representative of a field that gives those protons mass? Remembering that for most people if they have to take more than three steps, they’re lost?
I’m sure I’ll have lots more questions after these, but I’d love to start here.
Thanks,
Steve Whitt
For some reason my last post didn’t appear. Sorry if it does and this is a repeat.
1) Are you saying that the top quark in your example is in some sense colliding with Higgs bosons, causing it to flip from top-left to top-right?
2) If so, what causes the collisions for a stationary top quark? Are the Higgs particles in motion?
3) I’m pretty sure you’re not saying that this is in any way linked to relativistic mass increase. So why does a slow-moving top quark (which, I would think, will collide with more Higgs particles) have essentially the same mass as a stationary one?
4) Can you explain (or point me to another place on your site) how smashing two protons together can form a Higgs boson. Then, please explain how and why the Higgs decays to the other particles that we observe?
Thanks,
Steve Whitt
Steve — these are good questions and deserve careful answers. As you can imagine I am a bit swamped with Higgs-related things just now — if I forget to reply within the next week, feel free to bug me.
Thanks. I’ll try to be patient. But as I said, yours is the first explanation I’ve found that I think I can actually use here at the science museum. You’re my lifeline!
Hi again,
I’ve been studying your site much more closely and I think I’ve found a good answer to the last question in your article on photon-photon detection. Very interesting and well-written. I have another question about that, but it can wait.
I know you’re quite busy, but time is of the essence for me, too, if I’m to be able to explain these things to the general public. I have a thought about explaining the Higgs mechanism to laymen, and I was hoping you (or any other physicists reading this) could critique it.
I think of photons as disturbances in the electromagnetic field. A good analogy might be the running lights you see on theater marquees. The lights are just flashing on and off, but the particular sequence makes it seem as if a physical object is moving around the sign. Photons are like that, in that they are a disturbance in the electric and magnetic field at a location in space, but that location is always changing in a particular direction at the speed of light.
OK so far, or have I botched this?
Photons aren’t affected by the Higgs mechanism, but things like electrons and quarks are. If they weren’t, they would move much like photons, disturbing their own fields (electron fields, up quark fields, etc.) at the speed of light in a particular direction. But because the Higgs field essentially weighs the matter particles down, it keeps them from propagating their fields in the way Higgs-free photons can. In my mind’s eye I see it as the Higgs reaching up and grabbing the electron (or other matter) wave. saying, “Not so fast!” We experience this weighing down as mass.
(If they then ask, why does the Higgs field grab matter particles like that, I then have the description you gave me of the flipping of left- and right-particles back and forth many times a second. This flipping endows the particles with energy, which through E=mc^2 we measure as mass.)
I doubt that this will be a particularly useful explanation, as my sense is that it will be difficult to convince an inexperienced audience that all their particles are actually just disturbances in a field. But I wanted to know if this is at least a valid way of thinking about particles and the Higgs. I’ve never seen this analogy used, so I don’t want to use it if you or other physicists think it’s flawed. As a teacher, it’s always good to be able to explain things from different approaches.
Thanks for your help,
Steve Whitt
This bit confuses me: “When the Higgs field is not zero, a top-left particle would travel at the speed of light, alone.”
How can two flip-flopping particles, each traveling at the speed of light through the Higgs field, “average out” into a particle going less than the speed of light? Do they slow down as they oscillate into each other?
How do you even get a top-left particle “alone” in a non-zero Higgs field? Wouldn’t it mix up with a top-right?
Your comment about the Higgs effect being separate from gravity is somewhat mind-blowing. Do we know for sure then that the Higgs “mass” is the same as the gravity “mass” for massive (not-massless) particles? As I understand it, we believe the gravitational mass is the same as the inertial mass — but don’t really know why — and now it seems there’s another definition of mass?
Well, in the equations the Higgs effect has always been quite separate from gravity; I don’t know of any successful theory that had a way of tying them closely together, or any principle that would suggest they are related. Again, there may be many elementary particles in nature (including dark matter particles) that get little or none of their mass from the Higgs field. Gravity, on the other hand, pulls on all energy and momentum, including the energy stored in mass-energy (or “rest-energy” as it is often called.)
The mass provided by the Higgs is a contribution to the inertial mass of the corresponding particle.
Gravity, of course, does not provide mass; it just pulls on it… but …
While Einstein’s theory of gravity implies that gravitational mass is the same as inertial mass, it also implies that gravity is more complex than in Newton’s gravitational force law, and pulls in a complicated way on energy and momentum.
So the fact that many particle masses come from the Higgs field does not complicate the notion of mass. Mass is pretty simple; though it can get contributions from multiple places, they just add up. Gravity, after Einstein, is the complicated part.
The question of whether gravitational mass = inertial mass has been tested to high accuracy for ordinary matter. I am not sure what the limits are for protons, neutrons and electrons separately.
What an elegant way to describe the Higgs mechanism. Thanks for this site. I have a quick question. You mention the mass provided by the Higgs is a contribution to the inertial mass. Where does graviton fit in this picture? Also, in a zero Higgs universe, would there be a gravity affecting massless W’s or quarks as it affects photons in our universe? Thanks for your time.
Gravity is quite separate — it Einstein’s theory, it interacts with energy and momentum, and (almost incidentally) it interacts with mass because all mass has its associated E= mc2 “rest energy” (or mass-energy as I tend to call it on this site.) So yes, in a zero-Higgs universe, gravity (and the associated graviton particle that it should have, in our quantum world) will treat photons, W and Z particles the way it treats all massless particles.
Can you say more about the difference between chirality and helicity? I’ve always been confused why a neutrino-left does not become a neutrino-right with a lorentz boost ( assuming neutrinos have a small mass ).
Not in a few words, and it’s too advanced for this part of the website. This is the sort of technical question I’d prefer to discuss in the Technical Zone.
How come in Figure 3 you have not shown the direct interaction between the Higgs fields and the leptons and quarks? Thanks.
Just because it would have made the picture a lot more complicated-looking. See Figure 4; imagine if I had put all the interactions on top of one another. I don’t think you could have read the figure at all.
Someone on another forum asked how the Higgs particle acquired mass? That was a good question. I remembered this article and linked to it, but I got a detail wrong. You stated that the Higgs particle doesn’t get its mass from the Higgs field (there’s a correlation, but it’s indirect). How does the Higgs particle acquire mass, please?
We don’t really know yet. We just know that it would be massive even if the Higgs field’s value were zero… and so not all of its mass can come from the Higgs field itself. But we don’t understand enough about the Higgs field yet to give a confident answer.
I’m somewhat confused about why the photon ends up massless. Does the Higgs Field interact with isospin and hypercharge force carriers differently than it does with matter particles? More specifically:
You demonstrate that the process of the non-zero Higgs Field rapidly and repeatedly converting a top-left into a top-right and back again results in the (composite) top quark as we know it and gives it mass-energy; and you say that a photon is ALSO the result of the Higgs Field interacting with (and somehow combining) two particles (W3 and X).
Are these the same processes? In other words, is a photon the result of the Higgs Field rapidly and repeatedly converting a W3 into an X and back again? Or is the mechanism somehow different with force carriers? (And if the mechanism is somehow different with force carriers, how do the weak force carriers get their mass?)
I understand that the (massless) photon differs from the (massive) weak force carriers in that the latter are composites that *include* Higgs particles while the former is a composite only of W3 and X, but if the Higgs Mechanism gives mass-energy to matter particles that are Higgs-less composites, why does it not do so in the case of photons?
A related question, which might really be the same question phrased yet another way: given a non-zero Higgs Field, I gather from your article that the energy involved in interactions between top-lefts and top-rights is somehow “stored” in the composite top quark as mass-energy. If similar interactions between W3 and X particles result in a photon with no mass-energy, what happens to the energy involved in the W3–X interactions?
Thanks,
Mike
At some point we’re getting to the edge of what we can do without some math. I don’t think I have a simple answer for you that doesn’t involve actually checking simple but necessary details of equations. These things are not obvious. For example, a different type of Higgs field can leave both the photon and the Z massless, while other combinations of Higgs fields can leave nothing massless.
But I do want to emphasize the difference between composites and mixtures. Don’t confuse them! In fact, I said this in this very post, next to Figure 4:
This linking of the top-left and the top-right is not to be confused with the binding of two particles into a composite object, as a proton and an electron are bound together by the electromagnetic force to form a hydrogen atom. It is a different kind of combining, in which two elementary particles are mixed together into a single elementary particle.
The W is a mixture of a massless W and a part of the original Higgs field; the top quark is a mixture of top-left and top-right. They are still point-like objects even though they are mixtures of point-like objects. The proton, by contrast, is a composite object, made from a number of point-like objects. It is not point-like; it has a size that you can measure. And you can make it vibrate; the excited states of the proton, like excited states of an atom, exist because the proton has a size.
(Of course, when I call something point-like I can’t prove to you that it’s not composite. I can only prove to you that it has a size that is smaller than anything we can measure today — that for all practical purposes it behaves as if it is pointlike. 80 years ago we thought of the proton as pointlike, because we couldn’t yet detect its size.)
Yes, sorry, my word choice of “composite” here was poor, and I wouldn’t want to confuse any other readers. I should have used the word “mixture.”
But I myself wasn’t actually confused about the point-like “mixture” of top-left and top-right that makes a top quark with the “composite” nature of hadrons; I’m not sure if knowing this changes how you might answer my question: if the top-left and top-right are massless but nevertheless “mix” to form a massive top quark with a non-zero Higgs field, why do similar(?) interactions between the massless W3 and X result in a massless photon? What happens to the energy involved in that interaction if it doesn’t become mass-energy? Does this eventually boil down to the difference between the physical nature of fermions vs. that of bosons?
[Edit:]
But I myself wasn’t actually confused about the point-like “mixture” of top-left and top-right that makes a top quark ***vs.*** the “composite” nature of hadrons…
If the top quark’s energy is a summation of the top-right, top-left and the Higgs, then what is the mechanism that destroys the distinct characteristics of the three components and produces one indistinguishable “particle?
What does this got to do the whether the Higgs field exist or not? Well, if the top quark is stable once it is produced then the Higgs boson is entangled within that space for as long as the top quark’s lifetime. Yet I have not read anyway that says the top quark interacts with any other elementary particle. Should not the entangled Higgs have some interactions (even through gravity) with other particles? If the combination process is irreversible wouldn’t that contradict the SM itself?
Does the photon’s identity as a mixture of two more fundamental massless carrier particles, W3 and X, mean that the classical electromagnetic field itself can be characterized in the same way–in other words, could one write down classical field equations for the “hypercharge” and “isospin” fields, plus some classical description of the Higgs’ effect on the “hypercharge” field, and have Maxwell’s equations come out at the end?
Could one plot out an “X-Ray” of a classical electromagnetic field showing its “hypercharge” and “isospin” innards, or is the mixing too fundamentally a QFT phenomenon for that to be a meaningful idea?
The answer is yes to both questions. It is something you can largely understand in classical field theory, no quantum field theory required. I can probably explain it someday, thanks for asking.