Ok, everyone; by now you’ve all learned that the ATLAS experiment at the Large Hadron Collider [LHC] announced recently their measurement of the mass of the Higgs particle, in its decay to two photons, is at 126.6±0.3±0.7 GeV/c²; and meanwhile they measure the mass for apparently the same particle, in its decay to two lepton/anti-lepton pairs, to be 123.5±0.9+0.4-0.2 GeV/c², about 3 GeV/c² lower. “So bizarre”, wrote Michael Moyer at Scientific American, bandying about the idea that there are two Higgs-like bosons in this data (though, having pointed out the ambulance to you, and neglecting also to mention CMS’s data from November that directly disfavors this interpretation of the ATLAS data, he tells you later that some physics bloggers say you shouldn’t chase it…)
Well. How bizarre is this 2.7 standard deviation discrepancy really?
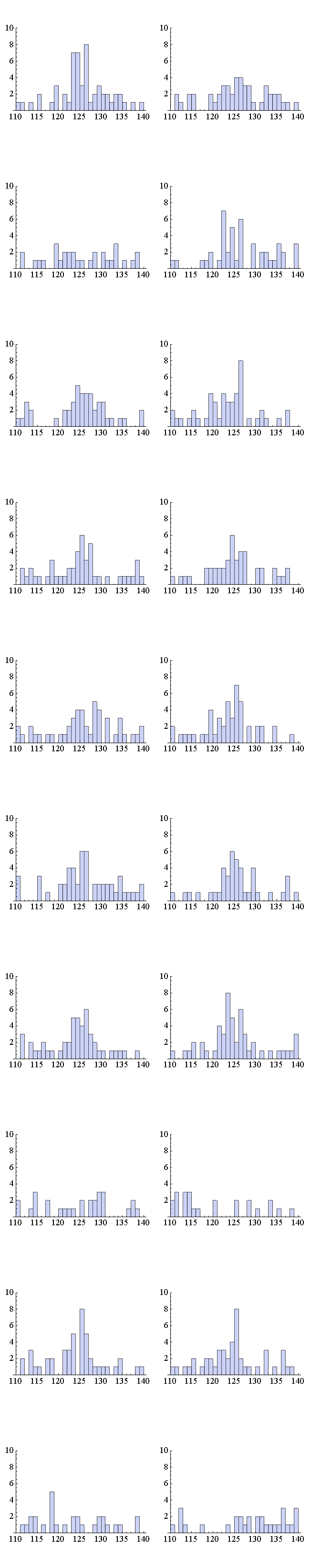
At the end of this post are 20 plots showing randomly generated data, in amounts comparable to those used in the current measurement of Higgs decaying to two lepton/anti-lepton pairs (often called “four leptons” for short). [Warning: This certainly hasn’t been done with the level of care needed to match the ATLAS measurement in any precise way; I’ll say a bit more below about the caveats.]
- In some of the plots, there’s just a random flat background of about 40 events, similar (though not identical) to what arises in the four-lepton Higgs measurement.
- In some of the plots, there’s a Higgs-like peak of about 20 events — a very sharp peak with a perfect detector, but one which is smoothed out a bit by the inevitable imperfections in a real particle detector.
- In each plot with a peak, the mass of the Higgs has been chosen to lie somewhere between 122 GeV/c² and 127 GeV/c² [not equally populated].
So:
- Can you tell which plots have a Higgs-like signal, and which ones don’t?
- In each plot where there is a signal, can you estimate the Higgs mass? Again, in each plot, it lies somewhere between 122 and 127 GeV/c². You’re not going to get it exactly right — that’s impossible — but do your best, and let’s see what happens.
A couple of additional comments to help you:
- The resolution on the measurement (i.e., the effect of imperfections in the measurement) is such that with infinite amounts of data, the peak that you’d observe would be a bump whose width, at half the bump’s maximum height, would be about 4 GeV/c².
- The bins in each plot are 1 GeV/c² wide; the bin just to the right of the number 2 in “125” runs from 125 to 126, the next from 126 to 127, and so forth.
Lastly, a caveat: in the real ATLAS or CMS measurement, the mass of the Higgs is not measured simply by fitting a Gaussian peak over a flat background. So don’t take this exercise too seriously! It’s just a useful learning experience, and nothing more. What the experiments actually do is far more sophisticated, accounting for the properties of each event separately!!
Here we go: Good Hunting! (If your browser has trouble with the figure, try clicking on it.)



7 Responses
Sorry, I can’t find it.
Completely not confident, especially by just using visual inspection.
Also not sure this is the sort of reply you were expecting but reading left to right and down the page and guessing the mass to the nearest Gev:
125
0
0
0
0
0
0
0
0
125
0
124
125
124
0
0
0
0
0
0
but each time I look again I seem to see less of a signal.
You did fine, in a sense, in that you declared defeat if you couldn’t find the mass. But there was a Higgs signal in most of the plots. The point, of course, is that one should not underestimate the challenges of working with very small numbers of events, as ATLAS and CMS are doing in their studies of the Higgs-like particle decaying to two lepton/anti-lepton pairs.
This example is quite illustrative. I would not attempt to identify the plots with a signal only by visual inspection; a serious statistical analysis, running the data through a computer program or at least a pocket calculator, seems necessary to me.
For example, the bottom-left plot seems to show a peak when inspected visually, but this peak is outside the expected mass range between 122 and 127, and also vanishes if smoothing out the plot by adding two adjacent bins into a single one.
Also, some plot may at first glance indicate that there is not a single peak but two or three of them; but when running a detailed analysis it may turn out that there is no statistically significant peak at all in them.
Even when doing detailed statistics, there are different ways to look a the data. One may test the hypothesis that there is no signal, and all the spikes are just noise; one may test the hypothesis that there is one single peak with a specified width (but this is cheating, using external information); and there are further hypotheses to test, such as for 2 or 3 peaks, or one large peak and a small one … (Here I refer to the simulated plots, not to the idea of 2 Higgs in the real LHC data.)
Certainly we need the patience to wait for a larger amount of data generated by the LHC before being able to tell details.
A bit surprising to me is that for the real LHC data, the error bars given for the Higgs mass estimates are so small; possibly there are additional, not yet detected sources of inaccurate measurements that need to be taken into account.
One reason the error bars are smaller is what I mentioned; that the methods used are more sophisticated, with certain events that are much better measured than others being weighted more heavily. But that also means that a single problematic event that is incorrectly believed to be well measured could push the result around.
In this case the error bars have a somewhat different meaning than by using simple, standard statistics on the data. Additional assumptions about the quality of each measurement enter the calculation; with the advantage of a better estimate of the mass, but at the cost of larger deviations if the assumptions made are not always valid.
If the result may depend significantly on a single event, an interesting test would be to do the same statistics again but excluding one (or two or three) events from the sample, and see how strongly the result differs. (Maybe they have done sort if this test in evaluating the measurements.)
However, even with a larger sample where individual measurements have less impact, a systematic error in the assumptions about the quality of the measurements may introduce a systematic bias.
Using “more sophisticated” methods that weigh the individual measurements may also make it more difficult to compare the results from different experiments and/or different detectors, at least if the weight calculation depends on the specific properties of the experiment/detector.
Certainly these issues are already being investigated now.