While I was busy with last week’s LHC workshop in London, a very interesting and short paper appeared, written by two of the world’s experts on violations of “Lorentz invariance”, the symmetry of space and time that underlies Einstein’s theory of special relativity. The authors, both from Boston University, are Andrew Cohen, who is highly regarded within the community for several significant contributions to high-energy physics, including his co-authorship of the key papers that led to the theory of a composite Higgs field known as “Little Higgs”, and Sheldon Glashow (formerly at Harvard), Nobel Prize winner for his work on the weak nuclear force, and famous for many other contributions of nearly equal importance. In the 1990s, Glashow, with the late great physicist Sidney Coleman, (highly respected not only for his exceptional theoretical research but also for his beautiful scientific writing and extraordinary teaching ability), wrote two important papers on violations of Lorentz invariance. It was they (to the best of my knowledge) who pointed out the enormous value of Cerenkov radiation in testing Einstein’s relativity principles. (I’ve just written a new short article on Cerenkov radiation, and there I briefly explain how Coleman and Glashow used it previously to test Lorentz invariance.)
What Cohen and Glashow did last week was to generalize this idea to point out a new physical phenomenon (new at least to me) and use it to argue that OPERA’s result is self-inconsistent. They argue that the very effect of faster-than-light travel that OPERA claims to observe would have caused distortions in its neutrino beam that clearly were not observed. Moreover, Cohen and Glashow also pointed out that at least two other experiments studying higher energy neutrinos put even stronger constraints on the possibility of anything similar to what OPERA observed.
What I find attractive about this paper is that it is simple and elegant, making use only of very well-known properties of the weak nuclear force and quantum field theory, basic conservation of energy and momentum, relations between energy and momentum and velocity for the various particles involved, and a small number of prior experimental results. It relies on very few assumptions about the nature of space and time. And it makes use of a novel (or at least poorly known) physical mechanism. Finally, it is a classic theorists’ paper, cornering the OPERA result, like a trial lawyer cleverly and swiftly tricking a thief into a self-contradiction.
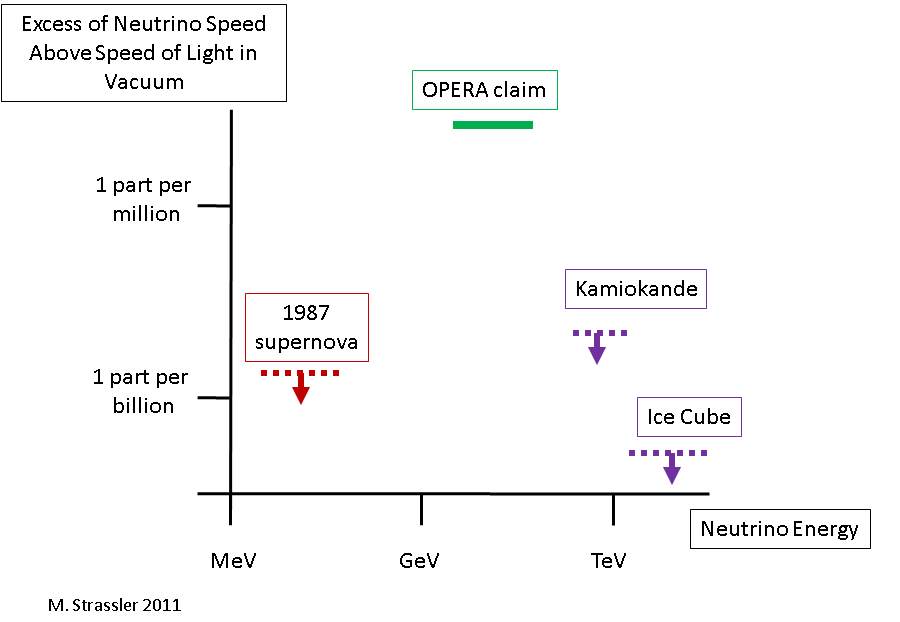
Before we get into the argument, let’s get a couple of things straight. First, neutrinos from supernova 1987a (as I explained here) arrived so close in time to the light from the supernova that their speed cannot have exceeded that of light by more than a few parts per billion. The energy of the supernova neutrinos was a few hundred times smaller than that of the neutrinos in the OPERA beam, so we cannot conclude from this observation that OPERA’s result is wrong, but it is something to keep in mind. If OPERA is right, the effect they observe must be growing with the energy of the neutrino.
You might wonder whether the supernova neutrinos are of one type, and might have one speed, while those at OPERA might be a different neutrino-type and travel at a different speed. This possibility, as Coleman and Glashow pointed out in their papers from the 1990s, is excluded by the various experiments on neutrino oscillations, whose internal self-consistency would be badly broken if different types of neutrinos traveled at speeds as different as would be required to reconcile the supernova neutrinos with OPERA’s neutrinos. In fact, the constraints on the differences between the speeds of different neutrino types is at the level of ten parts per billion trillion! We can forget about that possibility being important.
From these two paragraphs we conclude that if OPERA is right, the speed of all three types of neutrinos must increase with energy, differing from light speed by a few parts per billion or less for neutrinos with 0.01-0.04 GeV, and differing at a few parts per hundred thousand for OPERA’s neutrinos, with 10-40 GeV of energy. (For a reminder of what a GeV is, click here.)
The Cohen-Glashow argument
If you know about Cerenkov radiation (if not, read about it here), you know that any electrically-charged particle traveling faster than the speed of light will radiate bright light. Now that’s not supposed to be able to happen in empty space (vacuum), since nothing (if Einstein’s relativity is precisely correct) should be able to go faster than that. But in water, the speed of light is only three-quarters of what it is in vacuum, and high-energy particles can easily go faster than light travels in water. Muons from cosmic ray showers (sprays of particles created when high-energy particles from outer space hit an atomic nucleus somewhere high in the atmosphere) do it in droves, raining down on the ocean at close to the speed of light in vacuum. Moreover, Cerenkov light is used all the time in particle physics experiments; it serves as one of the best ways to detect and measure the properties of high-speed particles that can travel macroscopic distances.
Now neutrinos are not electrically charged, and at first glance they will not emit Cerenkov radiation. But this is not quite true, because neutrinos do have a very small magnetic moment (i.e., they act like extremely weak magnets) that is generated by a quantum effect involving the electromagnetic and weak nuclear forces. Consequently they too will emit a very dim form of Cerenkov radiation. It is too dim, however, to affect the OPERA measurement.
But then Cohen and Glashow point out that, for neutrinos, there’s a more important process… not obviously more important, and still small… but big enough. Just barely, too. This is a nice example of why in the end one has to stop talking and carry out honest and detailed calculations in physics; it often happens that you can’t guess the answer, and you have no choice but to follow the equations to their logical conclusion. The basic idea is that a neutrino that travels fast enough can potentially lose energy by emitting a particle and a corresponding antiparticle through effects of the weak nuclear force. Specifically, if a neutrino could travel faster than electrons could travel, then with sufficient energy the neutrino could emit an electron and a positron.
Well, are the OPERA neutrinos traveling faster than electrons can travel? Yes, because of what we know about electrons from Cerenkov radiation. We know that electrons at energies below a TeV cannot travel faster than the speed of light in vacuum by more than one part in a thousand trillion, because if they could, they would Cerenkov radiate even in vacuum. In this case we would never observe the high-energy electrons that we do in fact see in experiments. And therefore, if neutrinos travel faster than light by a few parts per hundred thousand, then they likewise travel faster than the maximum speed of electrons by about the same amount. It follows that for sufficiently high energy, neutrinos can spit off an electron-positron pair.
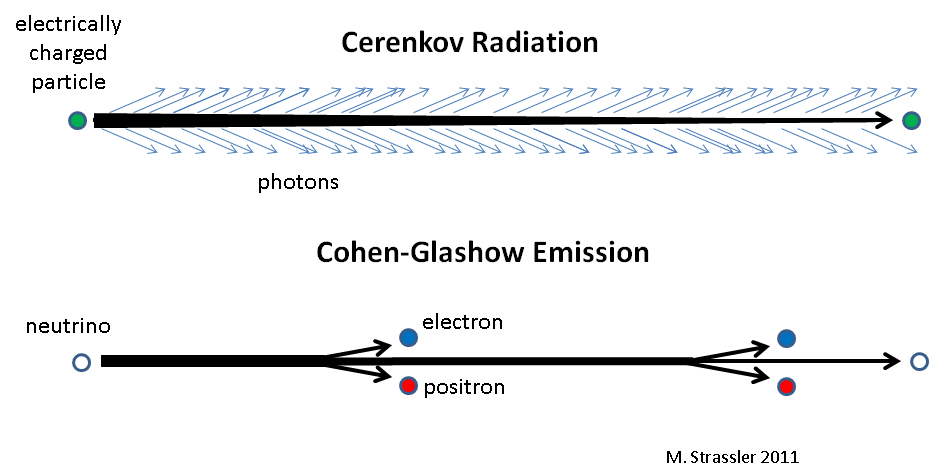
This process is like Cerenkov radiation, but only in part (see Figure 1). Cerenkov light is emitted as large numbers of photons, of many frequencies, virtually continuously. It is a process one can (and Heaviside apparently did) calculate in classical physics of the 19th century. But the type of radiation process discussed by Cohen and Glashow is very different. Because the weak nuclear force is indeed very weak in this context, an electron-positron pair is emitted only rarely as the neutrino travels along, with the first emission happening after some tens of kilometers; it is something that happens in discrete jumps, an inherently quantum mechanical phenomenon. It perhaps deserves its own name, so in this article I will call it Cohen-Glashow emission, or CG emission for short.
Now the calculation begins: how often does CG emission occur? And how much energy is lost along the way? According to Cohen and Glashow, if OPERA were correct — if neutrinos could travel faster than the speed of light in vacuum by the amount claimed by OPERA — then the higher-energy neutrinos among them could not have made the 730 kilometer trip from CERN to the Gran Sasso laboratory without losing a significant fraction of their energy. In fact, with each CG emission the typical high-energy OPERA neutrino loses 3/4 of its energy in one step! More precisely, the OPERA beam has a significant fraction of its neutrinos in the 20-40 GeV range, along with others down below 20 GeV. CG emission over 730 kilometers ought to have severely depleted the higher-energy neutrinos, moving almost all of them down well below 20 GeV. (The number they actually quote in the paper is even lower — 12.5 GeV — but I’m being a bit conservative here, see below.) This energy redistribution (see Fig. 2) would have been easily observed by OPERA. In fact, OPERA shows data in their paper (see Figure 13) indicating they have hordes of neutrinos above 20 GeV. Quoting from the OPERA paper “Data were then split into two bins of nearly equal statistics, including events of energy lower or higher than 20 GeV. The mean energies of the two samples are 13.9 and 42.9 GeV.”
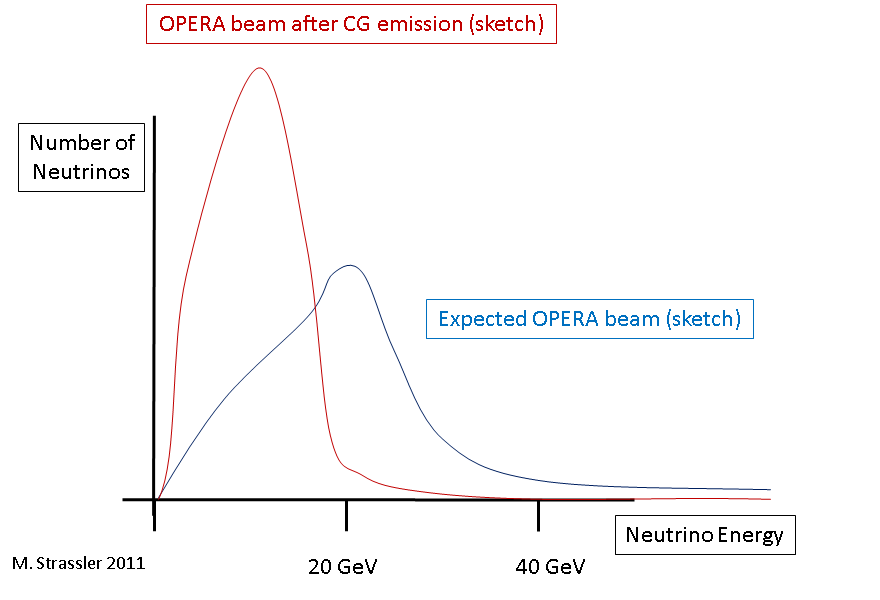
And thus the claim of Cohen and Glashow is that OPERA is inconsistent with itself — that it could not have seen a speed excess without an energy distortion, the latter being easier to measure than the former, but not observed. The upshot, then, is that OPERA’s finding that its neutrinos arrived earlier than expected cannot be due to their traveling faster than the speed of light in vacuum. Something is probably wrong with OPERA’s expectation, not the neutrinos.
One loophole you might worry about is that OPERA claims a measurement of a 60 nanosecond early arrival for its neutrinos. But all measurements come with uncertainties, and the uncertainties on the OPERA measurement are 10 to 15 nanoseconds, depending on how you combine the various sources of uncertainty. So while 60 might be the preferred experimental value, 30 nanoseconds might be the true value in nature. But my estimate is that even if OPERA’s result were an observation of a 30 nanosecond effect, the impact of CG emission on the neutrino beam would still be very significant. (The 12.5 GeV number in the paper would move up close to 20 GeV.) It doesn’t appear it would be possible for both the Cohen-Glashow paper and OPERA’s result to be correct simultaneously.
But these details almost don’t matter, because Cohen and Glashow then put another chunk of powerful evidence on the table. They point out that neutrinos have been observed, at two other experiments, SuperKamiokande and IceCube, 100 to 1000 times more energetic than the neutrinos in OPERA’s beam. These neutrinos come out of the earth having traveled many hundreds or thousands of kilometers across interior of the planet. The fact that these neutrinos did not lose most of their energy while traveling all that distance implies that they, too, did not undergo CG emission. In short, they must have traveled very close to, and conservatively no more than about fifteen parts per billion faster than, the speed of light in empty space. (The limit from IceCube data may be as good as ten parts per trillion!)
And so where do we stand, according to Cohen and Glashow?
- We have supernovas neutrinos with energies 100–1000 times smaller than those in OPERA’s beam that travel within a few parts per billion of light speed.
- We have neutrinos with energies 100–1000 times larger than OPERA’s that travel within a few tens of parts per billion of light speed.
- We have OPERA, which observes an effect of 20 parts per million, and yet if neutrinos traveled as fast as that, OPERA should have seen its high-energy neutrinos depleted before they even arrived, due to CG emission, but did not.
Can we conclude that the OPERA result is impossible? No. Implausible? Deeply.
Are there loopholes? Of course there are — theorists are very clever — and so it is too early to say that the curtain on OPERA has been lowered for good. I’m sure various people have one or another way to shut off Cerenkov-type radiation. But the story on the OPERA stage is beginning to look dreadfully contrived.
[I would like to thank Andy Cohen for clarifying a few points in his paper for me.]



63 Responses
I’m with you as far as “… any electrically-charged particle traveling faster than the speed of light will radiate bright light.”
But then I see this
“Now neutrinos are not electrically charged, and at first glance they will not emit Cerenkov radiation. But this is not quite true, because neutrinos do have a very small magnetic moment (i.e., they act like extremely weak magnets) that is generated by a quantum effect involving the electromagnetic and weak nuclear forces. Consequently they too will emit a very dim form of Cerenkov radiation.” which leaves me a bit uncomfortable.
Has this “very dim form of Cerenkov radiation” ever been observed?
It appears not as I read this
“no superluminal Cherenkov like e+e- pair or gamma emission event has been directly observed inside the fiducial volume of the “bubble chamber like” ICARUS TPC-LAr detector”
in http://arxiv.org/abs/1110.3763
On author asks “Since Neutrinos have never been observed directly making Cherenkov Radiation – why should anyone imagine they should?”
http://cosmologyscience.com/cosblog/must-ftl-neutrinos-cause-cerenkov-radiation/
No, no one has ever observed this extremely tiny effect. I put it in there for logical completeness, not because I thought it was practically observable.
“Why should anyone imagine that they should [radiate e+e- pairs]?” Because when you have a consistent theoretical framework that predicts all sorts of things about all the known particles of nature — I refer to quantum field theory, which correctly predicts emission of neutrinos by the sun, absorption of neutrinos by atoms, decay of pions and W particles to neutrinos, etc. etc. etc. etc. etc. — and which also correctly predicts Cherenkov radiation from all sorts of known particles in all sorts of well-studied situations — you should continue using that theoretical framework until experimental tells you that it breaks down. You should not assume: “oh, because I haven’t studied this particular situation, I should distrust my theoretical framework altogether.”
In other words, you certainly should “imagine” it; that’s what prediction is all about, imagining that your theoretical framework derived from and tested on previous experiments will work in other experiments that you haven’t done yet. This is induction, yes? Fundamental to science and engineering.
If you don’t do that, what was the point of science in the first place? You’d be afraid to imagine how to use engineering to design rockets into space because you’d never done it before. You’d be afraid to build the Large Hadron Collider [LHC] because “who knows, maybe all our laws of nature won’t work for such a machine?” You’d never have had any reason to think that the LHC could make Higgs particles, or even top quarks; no one had ever seen top quarks produced in proton-proton collisions before the LHC, they’d all been made with proton-antiproton collisions at the Tevatron.
The point of science is to use existing frameworks and see what they predict; if experiment disagrees, then it’s time to revise the framework. But if you stop using your existing frameworks — if you don’t study the logical consequences of what you think you’ve learned — then it is impossible to make progress.
I was very careful on this website to point out that the prediction by Cohen and Glashow of Cerenkov-like emission of electron-positron pairs was a strong argument against OPERA being a correct experiment — but not a refutation, because logically the argument required assuming the existing theoretical framework was not breaking down in a very subtle and surprising way. The reason the argument was strong is that Cerenkov radiation is a ubiquitous and very simple phenomenon, and eliminating it for neutrinos without causing problems for other earlier experiments would have been a huge theoretical challenge, perhaps impossible. But a strong argument is not a direct refutation; that had to await ICARUS’s more recent result from March, where they made the same measurement as OPERA and got a different result.
In any case, OPERA was wrong. So this is moot. And for various technical reasons having to do with the difficulty of setting up a suitable experimental situation, I suspect no one will likely observe Cherenkov emission of any sort off of neutrinos, at least not anytime soon.
Many thanks for your very understandable explanation of CG emission. The level you write at is good for me, so I have had a piece of luck finding your blog, through SWINY actually. Your conversation with Lee Smolin was interesting as well. I confess not to have read ALL the comments. Sue
I found an interesting explanation of the “OPERA” superluminosity. My friend John Celenza helped to write, review, check (or put in calculations) and clean up our short piece. Thanks for your patience John!
It has 399 words and 2454 characters including footnotes. The result if true is a very basic change in how we view the Universe.
Any suggestions on where to publish or post it?
I would like to call your attention to a wave packet deforming effect that could produce an early arrival, similar to what happens in pulse Lasers. This has been proposed in arXiv 1110.0243 and more recently arXiv 1112.0815 where 65 ns early arrival for short 3ns LHC pulse had been obtained!
Whatever the assumed neutrino propagation distance used to estimate the time-of-flight for simulated photons traversing the same distance, the actual neutrino traversal distance cannot be precisely determined and in fact may be somewhat variable, correct?
Do you expect that experiments at other laboratories can conclusively determine neutrino speed?
It would seem that an effort to produce a theoretical explanation for a yet to be reproduced experimental result is premature.
The fact is that multiple careful tests have been run including the latest post FTL OPERA announcement. All have given the same FTL result. This strongly suggests that the assumptions of the experiment must be examined, along with a reproduction of the experiment by competitive peers.
Has any credible party looked at OPERA’s assumptions? Any known effort to reproduce the experiment elsewhere?
Can you link us up?
As you say (and as I emphasized too, http://profmattstrassler.com/articles-and-posts/neutrinos-faster-than-light/opera-comparing-the-two-versions/ ) the repeat of the experiment eliminates one set of possible assumptions from question, but others remain.
I can’t tell you the situation exactly (you’d have to ask people at OPERA) but many credible people have looked at OPERA’s assumptions to a greater or lesser degree. But it hasn’t been terribly coherent as a process. (I don’t think a panel has been formed to do it, though it might be wise to assemble one, given the importance of the measurement.) OPERA as a collaboration has tried very hard to answer all questions that have been posed to them about their assumptions. They are also relying to some degree on the GPS and precision-timing/distance community, which has also been helping to answer questions. The paper is currently under review, I believe, so perhaps the reviewers will include experts who can dig even deeper than the community already has.
There are efforts to reproduce the measurement at MINOS (look at the comment by “Mayly” in the comment section to http://profmattstrassler.com/2011/10/26/a-few-tidbits-from-nagoya-including-opera-news/) I am sure many discussions about other experiments are underway, but these things take time… OPERA itself was not built in a week or a month, and any new experiments will probably take years to design, propose, fund and build.
If, for ANY reason, the estimated 731,278+/-0.2 meters distance traversed by neutrinos was overestimated by about 18 meters then the detected neutrinos DID NOT exceed the speed of light.
– Whatever the distance estimate used in calculating the comparative time-of-flight for light in a vacuum between CERN and the Gran Sasso neutrino detector, the 61 ns discrepancy would be fully accounted for by a distance of about 18 meters.
– The actual neutrino propagation path cannot be definitively determined. The neutrino propagation distance cannot be precisely determined.
– The approximate 730 km estimated traversal distance is dependent on a very complex method using coordinated GPS locations and standard geodesy routines. However, any relativistic spacetime distortions produced by the propagation of particles at near the speed of light within the Earth may not have been consistent with the methods employed by standard geodesy software. In this case the actual path length of neutrino propagation may have been overestimated.
As a layperson I cannot assess these issues, but some may be addressed in the report: Wolfgang Kundt, (2011). “Speed of the CERN Neutrinos released on 22.9.2011 – Was stated superluminality due to neglecting General Relativity?”, http://arxiv.org/abs/1111.3888v1
Cohen and Glashow explain why the OPERA neutrino anomaly is not a case of neutrinos traveling faster than c. Is the GPS timing slightly off? Are photons traveling slower than general relativity theory predicts because of dark matter or the Rañada-Milgrom effect? “To think, as dark-matter advocates say they do, that the universal MOND regularities exhibited by galaxies will one day be shown to somehow follow from complex formation processes, is, to my mind, a delusion.” — Mordehai Milgrom, “DM or MD?”, p. 1
http://arxiv.org/pdf/1101.5122v1 “MD or DM? Modified dynamics at low accelerations versus dark matter” by M. Milgrom, 2010, Proceedings of Science
Consider a hypothetical Principle of Dark Matter:
The -1/2 in Einstein’s field equations should be replaced by -1/2 + dark-matter-compensation-constant. If nature provides a curling-up mechanism for M-theory, then the dark-matter-compensation-constant is an approximate, heuristic mechanism that represents superpartners found in the supersymmetry predicted by Seiberg-Witten M-theory. If nature operates according to modified M-theory with Wolfram’s automaton, then the dark-matter-compensation-constant is a totally precise constant that can be calculated using Planck’s constant, Newton’s gravitational constant, the speed of light in a vacuum, and the Fredkin-Wolfram constant. Dark matter might be thought of as D-brane reinforcement of the Einsteinian gravitational signal. The Pioneer anomaly and Fernandez-Rañada’s idea of anomalous gravitational acceleration of clocks yield the value sqrt((60±10)/4) * 10^-5 for the dark-matter-compensation-constant. (Note that this value is within the range for explaining the OPERA anomaly.)
http://en.wikipedia.org/wiki/Pioneer_anomaly
A cop out might be an exaggeration; it is a very interesting paper but all it shows is that the OPERA result cannot be explained by any current model… That is all well and good but personally I don’t think this is enough as observational data says otherwise… It is not just a single observation that we can dismiss as erroneous….So in addition I think we need an explanation as to why such an anomaly can occur ….. E.g. the result is impossible (as argued by Cohen and Glashow) but under such and such circumstances we can explain it.
Something that you’ve said makes me think (perhaps incorrectly) that you are confused about how the OPERA measurement works. You said “It is not just a single observation…” That statement isn’t true… it is a *single* observation. It took 16,000 neutrinos to do it, because of the convoluted way the measurement is made (using long pulses and then doing a fit to the whole data set at the end.) That ONE observation is at most 6 standard deviations away from expectations, and with less aggressive statistical analysis, 3 or 4. As you know, any one measurement could easily be wrong, and so it makes sense to ask whether it is consistent. If you were thinking OPERA made 16,000 measurements, you would have had the wrong impression about the experiment, and also about Cohen and Glashow’s point. [Or am I confused about your confusion?]
In fact this is precisely why the new way of doing the measurement (with short pulses) detailed in my more recent post (http://profmattstrassler.com/2011/10/26/a-few-tidbits-from-nagoya-including-opera-news/) is so much better. Each neutrino will be, by itself, a rough measurement. My understanding is that they will have of order 10 such measurements by the end of the year.
This is indeed an excellent point. But 16000+ events are still 16000+ detections that lay as basis for the measurement and the whole experiment seem to be very precise. I may well be wrong in my opinion here but I really would have liked some more reasoning and discussion in the Glashow-Cohen paper as to how we can explain the observation – Not just saying it is impossible and that this in itself is the explanation…
I really must emphasize again that it is not 16000 detections of a violation of the speed limit. This is one of the main points I made right after the OPERA presentation. For instance. If you took only 1000 of those neutrinos, the measurement simply could not be made at all. Even with 8000, the signal would barely be significant. It becomes significant only with the use of the full data set of 16000. That’s because of the use of very long pulses; you cannot tell, for any one neutrino, how long it took to travel. You have to study the collective pattern of neutrino arrival times, compare it with the collective pattern of neutrino departure times, and infer from the collection that the arrival times are early.
The explanation of what the experimentalists did wrong could come from many different sources. There have been dozens of proposals from both theorists and experimentalists. They all contradict each other, so most of them (and perhaps all of them) are wrong. Even if Cohen and Glashow proposed something, you would not know at this point whether they were correct until this was confirmed by the OPERA experiment. Moreover, keep in mind that sometimes the error made by an experiment is never found.
So of course we would all like to know what went wrong, if indeed something went wrong, and we’d like to know as soon as possible. But this might take months or years.
Meanwhile, explaining the experiment was not what Cohen and Glashow were trying to do. They were pointing out a contradiction between standard Cerenkov radiation processes and the OPERA experiment — which implies some useful information: that if OPERA is correct, standard Cerenkov radiation processes must somehow be disarmed. That is valuable knowledge, in and of itself.
I recently sent the following observation to Physics Today, which they probably won’t publish, missing the humor in the sonnet, but I’d like to put here for your amusement.
Neutrinos oddly go..
Neutrinos created from pulsed Muons at Cern
aimed at a cave below fair Italy’s Gran Sasso,
pass through leagues of rock without concern
as if it were just glass, their passing so
unhindered. But with puzzling oddity, they
get there much too early, faster than light,
and, as if bouncing in a mirrored way,
change sex promiscuously.. It’s not right,
upsetting Physicists! Anomalies they shout!
But maybe it’s just refraction, just as light
must bend into density, neutrinos may bend out,
changing speed to keep its wave coherent.
Sleep well dear Einstein it’s only a curiosity
in our random, weird quantum particularity.
Kent Bowker Optical Society, Emeritus
Oct. 16, 2011
The Glashow and Cohen paper may be elegant but it is not an explanation of the OBSERVED data… they are merely saying… OPERA is wrong and OPERA is saying, well that this is what we observed… So it is a theoretical cop out that shows how the OPERA result is inconsistent but it does not explain the OBSERVATION.
Since when is pointing out a potential internal inconsistency in an experiment a “cop-out”?
Not exactly. If only a neutrino is affected by Cohen and Glashow “reduced Cherenkov” drag effect only very few times while crossing OPERA’s panels versus billions of neutrinos produced by CERN and if the detecting hardware OPERA is the most precise and sensible in use today, simply I can state that Cohen and Glashow “reduced” Cherenkov drag effect pratically doesn’ t occur because it is a too rare phoenomenon to be statistically relevant. Simply only a cat versus billions of dead cats involved in the paradox of Schrödinger sometimes survives but all the rest of cats are killed. For me what happens in such labs is too far from our normal experience in every day life consequently the meaning of the word “possible” is as far as another galaxy from the word “occurs” and a “possible phoenomenon” sometimes doesn’t occur at all in labs. No wine = no fiesta but someone else is drinking my wine far from here I am.
Cohen and Glashow do a calculation. They don’t state the result explicity, but in conversation with Cohen he told me that the probability of a 40 GeV neutrino making it to OPERA without undergoing Cohen-Glashow radiation is exp[-75], or 10^(-31), extremely small. Equivalently it means the typical neutrino at 40 GeV undergoes this process after about 10 of the 730 kilometers it needs to travel. I haven’t checked this myself. But equations beat words every time. (The effect, by the way, need not occur inside OPERA; it typically occurs in the rock during the transit from CERN to Gran Sasso.)
Well, the neat thing about the Standard Model is that however improbable an event might be, nothing is impossible. Yo Popper! I prefer the Smolin approach. Rather than simply refute the anomalous data, take a look at how it might be legit. Those physicists at CERN are no bums and they potentially risk a great deal releasing the superluminal results. But what do I know, an unordained, layman.
One should always do both; look for errors and inconsistencies (turns out to be easy in this case) and find high-quality ways for the data to be consistent (turns out to be very hard in this case). Sometimes it’s the other way round; it is hard to find inconsistencies and it is easy to make the data consistent. So OPERA is not doing so well, by comparison.
…simply I argue that bremsstrahlung regards only electrically charged & high speed particles flowing through electrical fields and neutrinos aren’t electrically charged particles even if very fast ones. Sun produces so many fast neutrinos per square meter flowing in any second through our bodies that a sort of Cherenkov radiation produced by solar neutrinos have to fulfill our natural environment. But this “phoenomenon” not happens at all. Cherenkov radiation is uncommonly detecteable only in few ph. labs or nuclear power plants. Neutrinos do not cause bremsstrahlung and so it is..
You aren’t seriously suggesting that Cohen and Glashow don’t know how to calculate processes involving weak-nuclear forces.
I’m a little confused about something: CG use the word Bremsstrahlung in the abstract and Cerenkov in the body. As I understand it, bremsstrahlung does not require exceeding the speed of light by the emitting particles. Is the abstract mis-speaking? Perhaps bremsstrahlung is a generic term that encompasses Cerenkov (because “braking” occurs).
This is just an example of the slipperiness of terminology, I think. Nowadays the term bremsstrahlung is used somewhat broadly than its original technical meaning. In this context, it just means that one particle spit off another, with no additional assumptions. That would then include Cerenkov-like radiation as a subclass. So in short there’s just one physical process here, and two ways of describing it.
Hi Matt,
I was thinking about what Opt was saying.
This leaves an open area of thinking in my mind about the oscillation factor and the collision point in relation to the QGP created?
Also would a GZK limit in terms of cosmic energetic particle collision be what is required by Cohen-Glashow?
Best,
From what I recall, extra dimensions that give a shorter path to neutrinos have been proposed as a scenario to explain the OPERA results. Would the above result still apply in that case?
What assumptions go into the extrapolation of the (known) weak interactions to superluminal neutrino speeds? Does one not implicitly assume some dispersion relation (or mass-shell condition) for the neutrinos in this continuation? Or do they continue the equations for “conventional” massive neutrinos to off-shell values of their momenta? What is the justification for doing so?
Remember that the effect OPERA is claiming is only a few parts in 100,000. Details of the weak nuclear force are very well tested for neutrinos of these energies, through experiments such as SLAC, LEP, the Tevatron and the LHC. An effect this small would not have been noticed in the measurements that confirm the equations that we use for the weak nuclear force, but conversely, such a small effect would not affect the use of the weak nuclear force equations in this context. In other words, one does not need to know the equations governing the weak nuclear force with high accuracy in order to use them here.
Could you please *stop* trying to explain the OPERA result with new physics ?
The reason for the discrepancy is with high probability a wrong distance measurement, the CERN coordinate is incorrect:
http://scienceblogs.com/startswithabang/2011/09/are_we_fooling_ourselves_with.php (currently last comment)
I’m sorry, but you do not understand. These people are not trying to do that. They are trying to show that *old* physics makes the experiment inconsistent.
Dear Prof. Strasser,
could You give some more details about the CG emission itself and how the electromagnetic and weak interactions are involved? For example I`d like to see the Feynmann diagramms …
Anyway, I like the beautiful and very clear explanations in this post that show how this mechanism leads to a self-contradiction of the Opera experiment 🙂
I’ll try to find time to add a Feynman graph to the post. But you can draw it yourself: a neutrino emits a Z particle (virtual) which turns into an electron-positron pair. The reason I hesitate to do this is that the graph looks nonsensical to a non-expert who doesn’t know what a virtual particle is. I need to write an article on “virtual particles” and what that term does and doesn’t mean. [It’s very confusing for the non-expert, who doesn’t have any reason to know what a Green’s function is and does.]
Ok, thanks 🙂
Hi Dr. Strassler,
I’m a fellow scientist, though I’m a research meteorologist, not a particle physicist, so quite obviously I don’t have anywhere near the deep understanding of particle physics that folks who actually work in it do! Recently, however, I have become fascinated with particle physics and have been reading quite a bit of material about it, including the material on your excellent website (in fact, it has inspired me to perhaps make a similar website devoted to explaining meteorology to the public; we’ll see if I can make time for that!). I thus think that I have reasonably well-informed “layman’s” understanding.
Anyway, I have read through the Cohen and Glashow paper and your commentary, and I think I understand the basic gist of it. One thing really bothers me about it; namely their claim to “refute the superluminal interpretation” of the OPERA results. Now, at least on the face of it, this at least seems to me to be a bit over the top. I agree that they bring experimental evidence to bear in their argument, and aren’t relying on a pure theoretical framework, but it seems to me that they still interpret that evidence through the lens of theory, namely that any high-energy superluminal neutrinos would have lost energy through CG emission, analogous to Cerenkov radiation. Am I completely off my mark here? But it seems to me that this at least partially is a theoretical argument, and it seems rather odd for a scientist to claim that a (at least partially) theoretical argument can “refute” an experimental result. It seems to me that only discovering the source of the error and/or failing to replicate the results in a well-constructed followup experiment can actually do the real work of “refuting” the results. And if the results are confirmed, and neutrinos really can travel FTL as near as we can possibly tell with our best measurement techniques, it seems that even if we don’t have a theory to explain it, that’s not a mark against the evidence.
I’m bringing this up because it’s quite possible that I have misapprehended the nature of the Cohen and Glashow argument, and I was hoping you could correct any misconceptions I might have. For example, has CG emission been observed? If so, my entire objection more-or-less evaporates. Also, please don’t take this as a jab against the authors themselves. I understand they are eminent and well-respected scientists.
Many thanks!
Dan D.
I had a similar reaction to the word “refute”. I think they chose the wrong term — what they did was present an argument that constrains the types of theories that could be consistent with OPERA data. In other words, only theories that don’t generate Cerenkov-type radiation would be permitted.
Dear Matt,
Thanks for your mention of the Cohen and Glashow paper. There is however an interesting loophole in their argument, which is that it assumes that the relativity of inertial frames is broken, so there is a preferred frame. But it is possible to modify special relativistic kinematics by non-linear terms while preserving the principle of relativity. As explained in arXiv:1110.0521, special relativity can be modified without breaking the principle of relativity, leading to modifications in dispersion relations, lorentz transformations and energy-momentum conservation laws. In these cases, superluminal neutrinos can be stable. An example of a framework where this is developed consistently is relative locality, arXiv:1101.0931.
Of course, the most likely hypothesis is still that the experiment is wrong. Even within a relativistic framework one still needs to explain the lack of universality and scale of the OPERA claim, because there are very good limits on non-linear terms in the relativistic kinematics of photons and electrons up to the Planck scale. But its still interesting to know if there is a second most likely hypothesis. Cohen and Glashow have shown convincingly it cannot be lorentz symmetry breaking. But it might be a modified version of special relativity.
Thanks,
Lee
Thank you, Lee, for this remark. Are you suggesting the loophole is large enough that the limits on nonlinear terms would allow for an effect as large as OPERA measures? And what precisely do you mean by “lack of universality” — neutrinos vs other particles, or something else?
Dear Matt,
The point is that there are no limits from frame dependent threshold effects such as discussed by Cohen and Glashow (and also in other papers by Gonzalez-Mestres and Bi et al) in a relativistic theory. Superluminal neutrinos can be stable in a deformed special relativity context.
But there are several other challenges to finding a model that fits all the data.
-The effect is much larger than expected by quantum gravity considerations,would suggest, ie the OPERA delta v is not order E/M_Planck but larger than this by 10^{12}-which is a factor of E/neutrino mass difference.
-An effect of this order is ruled out for photons and electrons, so there would have to be some reason it applies only to neutrinos.
-There is no power law dependence on energy that is consistent with all the data on neutrino speeds including OPERA, SN1987a and Fermilab 1979.
So we are pointing out a loophole but we do not so far have a realistic model that can account for OPERA.
Thanks,
Lee
Do I understand correctly that your approach allows for deformations of relativity that are *not* universal among particles?
If so, then I have two questions.
1) do you have any idea how you could make the deformations consistent with the electroweak theory [non-experts: the theory that combines the weak and electromagnetic forces] and still have electrons unaffected while neutrinos are affected [non-experts: the two particles are combined in the electroweak theory, see my posts on the Known Particles.]
2) the usual calculation of Cerenkov radiation nowhere uses relativity, as far as I can tell. It only relies on the fact that in *some* frame of reference the charged particle has a more rapidly rising “dispersion relation” [non-experts: relation between energy and momentum, and from there the mass and velocity] from that of light. So I am unclear why a non-universal relativity would avoid Cerenkov radiation; after all, it would still be present in ordinary materials. As long as in some frame the neutrino dispersion relation, which at low energy matches that of light, grows faster than that of both electrons and light (which you’ve just told me cannot differ from the usual one by one part in a trillion ), then it is kinematically allowed for neutrinos to decay to a neutrino and an electron-positron pair. Whether this statement is true in one frame or in all frames doesn’t seem to me to matter. It is enough that there is one frame in which I can make the argument. It might be more complicated to express in another frame… but that doesn’t matter. Or is there some alteration in the kinematics in modified relativity which makes this argument incorrect?
Let me make my second question simpler and more precise. Let us assume that we have a modified but non-universal form of relativity, and in this theory there is a massive charged particle X that can travel faster than light. I’ll assume usual relativity is a good approximation at low energies.
Let me also assume that there exists at least one frame in which rotational invariance is exact. [By the way, for OPERA’s neutrinos we know this must be the case for the earth’s frame to a good approximation, since OPERA sees no seasonal variation in its results.]
Now in this frame — which is the only frame I’ll ever appeal to — the energy E of an X particle is a function of the magnitude of its momentum, p, and not its direction. Therefore I have a simple dispersion relation E(p) [Energy as a function of the magnitude of momentum] for X,. Similarly I have another function E(p) for photons. These two functions tell me everything I need to know about the kinematics.
Now I ask: is the process X –> X + photon allowed *kinematically*. To answer this question, I would claim I only need E(p) for X, E(p) for photons, and the conservation of both energy and momentum. And I would claim that all I need to know is whether dE/dp for X at high energies exceeds E/p for photons at low energies. If so, then the conservation equations have a solution, and Cerenkov light can be emitted. And since, under my assumptions, X travels slower than light at low energy but faster than light at high energy, dE/dp for a sufficiently high-energy X *does* exceed E/p for low-energy photons. We still have to calculate the rate for this process, but the process is clearly not forbidden.
It’s a very low-brow argument, but that’s its power. Note I didn’t need to know any details of how relativity works. The argument seems to hold in all cases that I am aware of.
So how does your version of modified relativity evade the argument? Which of my assumptions or statements was flawed?
Dear Matt,
Thanks much for your further queries.
I believe the answer to the second query is that there are non-linear terms in the energy momentum relations, which prevent the decay in every frame. These are required to make the new dispersion relations and conservation laws covariant under the same transformations. This evades your argument because the non-linear terms depend on the ratio of energy to mass of each particle. This illustrated in the model we study in our paper.
The answer to the first question is no, we have no insight into how to make the deformations different for neutrinos and charged leptons without violating the symmetry of the electroweak theory. This is part of why we stress that we do not have a realistic model.