Matt Strassler [February 17, 2012]
Final draft form; comments welcome.
You’ve heard the famous statement that “a proton is made from two up quarks and a down quark”. But in this basic article, and this somewhat more advanced one, and in a recent post where I went into some details about what we know about proton structure, I’ve claimed to you that protons are chock full of particles, most of which carry a tiny fraction of the proton’s energy, and most of which are gluons, along with a substantial number of of quarks and antiquarks. [If this sounds unfamiliar, you should read those articles and posts before reading this one, which is a follow-up.] And I claimed that these complications make a big difference at the Large Hadron Collider [LHC].
What I want to do in this article is show you evidence that the statements made about proton structure in this post are true. After all, why should you have to take my word for such things? Let’s look at some LHC data, and see how it confirms these notions (though the current understanding of the proton itself arose three to four decades ago, through numerous past experiments which would form a story of their own.)
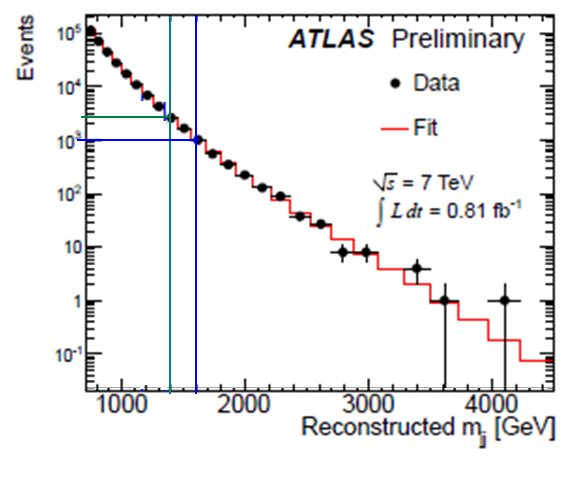
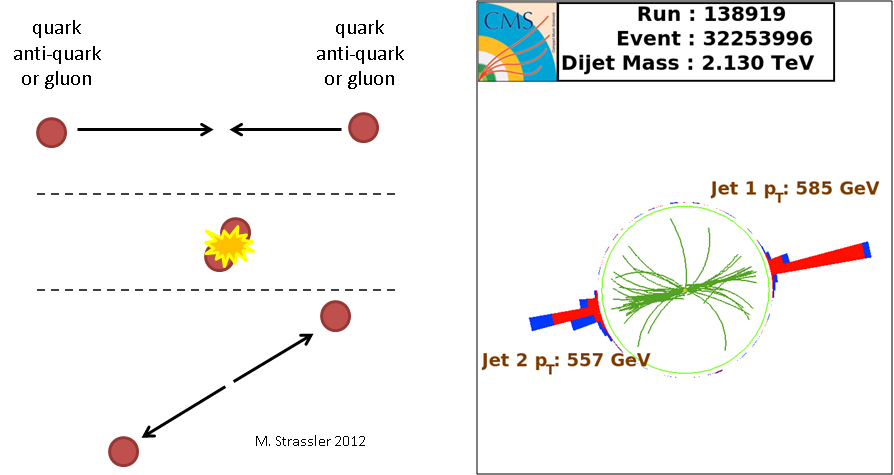
In the more advanced article I referenced above, I described the benefits (not all of them obvious) of running the Large Hadron Collider at an energy per collision of 8 TeV in 2012 instead of 7 TeV as in 2011. And at least one of the benefits — more Higgs particles for the same number of collisions — is a consequence of there being so many gluons in the proton. I showed you evidence [reproduced here in Figure 1] that most particles in the proton carry a tiny fraction of its energy. The plot in Figure 1 is made by looking at collisions in which something like what is shown in Figure 2 occurs: a quark or antiquark or gluon from one proton hits a quark or antiquark or gluon from another proton, scatters off of it (or might do something more complicated — for example, two gluons might collide and be converted into a quark and an antiquark), with the result that two particles (again, quarks, antiquarks or gluons) come flying out from the collision point. These two particles turn into jets (sprays of hadrons) for reasons you can learn by clicking this link. And the energies and directions of these jets are observed in whichever of the big particle detectors surrounds the collision point. That information is then used to infer how much energy the collision of the original two quarks/gluons/antiquarks must have had. (Precisely speaking, the invariant mass of the two jets, times c-squared, gives the energy of the collision of the initial quarks/antiquarks/gluons.)
The number of collisions of this type that have a given energy are shown in Figure 1, with energy in GeV running along the horizontal axis. Notice that its a logarithmic plot on the vertical axis: 105 means 1 with 5 zeroes, i.e. 100,000. So there were far, far more collisions observed at lower energy than at higher energy, consistent with the statement that most particles in the proton carry a small fraction of the proton’s energy. But the data shown only go down to 750 GeV, just over 10% of the collision energy.
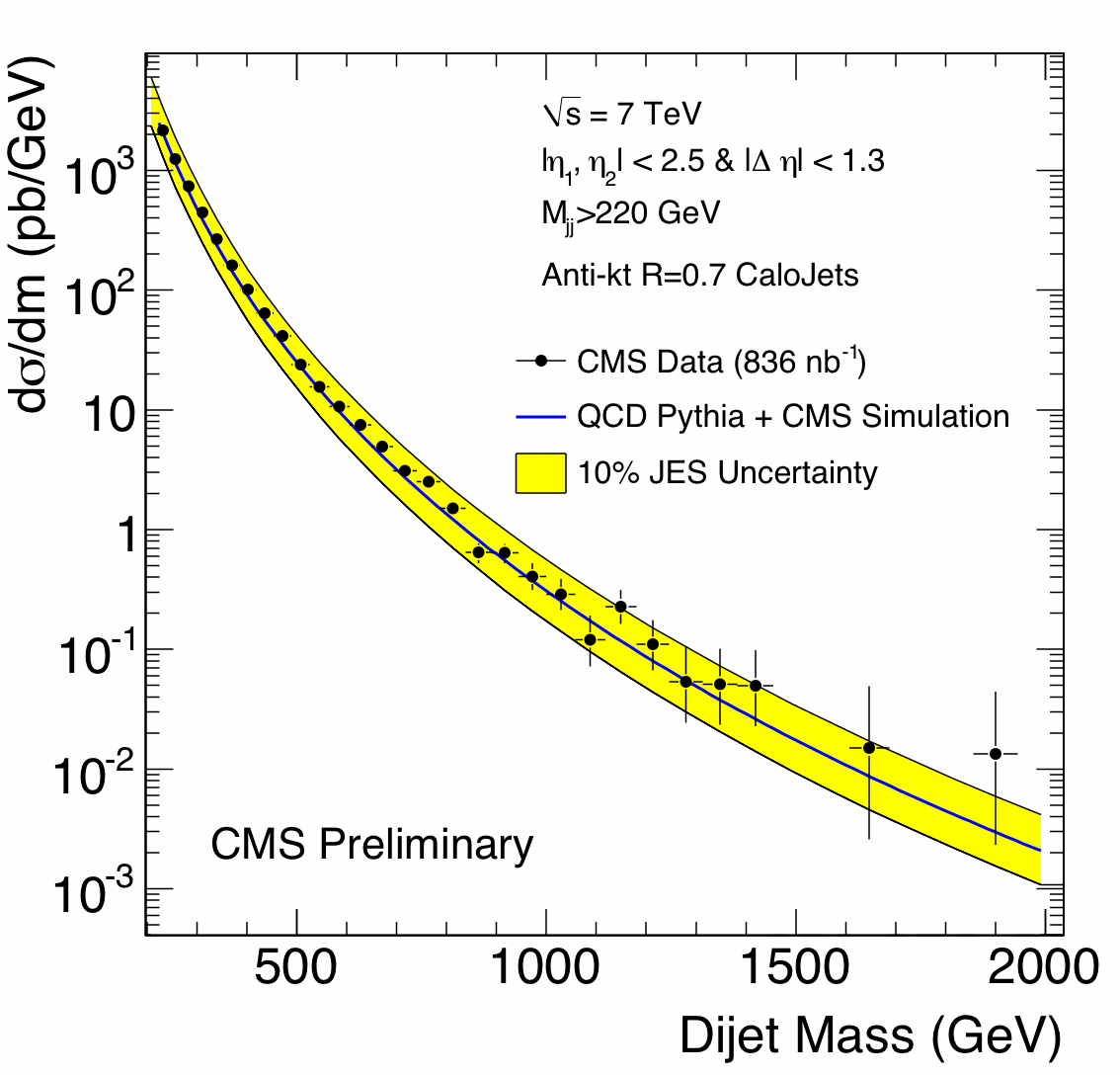
So let’s look at Figure 3, from the CMS experiment, which was taken in 2010, where they plotted collisions all the way down to 220 GeV. [What is plotted here — you can ignore this unless you study the plot in detail — is not the number of events but something slightly more confusing: the number of events per GeV, which means the number of events is divided by the width of the bin. ] You see the same effect continuing down as far as the data is plotted; the number of collisions of the type shown in Figure 2 is much larger at low energy than at high energy. And it continues to grow all the way down in energy until you can’t identify the jets anymore. There are many low-energy particles in a proton, and very few that carry a large fraction of the proton’s energy.
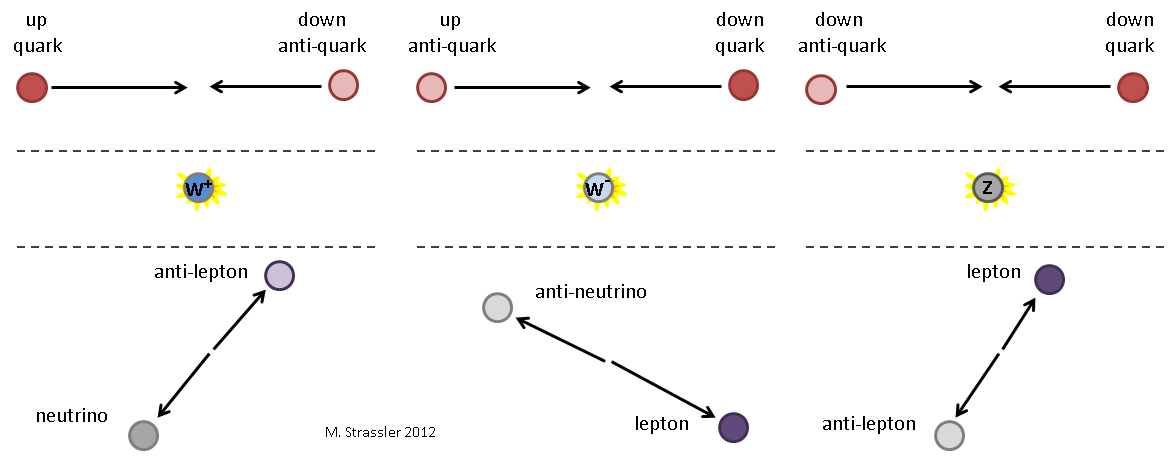
Now how about the claim that there are antiquarks in the proton? Three of the most spectacular processes that don’t look like the collision in Figure 1 and happen occasionally at the LHC (in one in a few million proton-proton collisions, roughly) involve the process: quark + anti-quark –> W+, W- or Z particle. These are shown in Figure 4.
The relevant data from CMS is shown in Figure 5 and Figure 6. Figure 5 shows that the rates for collisions to occur that produce (left) an electron or positron [i.e., anti-electron] and something undetected (presumably a neutrino or anti-neutrino), or (right) produce both a muon and an antimuon, are predicted correctly. The prediction is made by combining the “Standard Model of particle physics” (the equations used to predict the behavior of the known elementary particles) with the structure of the proton that I showed you on Wednesday. The big peaks in the data are due to the W particles and the Z particles produced. You see the agreement between the theory and the data is excellent.
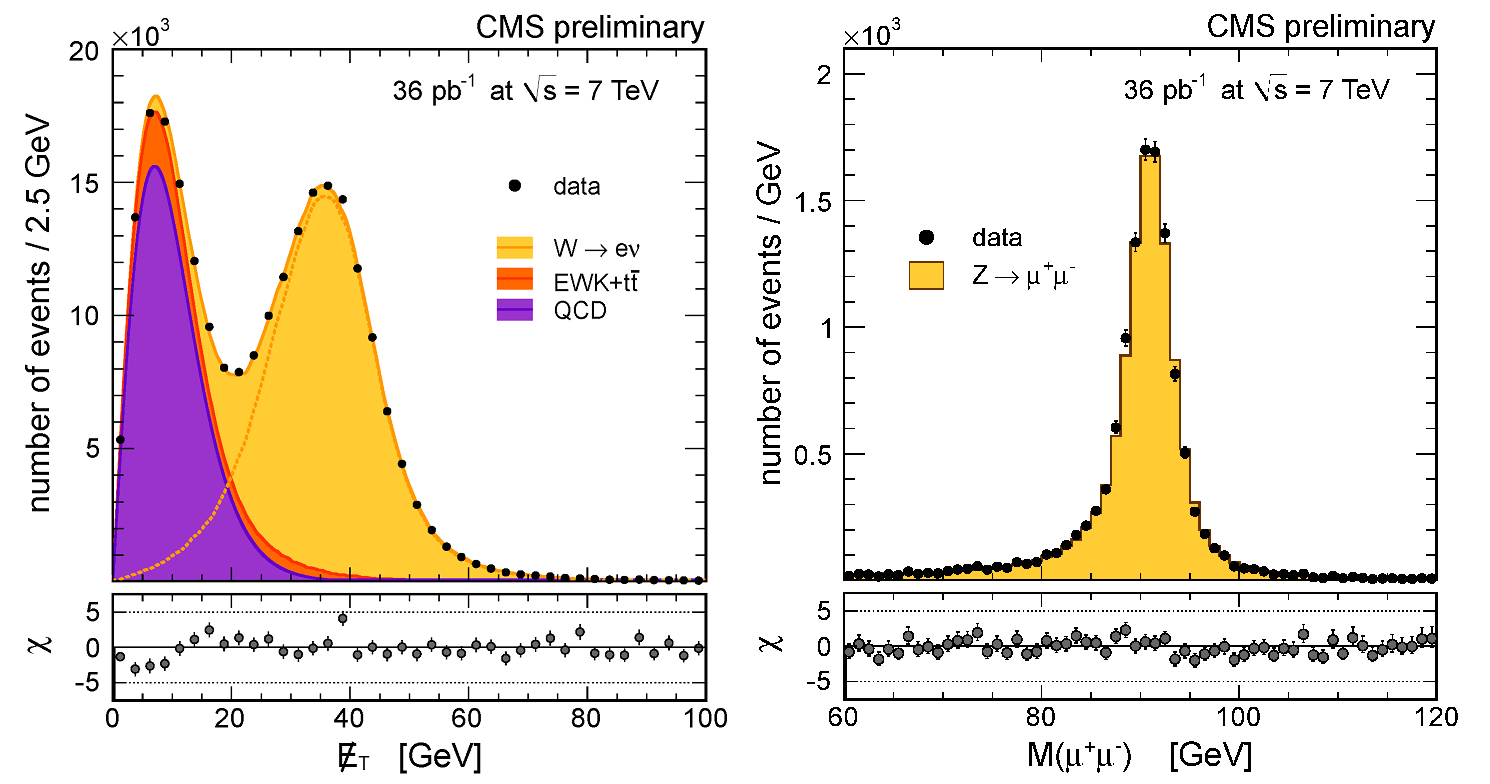
You can see this in even more detail in Figure 6, which shows that the rates for not just these but many related measurements — most of which involve quark collisions with anti-quarks — show excellent agreement of theory and data. [How to read this: Data (red dots) and theory (blue bars) never agree perfectly just due to statistical fluctuations, for the same reason that if you flip a coin ten times you won’t necessarily get heads five times and tails five times. The data points are therefore placed within an “error bar”, a vertical red band. The size of the error bar is such that for about 30% of the measurements the error bar should like outside the theory, but only for 5% of measurements should one expect the theory and the data point to be two error bars apart.] So you see that all the evidence confirms that there are many antiquarks inside a proton. And we do correctly understand how many there are that carry a particular fraction of the proton’s energy.


Now here’s a slightly tricky one. We even understand how many down and up quarks there are as a function of the energy they carry, because we correctly predict — to an accuracy of better than 10% — how many more W+ particles are produced than W– particles (Figure 7). The ratio of up antiquarks to down antiquarks is expected (and measured) to be close to 1, but we do expect more up quarks than down quarks, especially at higher energy. Looking at Figure 2, you can see that the ratio of W+ to W– particles produced should give an estimate of the ratio of up quarks to down quarks that participate in the making of W particles. But notice the ratio of W+ to W– in Figure 7 is measured to be 3-to-2, not 2-to-1. This, too shows that the naive picture of a proton as 2 up quarks and 1 down quark is far too simple. The simplistic 2-to-1 ratio is diluted, because of all the quark-antiquark pairs inside the proton, of which there are roughly equal numbers of up’s and down’s. The amount of dilution is determined by the mass of the W particle being 80 GeV; if you made it lighter, you’d see more dilution, and if you made it heavier you’d see less, because most quark-antiquark pairs in the proton carry low energy.

Finally, let’s confirm that most particles in the proton are gluons. To do this we use the fact that top quarks can be produced in two ways: one is by quark + antiquark –> top quark + top anti-quark, and the other is by gluon + gluon –> top quark + top anti-quark (Figure 8.) Well, we know we have the number of quarks and antiquarks, as a function of the energy they carry, basically right, from all the measurements shown in Figures 5 through 7. And from this we can use the Standard Model’s equations to predict how many top quarks would be produced through quark/antiquark collisions alone. But we also believe, from previous data, that there are many more gluons in the proton, so the process gluon + gluon –> top quark + top anti-quark should be more than 5 times larger. So that makes it easy to check if the gluons are really there; if they are not, then data should lie far below the theoretical prediction.
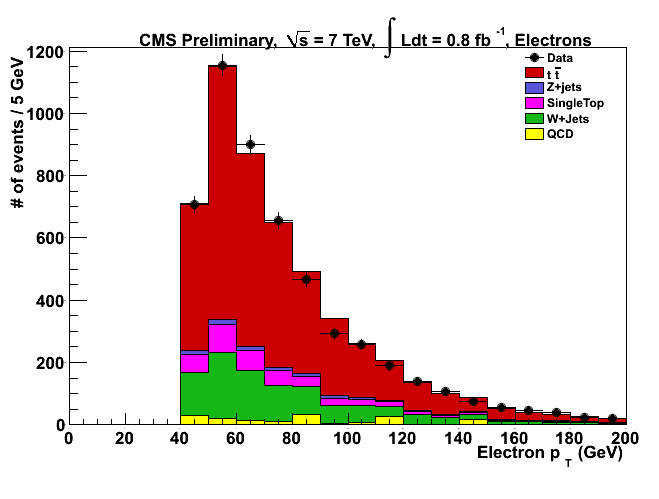
Well, data matches the theoretical expectation just fine. And so we can confirm that, indeed, most particles in the proton are gluons carrying a small fraction of the proton’s energy.
I could go on from here; if you doubt any of these plots, I can show you dozens more that serve as cross-checks. The point that I hope I have made to you is that particle physicists’ confidence in our understanding of the proton’s structure derives from measurements like these, and from many others, not only at the LHC but also at many past experiments, stretching back decades. We’re not just making this stuff up out of our heads. We got it from the source.


28 Responses
I personally find it easier to think of protons and neutrons as stable macrostates in the sense of statistical mechanics.
As in, they’re tiny blobs of material whose bulk properties- in this case electric charge, average quark content, etc.- are stable over time even though their actual compositions and exact (micro)state are constant fluctuating. Analogous to how the temperature of a piece of metal or the net charge in a capacitor can be a stable high-level state even when the constituent atoms and electrons’ states are constantly changing.
This made it more intuitive as to why the composition of a proton or neutron would change when you add in more energy, and why at super-high energies things like protons can ‘melt’ into a kind of quark-gluon liquid. It also makes sense of why ‘protons’ and ‘neutrons’ would behave differently when isolated in space vs. when they’re inside a nucleus. Because the collection of states we call ‘protons’ and ‘neutrons’ in both cases aren’t actually the exact same things; the underlying blobs of rapidly changing quarks & gluons behave differently when they’re isolated vs. mixed together in nuclei, or when they absorb a lot of external energy (e.g. at the LHC).
What you’ve really done here is make a statement about how quantum mechanics bears a resemblance to (but is not the same as) statistical mechanics.
As you say, it seems as though they are “they’re tiny blobs of material whose bulk properties- in this case electric charge, average quark content, etc.- are stable over time even though their actual compositions and exact (micro)state are constant fluctuating”. But in fact, quantum physics doesn’t quite say this. It says something far more subtle.
It says that “their actual compositions act as though they are constant fluctuating, in the sense that certain measurements can give variable answers if you do them multiple times. But in fact their microstate does not fluctuate at all. The point is that their compositions are not specified by their microstate; even though the microstate is definite, the structure and makeup of the state is not.”
This is similar to the statement that even when a particle’s position is precisely known, there is no knowledge of its velocity, and vice versa. Composition and energy cannot be simultaneously known, because states of definite composition do not have definite energy, and states of definite energy do not have definite composition.
A proton (or neutron) is a state of definite energy. Its composition is not precisely specified (though the fact that it always has three more quarks than anti-quarks IS specified), and so the only thing that can be predicted, for measurements of its composition, is the probabilities of certain outcomes. That sounds naively like statistical mechanics, but it is *not*; statistical mechanics would not correctly account for the correlations (i.e. entanglement among the proton’s constituents) within the proton state.
Since Proton and Neutron rest mass is known to five digits accuracy,
it seems like a “magic number” might be involved.
It is also amazing that there are exactly three “valence quarks” floating on top of that stew.
Thank you very much for writing these insightful articles. I have very little to contribute, but in the spirit of “comments welcome,” I will point out that the construction “(though the current understanding of the proton itself arose three to four decades ago, through numerous past experiments which would form a story of their own.)” is a bit awkward with the repeated “though.” Perhaps you can either drop the first “though” or replace the second “though” with “the.”
The second one is “through”, not “though”.
Oops — my bad then. .Looks like my parser was confused by the comma. :-X
Hello Matt,
after reading this wonderful article I came up with a question. Why don’t quarks and anti-quarks (that are antiparticles of quarks) inside a proton annihilate in the same way that electrons and positrons annihilate when they meet each other?
Actually they do. If you check one of the answers to the third article linked at the start of this one, he mentions that any particular quark will quickly be annihilated via collision with an antiquark. (And subsequent conversion to other particles like gluons.) This is why he notes that not only is the number of particle inside a proton is unknown, but it is also not constant.
I am not sure why however they can’t annihilate to photons making the proton evaporate, but I’m guessing it’s because doing so would require more energy. (The proton is a lowest energy state.)
There are two bits to the answer of why they don’t annihilate to photons:
1. quark-antiquark annihilation happens through the strong interaction and therefore you get gluons.
2. But sometimes (they have opposite charges) they annihilate through the electromagnetic interaction and you do get photons. But those photons are confined inside the proton too – why do you expect them not to be?
These photons don’t last very long and the photon-photon annihilation of quark-antiquark is very rare (scale of the difference of the strengths of the strong and electromagnetic forces) compared to gluon-gluon.
Incidentally, you don’t get anything else from a quark-antiquark collision inside a proton because of the energies – the total energy in the proton is just under a GeV, and all the other possible results (W, Z) of quark-antiquark collisions are higher energy than that.
You can get strange-antistrange quark pairs, but you need to go through a gluon-gluon pair to get there.
I expect photons not to be bound inside a proton because I cannot imagine what would bind them. They do not react to the strong force which is what binds quarks and gluons. They might be reabsorbed by charged particles, but what stops a photon being produced at the ‘surface’ of the proton or by chance not interacting with charged particles long enough to escape the proton? Certainly the ‘virtual photons’ produced by the proton are not confined which allows it to exert an electromagnetic force over a distance. I would be interested in any references that deal with the matter of photon confinement under these circumstances.
An electron could absorb a photon of specific wavelength which enable that electron to quantum jump energy level, maybe particles within the proton can do that too? Photon absorption somehow involved conversion of lateral kinetic energy of the photon into angular kinetic energy perhaps? Interesting to think about considering a photon is the least possible ball of ripples that an electric field could have while an electron (which absorbed that photon) is the least possible ball of ripples that an electron field could have. I surely like to know too how does it work 🙂
I mean linear not lateral, the direction of photon wave propagation is linear kinetic energy and it is converted into angular kinetic energy when absorbed by an electron… just a conjecture 🙂
Matt,
Excellent series. A couple of questions:
1) Can you explain how picoBarns/GeV (figure 3) translates into a count of events per bin? I understand how luminosity per bin could be proportional to the count, but not how inverse luminosity per GeV correlates to an absolute count.
2) Is there some way you can stop showing “particles” in the diagrams as if they were billiard balls? Billiard balls (and point-like particles) don’t behave like quanta – e.g., interact statistically, travel over multiple paths simultaneously, become entangled, preserve integral quantum numbers, etc. – and don’t behave like wave functions in general. As you have pointed out, thinking of sub-atomic “particles” as “chunks of matter” leads to all sorts of misconceptions, so traditional diagrams like these can be misleading.
Thanks again.
I agree, great article, thanks a lot.
Great article!
An obvious, perhaps naive, question: I have an egg. If I strike the egg with a hammer at a fixed velocity and in a prescribed manner then I will characteristically shatter the egg. If I repeat this, with some precision, with an identical or similar egg and hammer we will discover that the remaining parts will tend, with some regularity, to have the same features. With enough data I may even develop a mathematical characterization of these events that allows me to predict the outcome, even when I vary the velocity of the hammer.
The thing is, the features of the event are the product of the hammer and the uniformity of the world, not the humble egg. In other words, the resulting structure, the data, is the product of the hammer not the egg. If you are trying to deal with the epistemology involved then this would be a good question to answer.
But eggs are a very different thing from protons. They have a defined shell which is capable of shattering, for one, and a consistent structure (although not wholly consistent between different types of egg). Whereas, protons have a statistically descriptive contents list.
And the features of the hammer event would be very much to do with the humble egg. Your hammer may, as well as shell, cast out bits of albumen, yolk or even an unlucky embryo. Or all three. And the ratios in which it does this will enable you to make some assumptions about the contents of the egg.
The other problem you have is that “in a prescribed manner” and “repeat this, with some precision” are both very hard. Some eggs have an air gap at one end – therefore it would be possible that a random hit with a hammer (and, given the size of the ‘hammer’ and the ‘egg’ in the LHC – both being protons in this case – all hits are going to be random) that you might shatter a bit of the shell but have minimal impact on the rest of the egg.
Anyway – they’re not smashing protons in to protons to understand the structure of the proton. They’re doing it because the various collisions between proton components may lead to interesting outcomes.
This response doesn’t answer the epistemological question posed (and this is a post about epistemology). If I knew nothing about eggs a priori, I could certainly come up with a statistically descriptive contents list as a result of smashing eggs.
This is indeed an interesting question. There are a few things to keep in mind first though.
Firstly what we have in the case of the LHC is not a hammer and egg, but two eggs being hit together. (These are proton-proton collisions.) Immediately this changes your example since when I smash two eggs together in a certain manner (The easiest method i can think of would be dropping one egg onto another from a fixed height.) we’d expect what forms to be due entirely to the eggs themselves.
Secondly physicists have varied the ‘hammer’; all sorts of things have been fired at protons (And protons at all sorts of things.) and so far the results of such experiments support the quark model. The first evidence of quarks came from ‘deep inelastic scatter’ where leptons were fired at protons.
We also find, as mentioned in previous articles, that the theory has been used to make accurate (Quite accurate!) predictions that were then confirmed.
But the epistemological problem, being philosophical at heart is impossible to answer. We can never see the “ding an sich” (Thing per se) that is the proton, everything is filtered through our observations and the question quickly moves into the arena occupied by such problems as ‘What is knowledge’ and ‘Can we ever prove anything?’ And that way madness lies.
Fortunately we need not deal with the problem. Science merely says that this model of the proton is what agrees with observations. (Or makes the best predictions of reality.) It may be false, there may not be any thing as protons at all, the eggshell fragments may be totally due to the hammer, but until someone can provide observations that directly contradict the current theory, science says ‘If it isn’t broke, don’t fix it.’
And maybe, dare I say hopefully, somewhere deep in the LHC we may see something that shouldn’t be there, beckoning us onwards to new discoveries.
I was told that the proton-proton collisions at LHC obeys the E^2 – p^2c^2 = m^2c^4 energy formula, p^2c^2 is the momentum imparted to each proton by acceleration (your hammer) if there is no acceleration p^2c^2 = 0 and the whole energy equation E^2 – p^2c^2 = m^2c^4 will be reduced to the more familiar E = mc^2 (your egg).
The acceleration imparted 4 TeV energy for each proton that added up to 8 TeV per collision, enough to disintegrate the protons releasing its energy and components into extremely high pressure and temperature debris. I have no idea yet how CERN do the accounting of 600 million collisions per second that proved E^2 – p^2c^2 = m^2c^4 is obeyed.
A proton can indeed absorb a photon and enter a more energetic state. In fact this is a mechanism that slows high energy protons in cosmic rays, they ‘bump into’ the cosmic microwave background photons, absorb them and become excited, later decaying. http://en.wikipedia.org/wiki/Greisen%E2%80%93Zatsepin%E2%80%93Kuzmin_limit
But if you accelerate the egg won’t you pre-scramble the contents thereby affecting the outcome, data?
Thanks Matt, you have now totally converted me from the rather too simplistic Wikipedia version of the proton. I am really grateful for all your hard work in updating us on the current status of particle physics.