[This is a follow-up to Monday’s post, going more into depth.]
Among the known elementary particles are three cousins: the electron, the muon and the tau. The three are identical in all known experiments — they have all the same electromagnetic and weak nuclear interactions, and no strong nuclear interactions — except for effects that arise from the fact that they have different rest masses:
- electron rest mass: 0.000511 GeV/c2
- muon rest mass: 0.105658 GeV/c2
- tau rest mass: 1.777 GeV/c2
[These differences arise from their different interactions with the Higgs field; to learn more about this, see Chapter 22 of my book.]
[Note added for clarity: these particles do exhibit slightly different magnetic moments, dramatically different lifetimes, and a number of other differences — but all of those variations can be traced back to the difference in their masses.]
Question: How sure are we that these three cousins aren’t actually the same object, seen in three different quantum states?
Quantum States
This is a serious possibility, at first glance. After all, individual atoms have many states, in which they look roughly the same but have different energies — which means, because E=mc2, that they have different rest masses. In Fig. 1 are some of a hydrogen atom’s many possible states; the one of lowest energy is called the “ground state”, and ones with more energy are referred to as “excited states”.
In a number of ways, hydrogen atoms in these different states are almost the same; each has zero electric charge, and each contains an electron and a proton. They differ in what the electron is doing, as sketched in Figure 2.
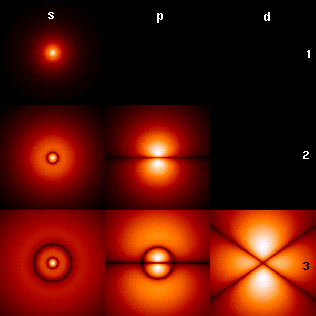
From the outside, though, they seem almost the same; the most obvious difference is that they have different amounts of energy. Might the electron, similarly, be the ground state of a complex object, with the muon and tau being that object’s most easily accessible excited states?
I’ve already mentioned one of the facts in favor of this hypothesis: the three particles’ identical interactions with all fields (except the Higgs field). Another hint that might support the hypothesis is this: when a muon or tau “decays” (i.e. when it transitions, via dissipation, to more stable particles), the outcome always includes an electron. For instance, the muon decays to an electron, a neutrino and an anti-neutrino. Tau decays are more complex, but in the end, an electron is always found among its decay products.
Nevertheless, the hypothesis is definitely wrong, as we can see by carefully comparing atoms, electrons, and protons. I’ll do this in two stages:
- Today I’ll describe what we learn from collisions of these particles.
- Soon I’ll describe what we learn from “spin” — angular momentum carried by a particle.
How to Excite an Atom
Let’s look at two typical ways to excite an atom; there are many others, but these two will do for today.
Shining Light
First, we could shine ultraviolet light (an invisible form of light at slightly higher frequency than visible light) on an atom. If so, we might observe processes such as those sketched in Figure 3: a photon of light strikes the atom in its ground state, and what emerges from the collision is the atom in one of its excited states (possibly plus one or more photons). The simplest possible process involves
- photon + atom in ground state → atom in excited state
The excited atom then reveals itself when, at a later time, short on human scales but often long on atomic scales, it decays back to the ground state,
- atom (excited state) → atom (ground state) + one or more photons
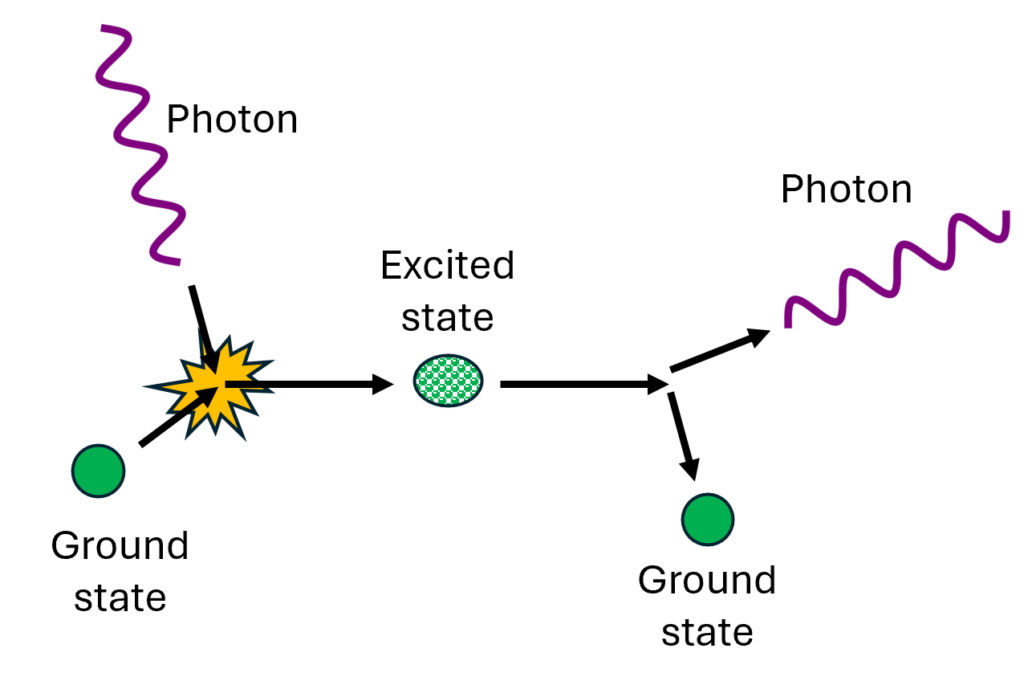
If there’s just one photon in the excited state’s decay, that photon has always the same frequency 
where h is Planck’s constant. [A nitpick: this formula applies when the excited atom is stationary, and has a small correction from the fact that the atom will be moving slowly after the decay.]
Atomic Collisions
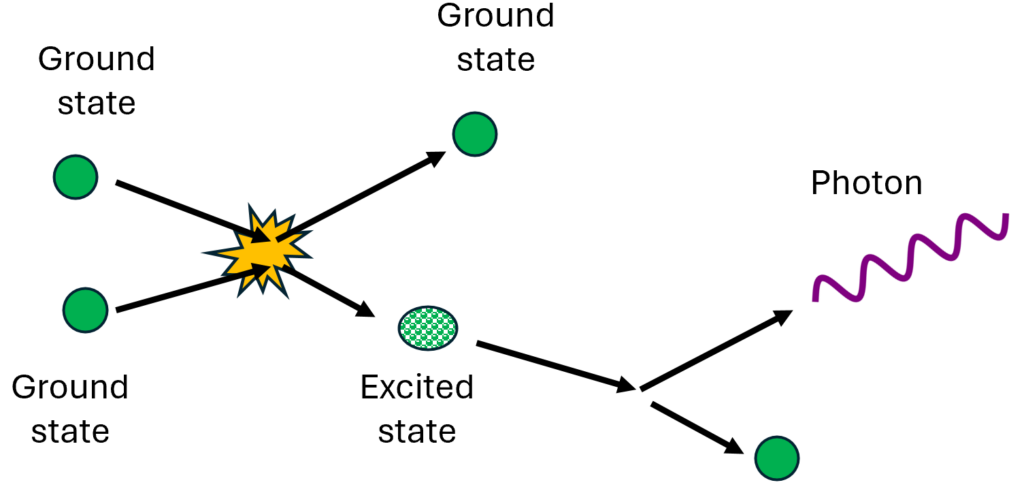
Second, we could slam two atoms in the ground state together. If the speed is high enough, one of the two atoms could come out in an excited state, as sketched in Figure 4.
atom (ground state) + atom (ground state) → atom (ground state) + atom (excited state)
Or both atoms could come out in excited states, though not necessarily the same ones. Again, we would learn which excited states were created by looking at the photons emitted when the atoms transition back down to the ground state.
Could We Excite an Electron?
Let’s imagine trying similar tricks just like this on the electron. We could shine high-energy light on the electron, or we could slam electrons together, seeing if we could turn an electron into a muon or a tau.
Shining Light
If a muon is an electron’s excited state, we could shine light waves — gamma-rays this time, as ultraviolet light would not be enough — at electrons, hoping to turn an electron into a muon. Using the notation
for electron (the minus-sign reflecting its negative electric charge)
for muon
for a photon (and
for a second photon)
we could try to look for the processes
,
possibly plus one or more photons, as shown in Figure 5.
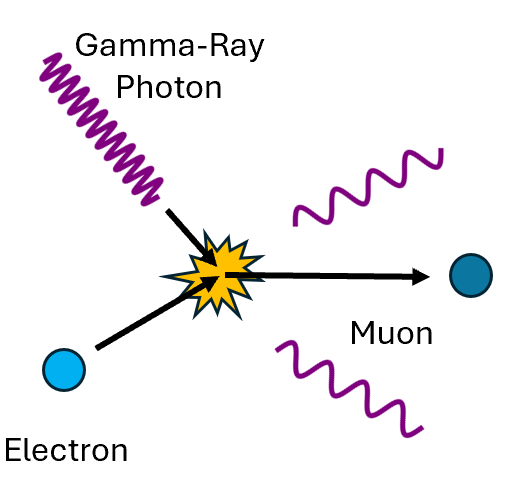
Direct searches for processes like this have been done, and none has ever been seen. Even more significantly, if they were possible, then the following related process (shown in Figure 6) would also be possible
This has been searched for with great effort. Experiments show that no more than one in 2 trillion muons decay this way. The analogous processes for tau’s have never been seen, either.
So what? Even though we can’t excite electrons this way, does that really prove that electrons can’t be excited in some other way?
Essentially, it does. The problem is that electrons are electrically charged, and so, if they are made from other, even more elementary objects, then one or more of these objects must also be electrically charged. By its very definition, “having non-zero electric charge” means “able to interact with photons.” It’s virtually impossible to imagine how an electrically charged interior would be unable to absorb photons. So this is an extremely strong mark against the idea.
But just to be sure, let’s try another approach.
Electron-Electron Collisions
We could also try aiming electrons at each other and seeing what happens when they collide. Just as atomic collisions cause atoms to be excited, we would expect that sufficiently powerful collisions would excite electrons to be muons and taus, and so we should observe processes similar to those in Fig. 4, such as
But again, none of these processes has ever been observed.
Electron-Positron Collisions
It’s interesting to compare this to what happens in collisions of electrons 
However, we never observe
or anything similar. Only a muon and an anti-muon, or a tau and an anti-tau, are ever created.
Meanwhile, another thing we observe
But clearly photons cannot be excited states of electrons, as photons have zero electric charge and smaller rest mass. So this process has nothing to do with creating an excited state.
Similarly, the processes that create 
Each of the two new particles is an antiparticle of the other: the muon 
We can consider other forms of scattering too, and in none of them do we ever see any of the processes that would be consistent with muons or taus being excited states of electrons. Moreover, all experiments on these particles agree with the math of the Standard Model of particle physics, which is based on the assumption that muons and taus are independent particles from electrons, and are not excited states of the latter.
We can conclude that this excited-electron hypothesis is completely dead. It has been for some decades.
What About Protons?
Protons, meanwhile, do have a size. Do the processes mentioned above work for them?
Yes. The first excited state of the proton 
is observed. (In fact this process has a major role to play in the features of cosmic rays, where it causes what is known as the GZK cutoff.) [More easily observed is 
Scattering processes also create the excited states:
So we see, in experiments, many processes that we would expect to see if the proton is a composite object made of more elementary objects, for which the usual proton is the ground state and the
From these excited states, the size of a proton can be roughly inferred, as explained here.
What About All Those Photons?
But what about the fact that even electron-electron collisions often generate photons? Might that be an indication that electrons have excited states?
In other words, even in processes as simple as two electrons that scatter and remain electrons, photons generally appear
- etc.
Why aren’t these indications that electrons are composite? Because, as with the electron-positron annihilation processes discussed earlier, processes like these are expected even for elementary electrons; and predictions for those processes, based on the assumption that electrons are elementary, agree perfectly with data.
So does this mean that electrons definitely are elementary, point-like objects? Not definitely, no. It simply means that if electrons are the ground states of something complex (such as a string, as would potentially be true in string theory), the excited states of that object have far too much rest mass for us to produce them using today’s technology. Someday, collisions may produce them. But for now, all we can say is that in every experiment we can currently perform, electrons appear elementary. So do muons and taus; so do the neutrinos; and so do all six quarks of the Standard Model.
22 Responses
You guys dont understand what mass and photons are. I do, i am currently working out this oscillation issue with neutrinos and electrons. Start first with understanding wjat gravity is. [link removed by host]
Thank you, Einstein Junior, for bringing your… innovative… work to our attention. I am sure that, had Einstein been alive today, he too would have spent his time reading other people’s blog posts, and advertising his ideas on their websites.
Why can’t they be excited states of weak charge? They would get excited by absorbing weak charges not photons. Isn’t that who they acquire mass from the higgs field in the fiest place?
There’s no sign of anything like that either. For instance, neutrinos scattering off electrons (which involves weak charge) don’t turn them into muons. Certain anti-neutrinos have a probability of doing so, but again, that’s consistent with annihilation processes similar to the electron-positron annihilation I discussed.
Why aren’t forbidden transitions (due to internal symmetries) enough to make the argument float?
Hi Matt, your question of three generations from a single object is intriguing.
My colleagues and I recently found a way to use Majorana-Weyl spinors from extra dimensions to encode on-shell states for three generations of Dirac spinors from 16 off-shell degrees of freedom by using Cl(4,4) instead of 3*8=24. This could also be formulated with the split octonions or simply seen as the 8s + 8c representations of Spin(4,4). By considering three overlapping conformal charts, this fulfills a vision from Bertram Kostant with three conformal charts as well as Wilczek et al.’s study of SO(3) symmetry.
The 128 spinor of Spin(4,12) in E8(-24) has onshell dof for three generations of the standard model in this manner as well. Does this count as three generations stemming from one object?
https://arxiv.org/abs/2002.02391
I am curious; I have an intuition for the excited states of an atom, electrons moving to higher energy levels. But how is a proton excited? From descriptions of it, it is a chaotic sea of quarks and gluons. I’d assume an excited state just added a quark-antiquark pair to the sea but that doesn’t seem to be the case. What is the difference between a proton and a delta?
A proton is a very complex object, and the excitation is likewise very complicated. We can calculate the Delta using computers (see Figure 2 of https://link.springer.com/article/10.1007/s00601-019-1488-x) but we cannot characterize it with simple math. We can pretend to, using the “non-relativistic quark model”, but that’s not at all an accurate description of what is a highly relativistic system. In that pretend model, one would say that one of the quarks’ spins has been flipped; the proton has spin 1/2 and the Delta has spin 3/2. But this is way too simple. And it would tell you nothing about the p(1440), the next excited state, which also has spin 1/2. Perhaps that is something like a sloshing mode of a liquid. But in the end, complex systems do not always lend themselves to simple pictures.
Goodness. Looking over this it seems like one might make an entire career rotted on the subject alone. I can barely grasp what they’re getting at,so I guess the answer is that there is indeed no simple intuition for how a proton ‘works’. Sad, but sobering.
It’s true that people have made much of their careers trying to understand protons and other particles made from quarks, antiquarks and gluons. These objects are not simple at all. Computers show that we have the right equations for the quarks and the gluons, because they can reproduce the proton’s features, but that doesn’t mean that there’s a simple summary of how a proton works.
If a tau or muon can decay into an electron plus other stuff, the reverse should be possible. (Since this involves neutrinos, it would be very rare indeed to observe this in an experiment.)
I’m curious about electron-muon collisions, where the particles have opposite charges — I assume from this post that nothing interesting happens until the muon decays, and then the electron-positron pair will annihilate.
An electron-muon collision does nothing; the two particles scatter off each other, and that’s it.
The same is true of an electron-antimuon collision does nothing; the two particles scatter off each other, and that’s it.
By the time the anti-muon decays, the electron in the collision is far away; meanwhile the positron from the anti-muon’s decay goes rushing off in some other direction at nearly the speed of light, so it won’t annihilate with the electron that participated in the collision. It will have to find another electron.
A muon decays to an electron, not a positron.
A neutrino beam colliding with an electron can turn into a muon ,while the electron becomes a different neutrino. (We know it’s the neutrino that turns into the muon, not the electron, because switching from neutrinos to antineutrinos causes the effect to shut off.)
The muon’s decay products include neutrinos, which means that the muon spin is more complicated than the electron spin. Hence the muon is not an excited electron. Speaking of which, you said this:
“Instead, the electron and positron are “annihilating” — they are transformed into a disturbance in the electromagnetic field (often called a “virtual photon” — but it is not a particle) — and this disturbance spontaneously transforms into two new particles that are “created” in their stead.”
If Erwin Schrödinger was here, I’m pretty sure he’s say it’s just a change from two closed paths to two open paths, because the opposite chiralities cancel. See this: https://5p277b.n3cdn1.secureserver.net/wp-content/uploads/SchrodingerClosedPath.jpg.
Data shows that the muon’s spin is exactly the same as the electron’s spin. The neutrinos are not inside the muon; they are created, from scratch, when the muon decays.
I didn’t say they were inside, I was referring to conservation of spin angular momentum. See “What is Spin?” by Hans Ohanian (https://physics.mcmaster.ca/phys3mm3/notes/whatisspin.pdf). Can you provide a link to the the data that shows that the muon’s spin is exactly the same as the electron’s spin?
You may not have understood Ohanian’s math, because you’ve drawn the wrong conclusion.
As for experiment: The electron and muon both have spin 1/2, and their similarity is evident in the fact that both have a “g-factor” extremely close to 2.
See “Measured g-factors” in https://en.wikipedia.org/wiki/G-factor_(physics) ; the electron and muon g-factors are actually the same to 5 decimal places, and are completely different from those of the proton and neutron. The differences between their g-factors are calculable — they are due to the two particles having different masses. These g-factors are two of the best-measured, best-calculated, and best-agreeing quantities in all of physics… despite a very small discrepancy in the muon’s case, which might or might not be a sign of something beyond the Standard Model.
https://en.wikipedia.org/wiki/Muon_g-2
I thought people gave up on trying to model electrons (and hence spin) classically when their point-like behavior was discovered? The picture I get from some particle blogs is that spin is seen more as a topological feature than angular momentum of a wavicle described by a wavefunction. C.f. https://en.wikipedia.org/wiki/Spin-1/2.
They didn’t. As far as I can tell, in the 1920s the Copenhagen School adopted Yakov Frenkel’s 1926 paper “On the electrodynamics of rotating electrons”, which said the electron will thus be treated simply as a point. This was a deliberate rejection of work by realists such as de Broglie, Schrodinger, Darwin, and Born & Infeld, who thought of the electron as a wave in a closed path. See Born and Infeld’s 1935 paper “On the quantization of the new field theory II”. However the Copenhagen view prevailed, especially since the realists were scattered by the Nazis. Then renormalisation set it in stone. Nowadays closed-path electron papers such as Williamson and van der Mark’s “Is the electron a photon with toroidal topology?” get rejected by Nature etc. Hence you don’t get to hear about it. Even though the Einstein-de Haas effect “demonstrates that spin angular momentum is indeed of the same nature as the angular momentum of rotating bodies as conceived in classical mechanics”.
Nice! I am curious: Do you have any thoughts or comments on the Koide formula [1]? From an information analysis perspective, such unexpected but precise relations typically turn out to be connected to the final resolution of the problem, with the equal pairing of nucleotide types in the story of the DNA double helix being a notable example.
Piotr Żenczykowski has a paper [2] that gives a more recent example of mulling over Koide’s finding.
———-
[1] Y. Koide, “New view of quark and lepton mass hierarchy,” Physical Review D 28 (1), 252 (1983). https://journals.aps.org/prd/abstract/10.1103/PhysRevD.28.252
[2] P. Żenczykowski, “Remark on Koide’s Z3-symmetric parametrization of quark masses,” Physical Review D 86 (11), 117303 (2012). https://arxiv.org/abs/1210.4125
I don’t have a strong opinion or comment. There are many ideas and speculations about these problems, and either most or all of them will turn out to be wrong.
If I may: I have an opinion. The base pairing in DNA has nothing to do with sequence (genetic) or sequence control (epigenetic) information. It stabilizes the DNA and enable it to compactify in cells – the human cell DNA totals 2 m – but sequence information can be carried by DNA quadruplexes, double strand DNA, single strand DNA, single strand RNA (with or without transcription depending on genes and species – say tRNA or RNA viruses) and proteins (after translation).
The open reading frame of a gene (to take a common form of sequence information) will be dominated by the triplet code along either direction of a strand, and it is here that the base pairing works its magic. The wobble base pair floppiness enables proper (and synonymous triplet codon) translation.
It is likely that the base pairing evolved in the RNA world before the triplet code and “storage and replication” sturdy DNA did. But if it did, it was likely to enable RNA replication, not primarily for RNA world sequence information.
As a context to the interest in sequence information – which ironically is of little interests for bioinformaticians or even biologists – Richard Dawkins discussed the population genetics information content of genomes in an essay, where he implicitly dismissed the Kolmogorov entropy measure of information to note that evolution is a process that channels Shannon information into the genome.
“But it still remains true that natural selection is a narrowing down from an initially wider field of possibilities, including mostly unsuccessful ones, to a narrower field of successful ones. This is analogous to the definition of information with which we began: information is what enables the narrowing down from prior uncertainty (the initial range of possibilities) to later certainty (the “successful” choice among the prior probabilities). According to this analogy, natural selection is by definition a process whereby information is fed into the gene pool of the next generation.
If natural selection feeds information into gene pools, what is the information about? It is about how to survive. Strictly it is about how to survive and reproduce, in the conditions that prevailed when previous generations were alive.”
https://web.archive.org/web/20000309190434/http://www.skeptics.com.au/journal/dawkins1.htm
IIRC a paper estimated that the entropy export needed for the “cooling to order” of the genome is 10^-11 parts of the entropy export needed for cell growth and replication. So it is an insignificant process as far as life is concerned.
The essay describes is a simplification since you can think of a population total genome pool as having mechanisms that increase the allele variety – mutation, recombination, migration – and mechanisms that decrease the allele variety – drift, selection, inbreeding. Notably base pairing is none of that.