A post for general readers:
Within the Standard Model, the quarks (and anti-quarks) are my favorite particles, because they are so interesting and so diverse. Physicists often say, in their whimsical jargon, that quarks come in various “flavors” and “colors”. But don’t take these words seriously! They’re just labels; neither has anything to do with taste or vision. We might just as well have said the quarks come in “gerflacks” and “sharjees”; or better, we might have said “types” and “versions”.
Today I’ll show you how one can easily see that each of the six flavors of quark comes in three colors (i.e., each gerflack/type of quark comes in three sharjees/versions.) All we’ll need to do is examine a simple property of the W boson, one of the other particles in the Standard Model.
[Another way to say this is that the Standard Model is often described as having a kind of symmetry named “SU(3)xSU(2)xU(1)”; today we’ll put the “3” in SU(3). ]
Gerflacks and Sharjees of Quarks
We know there are six types/gerflacks/flavors of quarks because each type of quark has its own unique mass and lifetime, a fact that’s relatively easy to confirm experimentally. Quarks 1 and 2 are called down and up, quarks 3 and 4 are called strange and charm, and quarks 5 and 6 are called bottom and top; again, the whimsical names don’t have any meaning, and we often just label them d, u, s, c, b, t.
But to understand why each type of quark comes in three versions/sharjees/colors is more subtle, because two quarks of the same “flavor” which differ only by their “color” appear the same in experiments (despite our intuition for what the word “color” usually means.)
What, in fact, is a “color”? Each color/sharjee/version is a kind of strong nuclear charge, analogous to electric charge, which we encounter in daily life through static electricity and other phenomena. Electric charge determines which objects attract and repel each other via electrical forces. Electrons have electric charge, and so do quarks; that’s why electrical forces affect them. But quarks, unlike electrons, have strong nuclear charge too, and those charges determine how quarks attract or repel one another via the the strong nuclear force.
And here’s the interesting point: whereas there is only one version of electric charge (electrons and protons and atomic nuclei have different amounts of it, but it is different amounts of the same thing), there are three different versions/sharjees/colors of strong nuclear charge. They are often called “red”, “green” and “blue”, or “redness”, “greeness” and “blueness”. (Remember, these are just names for sharjees — for versions of strong nuclear charge. In no sense do they represent actual colors that your eyes would see, any more than the six types/flavors of quarks would taste differently.)
“Color”? Which One?
The particles of the Standard Model which have strong nuclear charge are the quarks, their anti-particles (anti-quarks), and the gluons (associated to the strong nuclear force as photons are associated with electric and magnetic forces.) What strong nuclear charges do they have?
Before answering that, let me remind you about electric charges. Every type of particle in nature has a fixed electric charge. Ordinarily, atoms have electric charge 0 (i.e. they are electrically neutral), but within an atom,
- electrons have electric charge -1,
- protons have electric charge +1,
- neutrons, as their name suggests, are neutral — electric charge 0,
Also, photons, the particles of light that are associated with the electric field and electric forces, are electrically neutral too.
[In first-year university physics, we usually teach that electrons have electric charge “-e“, but professionals, for reasons I won’t address now, prefer to say the charge is “-1” and associate the “e” part of the charge to the electromagnetic force itself.]
In an analogous way,
- A quark has one color: a +1 for one (and only one) of the three sharjees
- An anti-quark has one anti-color: a -1 for one (and only one) of the three sharjees
- A gluon has one color and one anti-color: +1 for one sharjee, and -1 for one sharjee
Notice that while photons have no electric charge, gluons have strong nuclear charge. This has enormous importance. It makes it impossible to observe a particle’s color, because as the particle interacts via the strong nuclear force, its color changes. Metaphorically, a quark is bit like a light bulb that is always on but that flickers rapidly and unpredictably between red, green and blue. Contrast this with the electric charge of electrons, which never changes.
Worse, a quark is always confined within a proton or a neutron or some other hadron, combinations of particles whose total color is zero. We can never isolate a quark and try to examine it carefully; they are stuck inside colorless objects. By contrast, although atoms have zero electric charge, we can separate an electron from an atom and study it in isolation.
Since we never really observe a particle with a definite “color”, how can we possibly count how many colors there are? Fortunately, processes involving other forces, such as the weak nuclear force, don’t care what color a quark has, and so they can count for us.
The Decays of the W Boson
The particles associated with the weak nuclear force are the Z boson and the two W bosons (a W-plus with positive electric charge, and its anti-particle, a W-minus with negative electric charge.) The W bosons have a large mass, over 80 times the mass of a proton. They also have a very short lifetime, decaying in about a trillionth of a trillionth of a second.
Let’s put their decays to good use. Below in Figure 1 are shown the experimentally-observed probabilities for the various decays of the W-minus boson (W–) to an electron or one of its cousins (the muon and the tau) and a corresponding anti-neutrino, or to quark/antiquark pairs. [For W+ bosons, the probabilities are the same, except with each particle replaced by its anti-particle.] A striking pattern appears; of the W’s decays, 11% or about 1/9 are to an electron, and similarly for the muon and the tau, while 33% or about 1/3 — which is 3/9 — go to each quark/anti-quark pair — except the bottom/top pair, where we get zero.
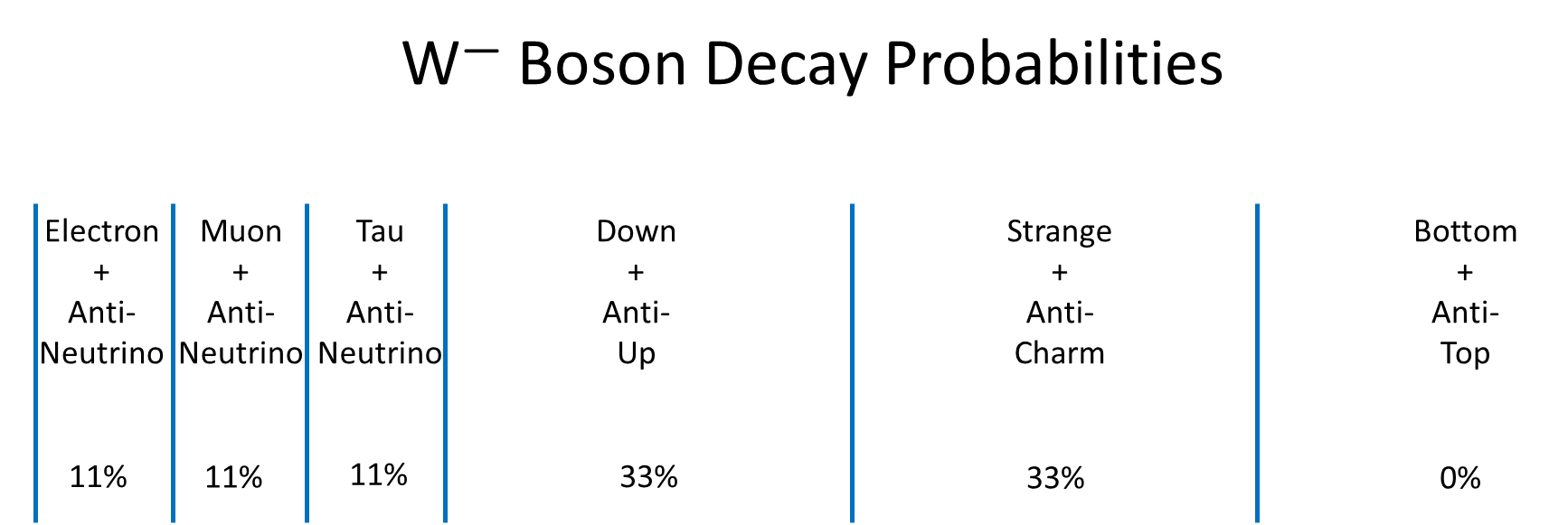
The zero for the last column is easy to understand in terms of a basic rule that applies to all decays: the rule of decreasing rest mass.
- The rest mass of a decaying particle must exceed the total rest mass of the particles emerging from the decay.
Since a top quark has greater mass than does a W boson, decays of a W to a top quark or anti-quark (plus anything else) are forbidden by this rule.
Once we account for this, it’s natural to interpret the experimental results as in Figure 2, where I’ve depicted the three versions/sharjees/colors of the quarks with visual colors. Because the W is color-less, if it decays to a red quark, the accompanying anti-quark must be anti-red (at least at the moment of their creation.) So if there are three colors of down quarks, then there are three ways that a W can decay to a down quark and an up anti-quark, one for each color. That means that in addition to the 3 pairs of electron-like particles and anti-neutrinos, there are really 6 quark/anti-quark pairs corresponding to two flavor possibilities with three colors each. That gives a total of nine pairs, each of which has probability 1/9 = 11%.

Notice that this only works with three colors/sharjees/versions. If we have N quark colors, then N<3 gives too large a probability for decays to electron + anti-neutrino, while N>3 gives one that’s too small. More precisely,
- the probability for W– to decay to electron plus anti-neutrino or any other similar pair = 1 / (3+2N)
- the probability for W- to decay to all colors of a particular quark/anti-quark pair = N / (3+2N)
With the precise measurements available today, it’s clear that only N=3 will do!
A few final comments
This argument has a loophole, for I quietly assumed that the weak nuclear force has the same strength for quarks and electrons. That’s not a foregone conclusion! One could try instead to explain Figure 1 by suggesting that there’s only one color, but the weak nuclear force is stronger for quarks, making W decays to quarks more likely. But such a hypothesis would be inconsistent with other experiments. For instance, the tau (the heaviest cousin of the electron) and the charm quark have very similar masses, and decay in similar ways. Because of this,
- if my original assumption is correct, their lifetimes should be similar, while
- if instead the weak nuclear force is stronger for quarks, then the charm quark should decay faster than the tau and so its lifetime should be markedly shorter.
Experimentally the tau and charm have approximately the same lifetimes, so the assumption is supported by the data. By itself, this piece of evidence doesn’t close the case, but it’s just one of many; decays of neutrons, scattering of neutrinos from atoms, etc. all support the idea that the weak nuclear force is “universal” — has the same strength for all quarks, neutrinos and electron-like particles. Also persuasive, although it requires expertise beyond today’s post, is that there’s no simple math that can make the weak nuclear force stronger for quarks; it leads to inconsistent equations.
The W boson is not the only particle that counts the number of colors. Decays of other particles, such as the Z boson, the Higgs boson, the tau, and the charm and bottom quarks, do so too. But each of these is a bit more complicated than the W, with new subtleties. I’ll return to some of these in later posts.
Finally, decays aren’t the only processes that can tell us the number of colors. In fact, the rate at which collisions of electrons and positrons produce hadrons can tell us both the number of colors per quark type and the electric charges of the different types of quarks. My next post in this series will explain how that works.



20 Responses
Nice
Posting to say thank you! Appreciate the work you put into these. Please keep them coming.
One of the oddest historical features in how symmetry theory was applied to fermions in the Standard Model is its lack of recognition of the electric-color separation asymmetry: Fermions with electric-charge-only exist, but fermions with color-charge-only do not. Color charge instead exists only in repeated finite-ratio combinations with an electric charge. The latter argues that the color charge definition used in the Standard Model does not reflect the data.
Sheldon Glashow first documented this data-driven simplification with his 1980 fermion charge cube mnemonic, though from old papers I suspect it originated with Salam. In Glashow’s all-positive cube example, the combined color and electric charges of the three anti-down quarks define the unit vectors of three orthogonal axes. All positive fermions and anti-fermions, including the neutrino as the zero charge case, become sums of 0, 1, 2, or 3 of these “new” color-electric charge units. Positioned vertically, the body diagonal becomes Maxwell’s charge displacement, with the positron at the top. Another cube with the anti-neutrino at the top does the same for all negatively charged fermions and anti-fermions.
So why bring up all of this ancient (42 years, wow!) history here?
Because the same mnemonic works amazingly well for remembering which weak interactions are allowed. Pair both Glashow cubes into a hypercube and the 16 (per family) pro- and anti- fermions pair into 8 “bridge vectors” with T3 isospin symmetry. Flip any vector up, and you get its positive (or zero) T3 isospin version. Flip it down, and you get its negative (or zero) form. Flip (interact) two_opposite_ vectors, and you get, well, a W exchange.
Especially in color, the resulting figures are quite pretty and mnemonic and also emphasize the importance of color conservation in weak interactions. You even get trivially obvious names for the eight: R, G, B, N (the last for Negative) for matter, and C, M, Y, P for the antimatter vectors:
https://sarxiv.org/apa.2022-08-05.0945.pdf
Ages ago, Heisenberg and Wigner noticed this same symmetry, muddled up by the presence of fermion triplets in protons and neutrons. Yet even now, if you do nothing more than label one state of a proton RuGdBd, then its exact T3 weak isospin symmetry becomes RdGuBu, the neutron. That’s rather cool since it means Heisenberg was, at least abstractly, _exactly_ correct as long as you flip all three nucleons at once and track color conservation.
They called their composite T3 vector a “nucleon.” I’ve been calling the eight fermion-level equivalents of nucleons “isovectors,” but perhaps there’s a better or even existing term for these T3 isospin pairs.
Dr.. Strassler, If I maybe so bold as to push back slightly on a comment you made above regarding the Standard Model not being “attractive.” Me personally, I think the Standard Model is pretty elegant. Particularly in the fact that there is still room for generational growth. Is it possible that any “ugliness” is introduced by us trying to force the standard model to comply with our understanding rather than us really trying to understand what the standard model is telling us? And, thank you so much for the comment about GUTs being speculative. So many comments I have run across look at GUTs and ToEs as dogma and in my mind this is where we lose understanding what the universe is trying to show/tell us.
Generational growth is ruled out. Any additional generations like the ones we observe are excluded by measurements of the Higgs boson’s production rate, and were already constrained by the Z boson’s decay properties.
Hi Professor, I just recently came across this blog and I love it. It super informative and accesible to the curious layman like me.
I have a question in principle unrelated to the post, you explain many times the concept of fields permeating the universe and how a particle is the smallest amplitude ripple (wave) in the corresponding field. What would then be a ripple of say the photon or electron field with greater amplitude than the smallest? The same particle but with more energy? Another particle? And is the amplitude capped somehow at some point on the bigger side? Thanks a lot for your time in advance! 🙏 😊
Only specific amplitudes are allowed — that’s quantum physics.
Square-root-of-2 times a single a photon’s amplitude is what you get when you put two photons of the same frequency on top of one another; together these have twice the energy of 1 photon. Square-root-of-n gives n photons, with n times the energy of 1 photon. For large enough n the amplitude seems continuously variable and there is no maximum amplitude.
For an electron you cannot increase the amplitude (for a fixed frequency); this is the Pauli Exclusion principle in action.
By contrast, to change the particle’s energy, you change its frequency, not its amplitude.
I need to warn you, though, that there is a small cheat, made for pedagogical reasons, in what I’m saying, which requires a footnote, because I haven’t told you the precise *shape* of the ripple — it is a wave, yes, but how many crests and troughs does it have? Clearly that number is not infinite. The amplitude (but not the energy) of a photon depends on this number. So the precise statement is: for ripples with a fixed number of crests and troughs and a definite frequency and wavelength, there is a minimum allowed amplitude — and that is the amplitude of a single photon, whose energy depends on the frequency but not on the number of crests and troughs. [This goes under the terminology of “wave packet”, and now things are getting a bit more complicated than I can really address here.]
Depending on your level of interest and comfort with math, you may find this useful: https://profmattstrassler.com/articles-and-posts/particle-physics-basics/fields-and-their-particles-with-math/
/Notice that while photons have no electric charge, gluons have strong nuclear charge. This has enormous importance./
This is “two photons effect (👀 or C^2)”? ..
where the “square” in Localized relativity appears “circle”, in length contraction has less energy (lowest energy) a SU(3) symmetry breaking or the RestMass.?
The W bosons have a large mass, over 80 times the mass of a proton. They also have a very short lifetime, decaying in about a trillionth of a trillionth of a second (decreasing rest mass or ‘Linear one photon’?).
Which group would accommodate all interactions and all particles?
In a sense, none. If you ignore the Higgs boson, SU(5) is great; that’s the original theory of grand unification by Georgi and Glashow from 1974. But the Higgs boson doesn’t fit, and you have to add a Higgs that carries color (a color-triplet Higgs) in order to make the SU(5) structure work. Unfortunately, that Higgs violates baryon number, and now you have to start playing games to assure that the proton doesn’t instantly decay. Moreover, you get predictions that relate quark and lepton masses that don’t work, so you need additional structure; more Higgs bosons, typically. There is no grand unified theory that works “right-out-of-the-box”; you always have to massage it in ways that aren’t particularly aesthetically attractive. Then again, the Standard Model isn’t attractive either.
Is there a higher symmetry group (broken) which can contain both quarks and leptons?
That’s what grand unification is for. But grand unification’s still just speculation at this point.
I’m loving this series of articles. Thank you very much for them.
One question: is it just coincidence that there are 6 quarks, arranged as three generations of pairs, and six leptons, also arranged as three generations of pairs?
There are various ways to answer that question, and the answers are something between “no” and “probably not.”
There’s no theorem that it had to be this way, nor is there a physical principle which explains either the pairing within the quarks and leptons, the “generational” structure, or the fact that there are 3 generations.
One thing we can say is that each generation, combined with the known forces, gives a consistent mathematical structure; if you were then to remove either the quarks or the leptons from that generation, or reassign how a subset of the generation’s particles interacts with the forces, you would generally *not* get a consistent structure. But still, if you work at it you can find many consistent combinations of particles that could make up a generation — so the one we observe isn’t unique.
As for the pairing, there’s a link between how the pairing works and how the weak nuclear force works, as we’ll see in a later post. It has to do with the “2” in SU(2). But that, again, isn’t unique. Even SU(2) can have particles that come in sets of 3 or 4 or more, so pairs, while simplest, aren’t required. And even the observed pairing wouldn’t appear as it does were it not for the details of the Higgs field, which “marries” paired particles with some un-paired ones to make the particles we’re used to.
Nice post. But historically, wasn’t, apparent violation of Pauli principle by bound quarks the reason to introduce color. I was at Maryland in 60s and I thought Greenberg introduced color, although he probably does not get full credit for it. W decays were not known for quite a few years after that.
Historically there’s much more to the story; but if we taught Maxwell’s equations according to how they were understood historically, no one would understand them.
I’m a little confused about the analogy between electric charge and color charge. (I know the underlying math reasonably well.)
I tried to give a friend an explanation similar to this post recently, then realized I didn’t believe it…
The electric charge determines the representation of U(1) that acts on a particle: for charge +Q, the group element exp(i theta) acts via exp(i Q theta).
The thing that determines the representation of SU(3) that acts on a particle isn’t color charge in the sense of a (r,g,b) vector, right? Instead, shouldn’t the “SU(3) charge” in that sense be something that’s always the same for quarks (and corresponds to the 3 irrep) and always the same for gluons (corresponding to the 8 irrep)?
And then the (r,g,b) vector is analogous to the phase of an electron’s state, it’s the thing the group acts on.
Except… Electric charge is conserved. “Redness” is conserved. So maybe they are analogous in some other way?
(For spin: spin “s” determines the rep, like electric charge or “quarks are in rep 3”, the (2s+1)-dimensional vector is acted on, like electron phase or quark (r,g,b), and the conserved quantity is s_z, like electric charge (again) or redness. So maybe U(1) is a special case?)
Help!
Let’s save your question for the next post. I don’t want to answer advanced questions in posts for the general reader.
Dr. Strassler, outstanding paper. The two figures were incredibly useful in providing a visual of what is happening. Thank You. I know that your paper is about establishing 3 quark colors and the figures are great, but looking at the figures highlighted a couple of questions for me. First your figures reflect what happens with the W-Minus boson decay. What about the W+ boson decay and how it would effect the “odds” of what would occur. Or, would the W+ and W- decay be considered as independent decay processes. The W+ decay would also result in anti-matter charged particles so does this also play into the decay process? Second, looking at the decay process in the first figure there is no rest mass consistency in the decay particles. What happen to the “extra” mass/energy? Example, W-goes to a down and anti-up quark. Rest mass of W- is listed as 80GeV/c^2 while the down and anti-up have a estimated, listed combined rest mass of 6.9 MeV/c^2. Thank you again Dr. Strassler.
W+ decay is the same as W- decay except with all particles exchanged with their antiparticles. For example, W+ –> positron + neutrino with probability 11%, and –> up quark + down anti-quark with probability 33%.
In a decay, the mass-energy of the initial particle is converted to mass-energy **and** motion-energy of the particles in the decay. So the energy goes into the down-quark’s and up-antiquarks’ motion.